Question and Answers Forum

AllQuestion and Answers: Page 1322

Question Number 80159 Answers: 1 Comments: 4

IF THE SUM OF p TERMS OF AN A.P. IS EQUAL TO SUM OF ITS q TERMS. PROVE THAT THE SUM OF (p+q) TERMS OF IT IS EQUAL TO 0(ZERO).

$$\boldsymbol{{IF}}\:\:\boldsymbol{{THE}}\:\:\:\boldsymbol{{SUM}}\:\:\:\boldsymbol{{OF}}\:\:\:\boldsymbol{{p}}\:\:\boldsymbol{{TERMS}} \\ $$$$\boldsymbol{{OF}}\:\:\boldsymbol{{AN}}\:\:\:\:\boldsymbol{{A}}.\boldsymbol{{P}}.\:\:\:\boldsymbol{{IS}}\:\:\:\boldsymbol{{EQUAL}}\:\:\boldsymbol{{TO}} \\ $$$$\boldsymbol{{SUM}}\:\:\boldsymbol{{OF}}\:\:\:\boldsymbol{{ITS}}\:\:\:\boldsymbol{{q}}\:\:\:\boldsymbol{{TERMS}}.\:\: \\ $$$$\boldsymbol{{PROVE}}\:\:\boldsymbol{{THAT}}\:\:\boldsymbol{{THE}}\:\:\boldsymbol{{SUM}}\:\:\boldsymbol{{OF}} \\ $$$$\left(\boldsymbol{{p}}+\boldsymbol{{q}}\right)\:\:\boldsymbol{{TERMS}}\:\:\boldsymbol{{OF}}\:\:\:\boldsymbol{{IT}}\:\:\:\boldsymbol{{IS}}\:\:\: \\ $$$$\boldsymbol{{EQUAL}}\:\:\boldsymbol{{TO}}\:\:\mathrm{0}\left(\boldsymbol{{ZERO}}\right). \\ $$

Question Number 80146 Answers: 1 Comments: 0

Two system of rectangular axes have the same origin. If a plane cuts them at distance a, b, c and p, q, r respectively, then prove with the help of an appropriate diagram that : (1/a^2 ) + (1/b^2 ) + (1/c^2 ) = (1/p^2 ) + (1/q^2 ) + (1/r^2 )

$${Two}\:{system}\:{of}\:{rectangular}\:{axes}\:{have} \\ $$$${the}\:{same}\:{origin}.\:{If}\:{a}\:{plane}\:{cuts}\:{them} \\ $$$${at}\:{distance}\:{a},\:{b},\:{c}\:{and}\:{p},\:{q},\:{r} \\ $$$${respectively},\:{then}\:{prove}\:{with}\:{the}\:{help} \\ $$$${of}\:{an}\:{appropriate}\:{diagram}\:{that}\:: \\ $$$$\frac{\mathrm{1}}{{a}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{{b}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{{c}^{\mathrm{2}} }\:=\:\frac{\mathrm{1}}{{p}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{{q}^{\mathrm{2}} }\:+\:\frac{\mathrm{1}}{{r}^{\mathrm{2}} } \\ $$

Question Number 80139 Answers: 1 Comments: 6

Question Number 80142 Answers: 2 Comments: 0

a. Σ_(k=1) ^∞ ((k^3 /2^k ))=? b. Σ_(k=1) ^∞ (((k^3 +k^2 +k+1)/7^k ))=?

$$\mathrm{a}.\:\:\:\underset{\boldsymbol{\mathrm{k}}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\left(\frac{\boldsymbol{\mathrm{k}}^{\mathrm{3}} }{\mathrm{2}^{\boldsymbol{\mathrm{k}}} }\right)=? \\ $$$$\boldsymbol{\mathrm{b}}.\:\:\:\:\underset{\boldsymbol{\mathrm{k}}=\mathrm{1}} {\overset{\infty} {\sum}}\:\:\left(\frac{\boldsymbol{\mathrm{k}}^{\mathrm{3}} +\boldsymbol{\mathrm{k}}^{\mathrm{2}} +\boldsymbol{\mathrm{k}}+\mathrm{1}}{\mathrm{7}^{\boldsymbol{\mathrm{k}}} }\right)=? \\ $$

Question Number 80131 Answers: 0 Comments: 2

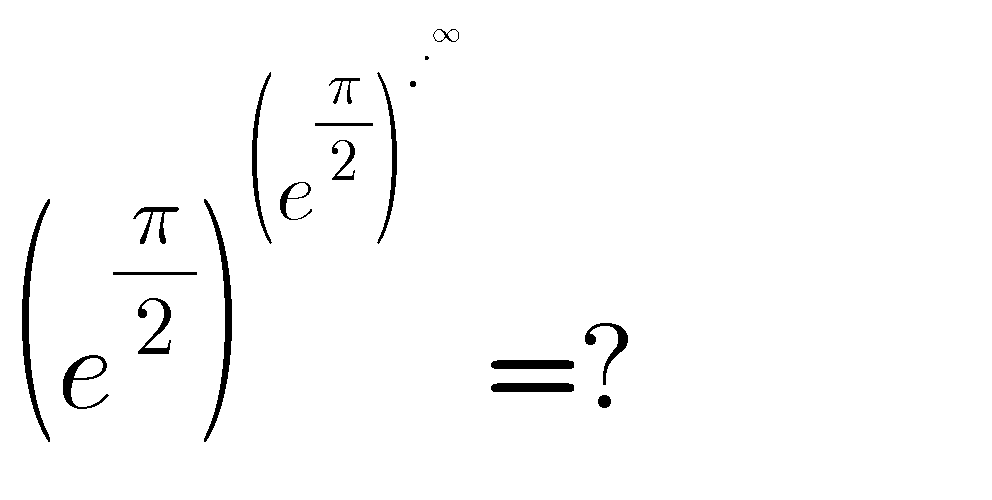
Question Number 80145 Answers: 1 Comments: 5

{ (((x/a)+(y/b)=a^2 +b^2 )),(( [a,b∈R])),((ab(x^2 −y^2 )=xy(a^2 −b^2 ))) :}

$$\begin{cases}{\frac{\boldsymbol{\mathrm{x}}}{\boldsymbol{\mathrm{a}}}+\frac{\boldsymbol{\mathrm{y}}}{\boldsymbol{\mathrm{b}}}=\boldsymbol{\mathrm{a}}^{\mathrm{2}} +\boldsymbol{\mathrm{b}}^{\mathrm{2}} }\\{\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left[\boldsymbol{\mathrm{a}},\boldsymbol{\mathrm{b}}\in\boldsymbol{\mathrm{R}}\right]}\\{\boldsymbol{\mathrm{ab}}\left(\boldsymbol{\mathrm{x}}^{\mathrm{2}} −\boldsymbol{\mathrm{y}}^{\mathrm{2}} \right)=\boldsymbol{\mathrm{xy}}\left(\boldsymbol{\mathrm{a}}^{\mathrm{2}} −\boldsymbol{\mathrm{b}}^{\mathrm{2}} \right)}\end{cases} \\ $$

Question Number 80119 Answers: 2 Comments: 0

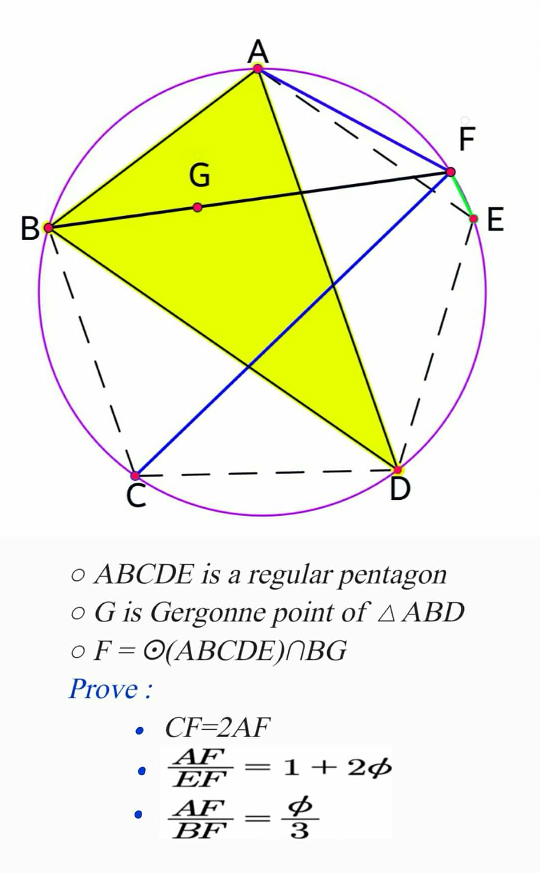
Question Number 80144 Answers: 0 Comments: 1

solve for x: (((√x)+1)/(√(x+1)))+ax^2 =x(a^2 +1) [a∈R]

$$\boldsymbol{\mathrm{solve}}\:\boldsymbol{\mathrm{for}}\:\boldsymbol{\mathrm{x}}: \\ $$$$\frac{\sqrt{\boldsymbol{\mathrm{x}}}+\mathrm{1}}{\sqrt{\boldsymbol{\mathrm{x}}+\mathrm{1}}}+\boldsymbol{\mathrm{ax}}^{\mathrm{2}} =\boldsymbol{\mathrm{x}}\left(\boldsymbol{\mathrm{a}}^{\mathrm{2}} +\mathrm{1}\right)\:\:\:\:\:\:\left[\boldsymbol{\mathrm{a}}\in\boldsymbol{\mathrm{R}}\right] \\ $$

Question Number 80116 Answers: 1 Comments: 1

Find S_m =Σ_(n=0) ^∞ (1/(Π_(k=1) ^m (n+k)))=? (m≥2)

$${Find} \\ $$$${S}_{{m}} =\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\underset{{k}=\mathrm{1}} {\overset{{m}} {\prod}}\left({n}+{k}\right)}=? \\ $$$$\left({m}\geqslant\mathrm{2}\right) \\ $$

Question Number 80113 Answers: 0 Comments: 1

how do you simply sin (tan^(−1) (3x)+cos^(−1) (x)) ?

$${how}\:{do}\:{you}\:{simply} \\ $$$$\mathrm{sin}\:\left(\mathrm{tan}^{−\mathrm{1}} \left(\mathrm{3}{x}\right)+\mathrm{cos}^{−\mathrm{1}} \left({x}\right)\right)\:? \\ $$

Question Number 80102 Answers: 0 Comments: 0

Question Number 80093 Answers: 3 Comments: 0

Solve for x and y x^(√y) = 64 y^(√x) = 81

$$\mathrm{Solve}\:\mathrm{for}\:\:\mathrm{x}\:\mathrm{and}\:\mathrm{y} \\ $$$$\:\:\:\:\:\mathrm{x}^{\sqrt{\mathrm{y}}} \:\:\:=\:\:\mathrm{64} \\ $$$$\:\:\:\:\:\mathrm{y}^{\sqrt{\mathrm{x}}} \:\:\:=\:\mathrm{81} \\ $$

Question Number 80088 Answers: 0 Comments: 0

When the father was son′s age, the son was ten years old; when the son will be father′s age, the father will be seventy. What are their ages ?

$$\:{When}\:\:{the}\:\:{father}\:\:{was}\:{son}'{s}\:\:{age},\:\:{the}\:\:{son} \\ $$$$\:\:{was}\:\:{ten}\:\:{years}\:\:{old};\:\:{when}\:\:{the}\:\:{son}\:\:{will}\:\:{be}\:\:{father}'{s}\:\:{age}, \\ $$$$\:\:{the}\:\:{father}\:\:{will}\:\:{be}\:\:{seventy}. \\ $$$$\:\:{What}\:\:{are}\:\:{their}\:\:{ages}\:\:? \\ $$

Question Number 80084 Answers: 0 Comments: 3

−1=(−1)^1 =(−1)^(2/2) =((−1)^2 )^(1/2) =(1)^(1/2) = =(√1)=1 what do you think about this?

$$\:\:−\mathrm{1}=\left(−\mathrm{1}\right)^{\mathrm{1}} =\left(−\mathrm{1}\right)^{\frac{\mathrm{2}}{\mathrm{2}}} =\left(\left(−\mathrm{1}\right)^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{2}}} =\left(\mathrm{1}\right)^{\frac{\mathrm{1}}{\mathrm{2}}} = \\ $$$$=\sqrt{\mathrm{1}}=\mathrm{1}\:\: \\ $$$$\mathrm{what}\:\mathrm{do}\:\mathrm{you}\:\mathrm{think}\:\mathrm{about}\:\mathrm{this}? \\ $$

Question Number 80068 Answers: 2 Comments: 3

Question Number 80065 Answers: 0 Comments: 0

Question Number 80064 Answers: 1 Comments: 6

lim_(x→−∞) [(√(1−xe^x ))]

$$\underset{{x}\rightarrow−\infty} {\mathrm{lim}}\:\left[\sqrt{\mathrm{1}−{xe}^{{x}} \:}\right] \\ $$

Question Number 80057 Answers: 1 Comments: 2

Question Number 80053 Answers: 0 Comments: 4

Find integer x, y such that 2^x −y^2 =615

$${Find}\:{integer}\:{x},\:{y}\:{such}\:{that} \\ $$$$\mathrm{2}^{{x}} −{y}^{\mathrm{2}} =\mathrm{615} \\ $$

Question Number 80052 Answers: 0 Comments: 0

∫ e^(sin 2x) .cos x dx =

$$\int\:\mathrm{e}^{\mathrm{sin}\:\mathrm{2x}} .\mathrm{cos}\:\mathrm{x}\:\mathrm{dx}\:= \\ $$$$ \\ $$

Question Number 80108 Answers: 1 Comments: 3

a,b,c ∈R ((b+c+d)/a)=((a+c+d)/b)=((a+b+c)/d)=((a+b+d)/c)=r what is r?

$${a},{b},{c}\:\in\mathbb{R} \\ $$$$\frac{{b}+{c}+{d}}{{a}}=\frac{{a}+{c}+{d}}{{b}}=\frac{{a}+{b}+{c}}{{d}}=\frac{{a}+{b}+{d}}{{c}}={r} \\ $$$${what}\:{is}\:{r}? \\ $$

Question Number 80039 Answers: 1 Comments: 6

prove that (1+x)(1+(1/x))≥4

$${prove}\:{that} \\ $$$$\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+\frac{\mathrm{1}}{{x}}\right)\geqslant\mathrm{4} \\ $$

Question Number 80037 Answers: 0 Comments: 0

A matrix A= [(a_(ij) ) ] is an upper triangular matrix if

$$\mathrm{A}\:\mathrm{matrix}\:{A}=\begin{bmatrix}{{a}_{{ij}} }\end{bmatrix}\:\mathrm{is}\:\mathrm{an}\:\mathrm{upper}\:\mathrm{triangular} \\ $$$$\mathrm{matrix}\:\mathrm{if} \\ $$

Question Number 80036 Answers: 1 Comments: 3

Σ_(n=1) ^∞ (1/((n+1)(n+2)(n+3)))=

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\:\frac{\mathrm{1}}{\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)\left({n}+\mathrm{3}\right)}=\: \\ $$

Question Number 80027 Answers: 0 Comments: 4

find minimum value of (√(x^2 +4))+(√(x^2 −24x+153)) for x≥0 in R

$${find}\:{minimum} \\ $$$${value}\:{of}\:\sqrt{{x}^{\mathrm{2}} +\mathrm{4}}+\sqrt{{x}^{\mathrm{2}} −\mathrm{24}{x}+\mathrm{153}} \\ $$$${for}\:{x}\geqslant\mathrm{0}\:{in}\:\mathbb{R} \\ $$

Question Number 80015 Answers: 2 Comments: 2

Pg 1317 Pg 1318 Pg 1319 Pg 1320 Pg 1321 Pg 1322 Pg 1323 Pg 1324 Pg 1325 Pg 1326

Contact: info@tinkutara.com