Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1319

Question Number 80587 Answers: 1 Comments: 6

Question Number 80586 Answers: 0 Comments: 1

Question Number 80585 Answers: 0 Comments: 9

Question Number 80580 Answers: 2 Comments: 0

Find general solution for k such that 7^k ≡1 mod (35)

$${Find}\:{general}\:{solution}\:{for}\:{k}\:{such}\:{that} \\ $$$$\mathrm{7}^{{k}} \equiv\mathrm{1}\:{mod}\:\left(\mathrm{35}\right) \\ $$

Question Number 80559 Answers: 0 Comments: 0

Question Number 80550 Answers: 1 Comments: 1

Question Number 80574 Answers: 1 Comments: 2

Question Number 80543 Answers: 1 Comments: 6

Question Number 80540 Answers: 0 Comments: 1

Question Number 80539 Answers: 0 Comments: 1

Question Number 80529 Answers: 0 Comments: 1

Question Number 80519 Answers: 2 Comments: 2

g(x)=2cos^2 x+sin(2x). g′(x)= ..........?

$$\mathrm{g}\left({x}\right)=\mathrm{2}{cos}^{\mathrm{2}} {x}+{sin}\left(\mathrm{2}{x}\right). \\ $$$${g}'\left({x}\right)=\:..........? \\ $$

Question Number 80515 Answers: 0 Comments: 1

∫(dx/((1+x^φ )^φ ))

$$\int\frac{{dx}}{\left(\mathrm{1}+{x}^{\phi} \right)^{\phi} } \\ $$

Question Number 80508 Answers: 0 Comments: 4

solve the D.E x^2 +(y^2 +1)dx+y(√(x^3 +1)) dy=0

$${solve}\:{the}\:{D}.{E}\: \\ $$$${x}^{\mathrm{2}} +\left({y}^{\mathrm{2}} +\mathrm{1}\right){dx}+{y}\sqrt{{x}^{\mathrm{3}} +\mathrm{1}}\:{dy}=\mathrm{0} \\ $$

Question Number 80505 Answers: 0 Comments: 8

Given that 7^k ≡1 (mod 15) a) Write down three values of k. b) Find the general solution of the equation 7^k ≡ 1 (mod 15)

$$\mathrm{Given}\:\mathrm{that}\:\:\mathrm{7}^{{k}} \:\equiv\mathrm{1}\:\left(\mathrm{mod}\:\mathrm{15}\right) \\ $$$$\left.\mathrm{a}\right)\:\mathrm{Write}\:\mathrm{down}\:\mathrm{three}\:\mathrm{values}\:\mathrm{of}\:{k}. \\ $$$$\left.\mathrm{b}\right)\:\mathrm{Find}\:\mathrm{the}\:\mathrm{general}\:\mathrm{solution}\:\mathrm{of}\: \\ $$$$\mathrm{the}\:\mathrm{equation}\:\:\mathrm{7}^{{k}} \:\equiv\:\mathrm{1}\:\left({mod}\:\mathrm{15}\right) \\ $$

Question Number 80493 Answers: 0 Comments: 4

Solve for a, b and c a + b + c = (1/2) ..... (i) abc = − (1/4) ...... (iii) ab + ac + bc = (3/2) ...... (iv)

$$\mathrm{Solve}\:\mathrm{for}\:\:\mathrm{a},\:\mathrm{b}\:\mathrm{and}\:\mathrm{c} \\ $$$$\:\:\:\:\:\:\mathrm{a}\:+\:\mathrm{b}\:+\:\mathrm{c}\:\:=\:\:\frac{\mathrm{1}}{\mathrm{2}}\:\:\:\:\:\:.....\:\left(\mathrm{i}\right) \\ $$$$\:\:\:\:\:\:\:\mathrm{abc}\:\:\:=\:\:\:−\:\frac{\mathrm{1}}{\mathrm{4}}\:\:\:\:\:......\:\left(\mathrm{iii}\right) \\ $$$$\:\:\:\:\:\:\mathrm{ab}\:+\:\mathrm{ac}\:+\:\mathrm{bc}\:\:\:=\:\:\frac{\mathrm{3}}{\mathrm{2}}\:\:\:\:\:\:\:......\:\left(\mathrm{iv}\right) \\ $$

Question Number 80485 Answers: 0 Comments: 1

what is the king rule?

$${what}\:{is}\:{the}\:{king}\:\:{rule}? \\ $$

Question Number 80477 Answers: 0 Comments: 5

Question Number 80475 Answers: 0 Comments: 0

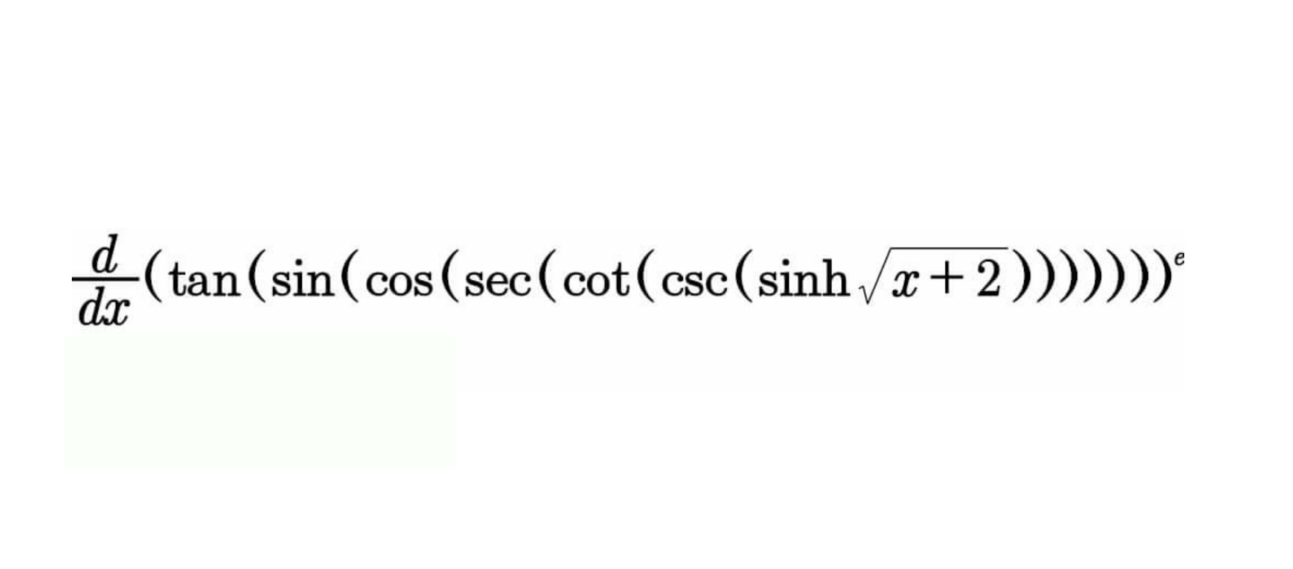
Question Number 80503 Answers: 1 Comments: 0

prove that they are infinitely many primes

$$\mathrm{prove}\:\mathrm{that}\:\mathrm{they}\:\mathrm{are}\:\mathrm{infinitely}\:\mathrm{many} \\ $$$$\mathrm{primes} \\ $$

Question Number 80504 Answers: 0 Comments: 2

Solve the system of congruences x ≡ 2 (mod 3) x ≡ 5( mod 7)

$$\mathrm{Solve}\:\mathrm{the}\:\mathrm{system}\:\mathrm{of}\:\mathrm{congruences} \\ $$$${x}\:\equiv\:\mathrm{2}\:\left(\mathrm{mod}\:\mathrm{3}\right) \\ $$$${x}\:\equiv\:\mathrm{5}\left(\:\mathrm{mod}\:\mathrm{7}\right) \\ $$$$\: \\ $$

Question Number 80501 Answers: 0 Comments: 0

(5/7) = (a_2 /(2!)) + (a_3 /(3!)) + (a_4 /(4!)) + (a_5 /(5!)) + (a_6 /(6!)) + (a_7 /(7!)) 0 ≤ a_i < i , a_i ∈ N Find possible value of a_2 + a_3 + a_4 + a_5 + a_6 + a_7 .

$$\frac{\mathrm{5}}{\mathrm{7}}\:\:=\:\:\frac{{a}_{\mathrm{2}} }{\mathrm{2}!}\:+\:\frac{{a}_{\mathrm{3}} }{\mathrm{3}!}\:+\:\frac{{a}_{\mathrm{4}} }{\mathrm{4}!}\:+\:\frac{{a}_{\mathrm{5}} }{\mathrm{5}!}\:+\:\frac{{a}_{\mathrm{6}} }{\mathrm{6}!}\:+\:\frac{{a}_{\mathrm{7}} }{\mathrm{7}!} \\ $$$$\mathrm{0}\:\:\leqslant\:{a}_{{i}} \:<\:{i}\:\:,\:\:{a}_{{i}} \:\:\in\:\mathbb{N} \\ $$$${Find}\:\:{possible}\:\:{value}\:\:{of}\:\:\:{a}_{\mathrm{2}} \:+\:{a}_{\mathrm{3}} \:+\:{a}_{\mathrm{4}} \:+\:{a}_{\mathrm{5}} \:+\:{a}_{\mathrm{6}} \:+\:{a}_{\mathrm{7}} \:\:. \\ $$

Question Number 80455 Answers: 1 Comments: 2

lim_(x→0) (((1+mx)/(1−nx)))^((mn)/x)

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left(\frac{\mathrm{1}+{mx}}{\mathrm{1}−{nx}}\right)^{\frac{{mn}}{{x}}} \\ $$

Question Number 80452 Answers: 0 Comments: 1

find ∫_(−∞) ^(+∞) ((cos(2x^2 +1))/(x^4 −x^2 +3))dx

$${find}\:\:\int_{−\infty} ^{+\infty} \:\:\:\frac{{cos}\left(\mathrm{2}{x}^{\mathrm{2}} +\mathrm{1}\right)}{{x}^{\mathrm{4}} −{x}^{\mathrm{2}} \:+\mathrm{3}}{dx} \\ $$

Question Number 80451 Answers: 0 Comments: 1

calculate ∫_0 ^∞ ((cos(πx))/((x^2 +3)^2 ))dx

$${calculate}\:\:\int_{\mathrm{0}} ^{\infty} \:\:\:\:\frac{{cos}\left(\pi{x}\right)}{\left({x}^{\mathrm{2}} +\mathrm{3}\right)^{\mathrm{2}} }{dx} \\ $$

Question Number 80448 Answers: 1 Comments: 3

Hello All of You verry Nice Day, God bless You love peace and happiness Solve for (x,y)∈R^2 { ((x^2 +y^2 =2x+3y+1)),((x^4 +y^4 =4x^2 +9y^2 +12xy+2x^2 y^2 +18)) :}

$${Hello}\:{All}\:{of}\:{You}\:{verry}\:{Nice}\:{Day},\:{God}\:{bless}\:{You}\:{love}\:{peace}\:{and}\: \\ $$$${happiness}\: \\ $$$${Solve}\:{for}\:\left({x},{y}\right)\in\mathbb{R}^{\mathrm{2}} \: \\ $$$$\begin{cases}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} =\mathrm{2}{x}+\mathrm{3}{y}+\mathrm{1}}\\{{x}^{\mathrm{4}} +{y}^{\mathrm{4}} =\mathrm{4}{x}^{\mathrm{2}} +\mathrm{9}{y}^{\mathrm{2}} +\mathrm{12}{xy}+\mathrm{2}{x}^{\mathrm{2}} {y}^{\mathrm{2}} +\mathrm{18}}\end{cases} \\ $$$$ \\ $$

Pg 1314 Pg 1315 Pg 1316 Pg 1317 Pg 1318 Pg 1319 Pg 1320 Pg 1321 Pg 1322 Pg 1323

Contact: info@tinkutara.com