
AllQuestion and Answers: Page 1318
Question Number 81320 Answers: 0 Comments: 0
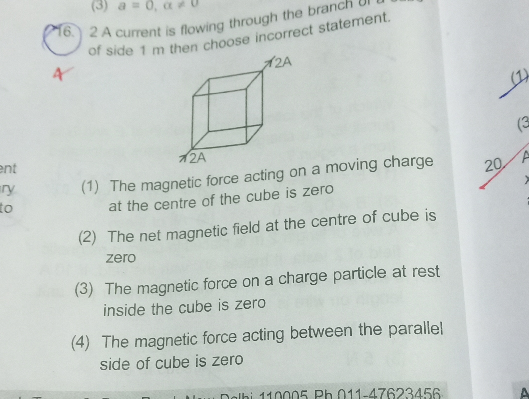
Question Number 81319 Answers: 1 Comments: 0
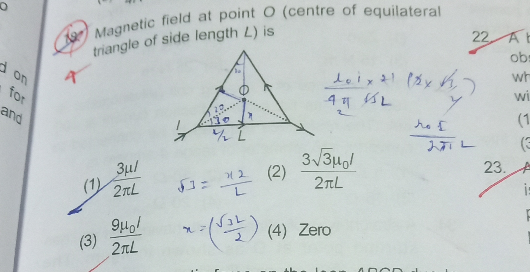
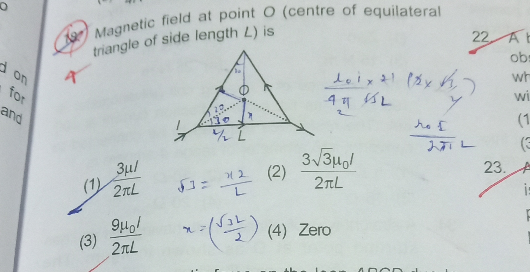
Question Number 81318 Answers: 0 Comments: 1

Question Number 81313 Answers: 1 Comments: 5
Question Number 81311 Answers: 0 Comments: 3
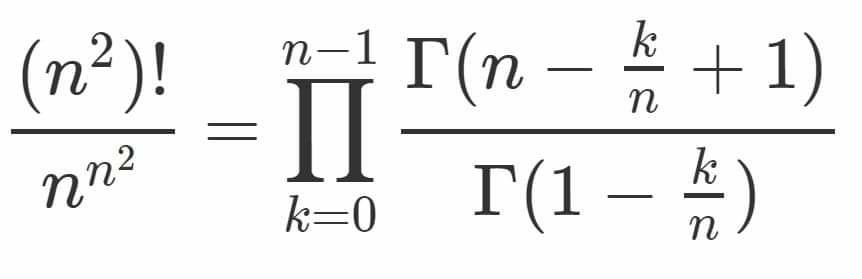
Question Number 81308 Answers: 1 Comments: 2

Question Number 81303 Answers: 1 Comments: 1

Question Number 81301 Answers: 0 Comments: 2

Question Number 81295 Answers: 1 Comments: 2
Question Number 81290 Answers: 0 Comments: 3
Question Number 81289 Answers: 0 Comments: 5
$${discussthesymmetryofthefollowingcurves} \\ $$$${squareofx}+{squareofy}=\mathrm{1} \\ $$
Question Number 81286 Answers: 1 Comments: 0
Question Number 81279 Answers: 0 Comments: 2
$$\int\:\frac{\sqrt{\mathrm{2}{x}+\mathrm{1}}}{\mathrm{3}{x}}\:{dx}\:=\:? \\ $$
Question Number 81275 Answers: 0 Comments: 0
Question Number 81274 Answers: 0 Comments: 0
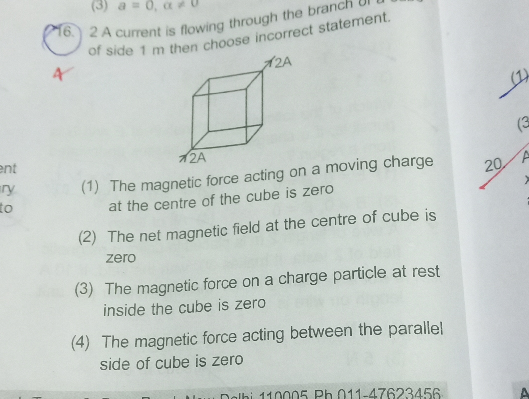
Question Number 81267 Answers: 0 Comments: 5
Question Number 81242 Answers: 1 Comments: 3

Question Number 81226 Answers: 1 Comments: 0
Question Number 81221 Answers: 0 Comments: 1

Question Number 81219 Answers: 1 Comments: 4
Question Number 81217 Answers: 2 Comments: 0

Question Number 81251 Answers: 1 Comments: 7
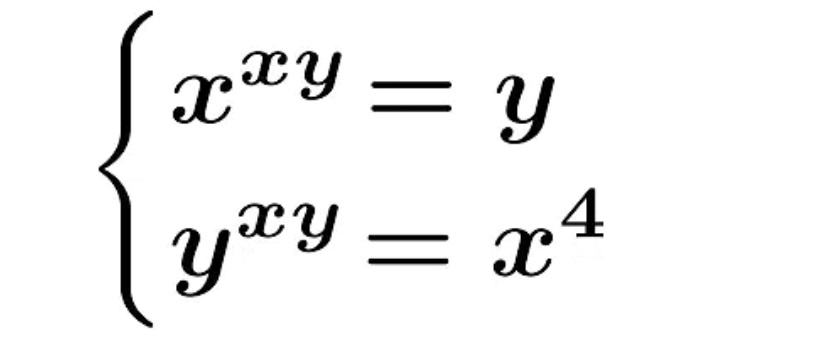
Question Number 81206 Answers: 1 Comments: 1

Question Number 81195 Answers: 0 Comments: 7
Question Number 81193 Answers: 1 Comments: 3

Question Number 81188 Answers: 2 Comments: 1
Pg 1313 Pg 1314 Pg 1315 Pg 1316 Pg 1317 Pg 1318 Pg 1319 Pg 1320 Pg 1321 Pg 1322
