
AllQuestion and Answers: Page 1317
Question Number 80828 Answers: 0 Comments: 2
Question Number 80823 Answers: 1 Comments: 0
Question Number 80816 Answers: 1 Comments: 2
Question Number 80815 Answers: 0 Comments: 0
Question Number 80814 Answers: 0 Comments: 2
Question Number 80813 Answers: 1 Comments: 0
Question Number 80809 Answers: 1 Comments: 5
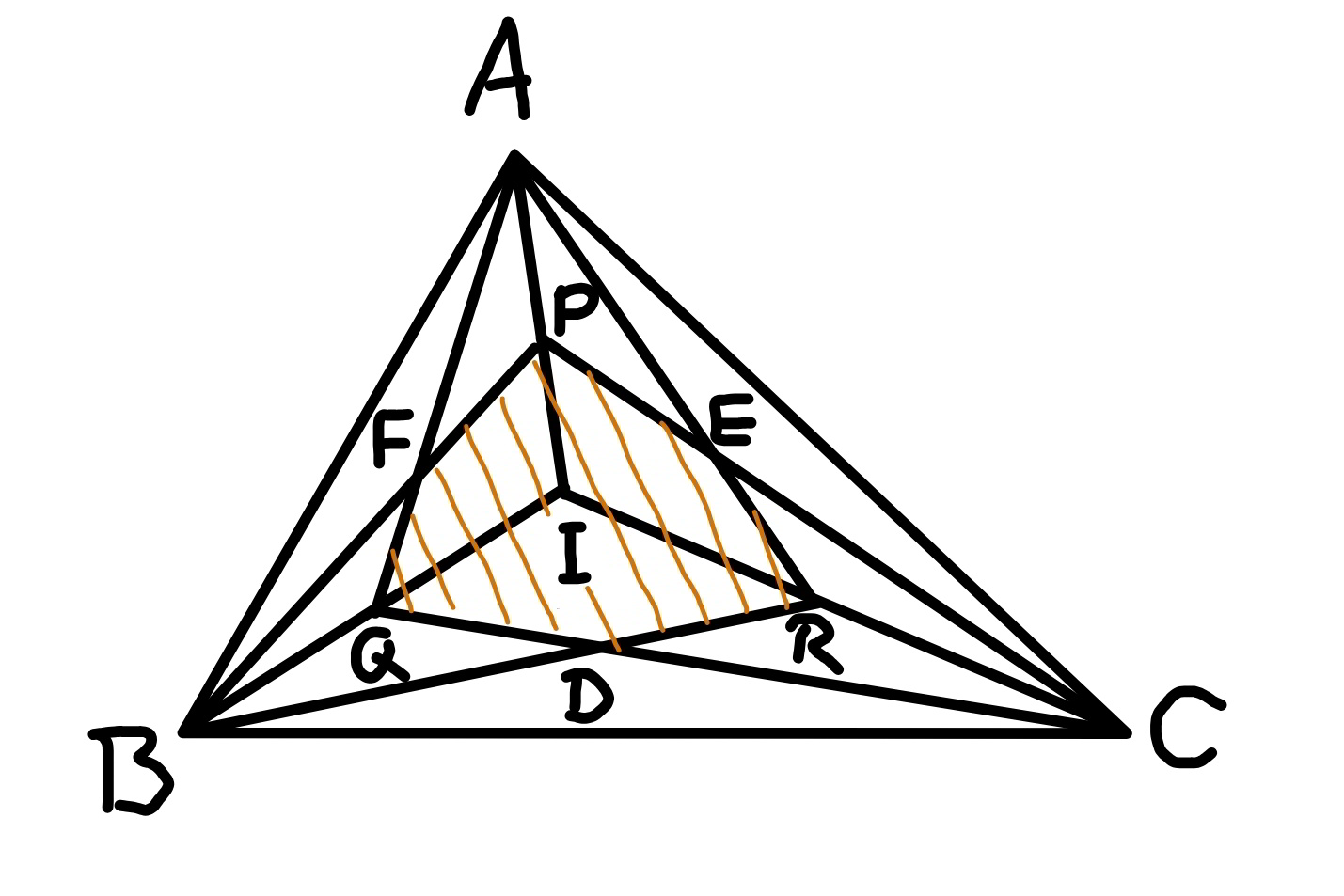
Question Number 80804 Answers: 2 Comments: 2
Question Number 80798 Answers: 0 Comments: 1
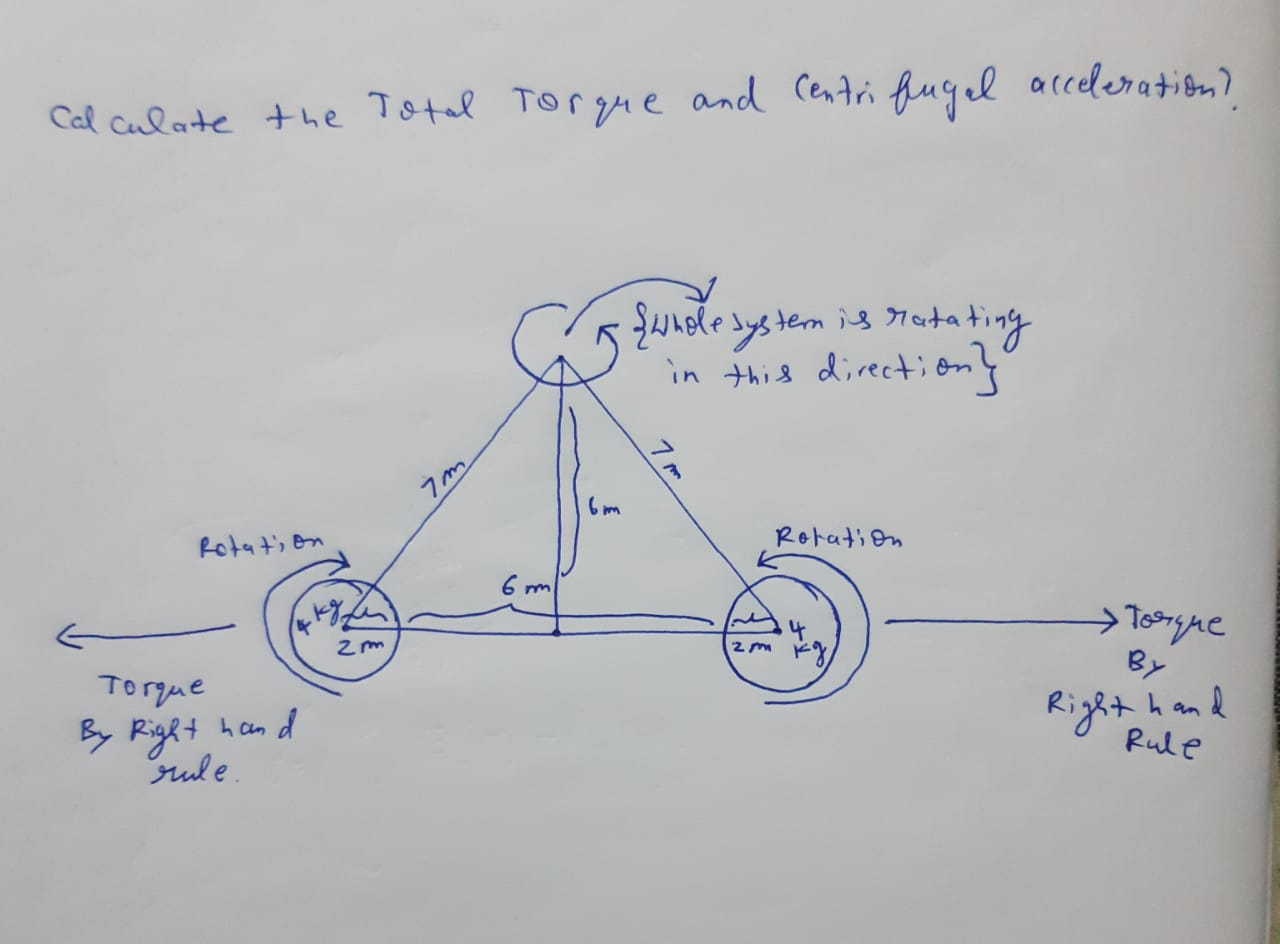
Question Number 80795 Answers: 0 Comments: 3

Question Number 80792 Answers: 1 Comments: 0
Question Number 80788 Answers: 0 Comments: 0

Question Number 80786 Answers: 1 Comments: 5

Question Number 80780 Answers: 1 Comments: 1
Question Number 80777 Answers: 1 Comments: 0
Question Number 80775 Answers: 0 Comments: 0
Question Number 80770 Answers: 1 Comments: 1
$$\int\mathrm{x}^{\mathrm{2}} +\mathrm{3x}\:\mathrm{dx}=.. \\ $$
Question Number 80764 Answers: 1 Comments: 0
Question Number 80761 Answers: 0 Comments: 1

Question Number 80760 Answers: 0 Comments: 1
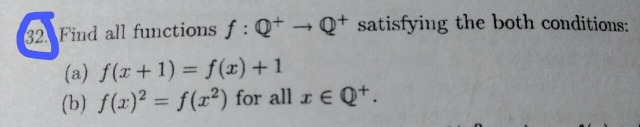
Question Number 80832 Answers: 1 Comments: 2
Question Number 80752 Answers: 1 Comments: 1

Question Number 80748 Answers: 1 Comments: 3
Question Number 80747 Answers: 1 Comments: 1
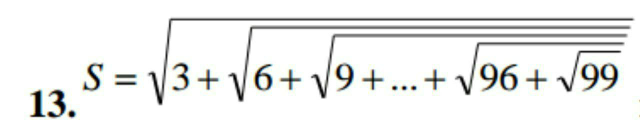
Question Number 80746 Answers: 1 Comments: 2
Question Number 80739 Answers: 0 Comments: 2
Pg 1312 Pg 1313 Pg 1314 Pg 1315 Pg 1316 Pg 1317 Pg 1318 Pg 1319 Pg 1320 Pg 1321
