
AllQuestion and Answers: Page 1311
Question Number 82057 Answers: 2 Comments: 1

Question Number 82056 Answers: 0 Comments: 0
Question Number 82042 Answers: 0 Comments: 4

Question Number 82041 Answers: 1 Comments: 4
$${show}\:{that} \\ $$$$\pi^{{ie}} +\frac{\mathrm{1}}{\mathrm{2}}=\mathrm{0} \\ $$
Question Number 82034 Answers: 0 Comments: 1
Question Number 82026 Answers: 1 Comments: 0
Question Number 82022 Answers: 0 Comments: 0

Question Number 82020 Answers: 0 Comments: 2
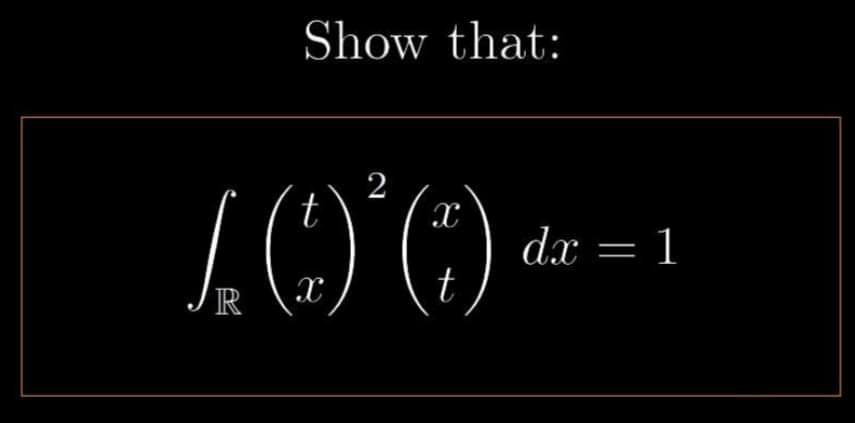
Question Number 82019 Answers: 1 Comments: 0

Question Number 82018 Answers: 2 Comments: 2
Question Number 81996 Answers: 0 Comments: 0
Question Number 81994 Answers: 0 Comments: 0
Question Number 81993 Answers: 0 Comments: 0
Question Number 81983 Answers: 0 Comments: 4

Question Number 81980 Answers: 1 Comments: 2
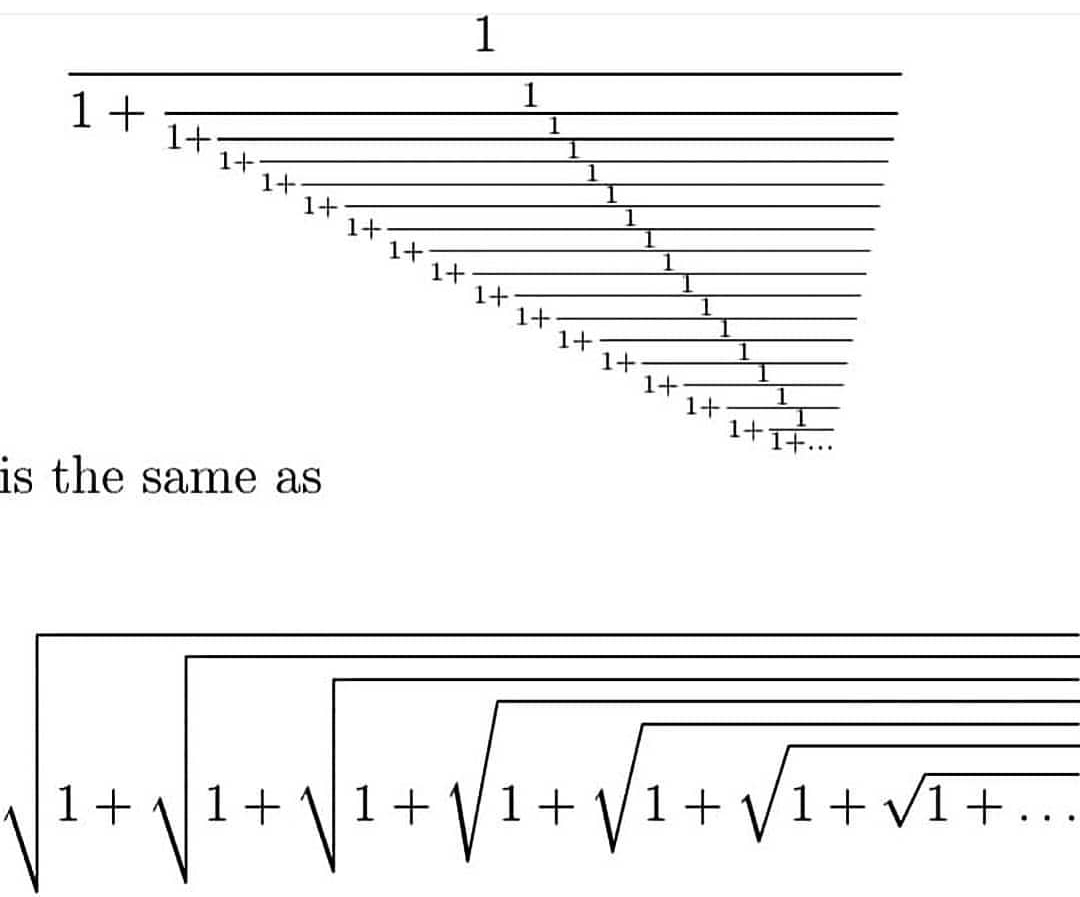
Question Number 82031 Answers: 1 Comments: 0
Question Number 82030 Answers: 1 Comments: 0
Question Number 81975 Answers: 2 Comments: 5

Question Number 81972 Answers: 1 Comments: 1

Question Number 81971 Answers: 1 Comments: 0
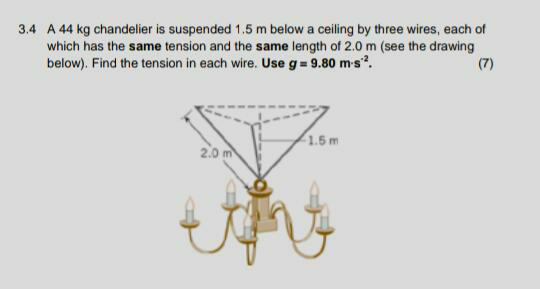
Question Number 81970 Answers: 0 Comments: 1
Question Number 81968 Answers: 1 Comments: 1
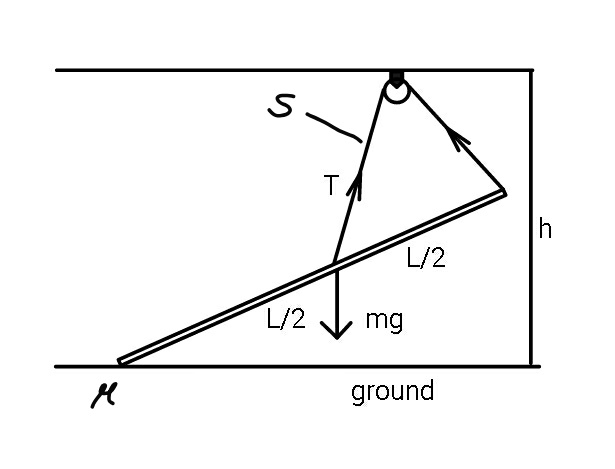
Question Number 81966 Answers: 1 Comments: 0
Question Number 81963 Answers: 1 Comments: 2
Question Number 82130 Answers: 0 Comments: 5
Question Number 81954 Answers: 0 Comments: 6
Pg 1306 Pg 1307 Pg 1308 Pg 1309 Pg 1310 Pg 1311 Pg 1312 Pg 1313 Pg 1314 Pg 1315
