Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1302

Question Number 83095 Answers: 0 Comments: 0

∫cosec x^5 dx

$$\int\mathrm{cosec}\:{x}^{\mathrm{5}} {dx} \\ $$

Question Number 83094 Answers: 0 Comments: 0

∫cosec x^5 dx

$$\int\mathrm{cosec}\:{x}^{\mathrm{5}} {dx} \\ $$

Question Number 83093 Answers: 0 Comments: 1

∫cosec x^5 dx

$$\int\mathrm{cosec}\:{x}^{\mathrm{5}} {dx} \\ $$

Question Number 83092 Answers: 0 Comments: 0

∫cosec x^5 dx

$$\int\mathrm{cosec}\:{x}^{\mathrm{5}} {dx} \\ $$

Question Number 83085 Answers: 1 Comments: 2

1) find ∫ (dx/((x^2 +1)^4 )) 2)calculate ∫_0 ^∞ (dx/((x^2 +1)^4 ))

$$\left.\mathrm{1}\right)\:{find}\:\int\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{4}} } \\ $$$$\left.\mathrm{2}\right){calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{dx}}{\left({x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{4}} } \\ $$

Question Number 83078 Answers: 0 Comments: 1

If I_1 =∫_e ^e^2 (dx/(log x)) and I_2 = ∫_( 1) ^2 (e^x /x) dx, then

$$\mathrm{If}\:\:\:{I}_{\mathrm{1}} =\underset{{e}} {\overset{{e}^{\mathrm{2}} } {\int}}\:\frac{{dx}}{\mathrm{log}\:{x}}\:\:\mathrm{and}\:\:{I}_{\mathrm{2}} =\:\underset{\:\mathrm{1}} {\overset{\mathrm{2}} {\int}}\:\frac{{e}^{{x}} }{{x}}\:{dx},\:\mathrm{then} \\ $$

Question Number 83077 Answers: 1 Comments: 0

If I_1 =∫_e ^e^2 (dx/(log x)) and I_2 = ∫_( 1) ^2 (e^x /x) dx, then

Question Number 83075 Answers: 1 Comments: 1

∫_(−1 ) ^3 (tan^(−1) (x/(x^2 +1)) + tan^(−1) ((x^2 +1)/x))dx =

$$\underset{−\mathrm{1}\:\:} {\overset{\mathrm{3}} {\int}}\:\left(\mathrm{tan}^{−\mathrm{1}} \frac{{x}}{{x}^{\mathrm{2}} +\mathrm{1}}\:+\:\mathrm{tan}^{−\mathrm{1}} \:\frac{{x}^{\mathrm{2}} +\mathrm{1}}{{x}}\right){dx}\:= \\ $$

Question Number 83074 Answers: 0 Comments: 2

find all function satisfying ∀ x∈R\{kπ , k∈Z} f(x)+∫_0 ^1 f^2 (x)dx=(x/(sin(πx)))

$${find}\:{all}\:{function}\:\:{satisfying}\:\:\forall\:{x}\in\mathbb{R}\backslash\left\{{k}\pi\:,\:\:{k}\in\mathbb{Z}\right\} \\ $$$${f}\left({x}\right)+\int_{\mathrm{0}} ^{\mathrm{1}} {f}^{\mathrm{2}} \left({x}\right){dx}=\frac{{x}}{{sin}\left(\pi{x}\right)} \\ $$

Question Number 83064 Answers: 2 Comments: 0

show that ∫_0 ^(π/2) ((sin(nx))/(sin(x)))dx=(π/2) n is posative odd number

$${show}\:{that} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{sin}\left({nx}\right)}{{sin}\left({x}\right)}{dx}=\frac{\pi}{\mathrm{2}} \\ $$$${n}\:{is}\:{posative}\:{odd}\:{number} \\ $$

Question Number 83063 Answers: 1 Comments: 3

Question Number 83050 Answers: 0 Comments: 1

Question Number 83049 Answers: 0 Comments: 0

Question Number 83042 Answers: 0 Comments: 1

let a,b two positive reals such as a^2 −b^2 =ab Explicit f(a,b)=∫_0 ^(π/2) (du/(√(a+bsin^2 u)))

$${let}\:\:{a},{b}\:{two}\:{positive}\:{reals}\:{such}\:{as}\:\:{a}^{\mathrm{2}} −{b}^{\mathrm{2}} ={ab} \\ $$$${Explicit}\:\:\:{f}\left({a},{b}\right)=\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{du}}{\sqrt{{a}+{bsin}^{\mathrm{2}} {u}}}\: \\ $$

Question Number 83037 Answers: 0 Comments: 3

If m=((1−cosθ)/(sinθ)) , show that (1/m)= ((1+sinθ)/(sinθ))

$$\mathrm{If}\:\mathrm{m}=\frac{\mathrm{1}−\mathrm{cos}\theta}{\mathrm{sin}\theta}\:,\:\:\mathrm{show}\:\mathrm{that}\:\frac{\mathrm{1}}{\mathrm{m}}=\:\frac{\mathrm{1}+\mathrm{sin}\theta}{\mathrm{sin}\theta} \\ $$

Question Number 83036 Answers: 0 Comments: 3

Prove that cos^4 θ−sin^4 θ=cos^2 θ−sin^2 θ

$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{cos}^{\mathrm{4}} \theta−\mathrm{sin}^{\mathrm{4}} \theta=\mathrm{cos}^{\mathrm{2}} \theta−\mathrm{sin}^{\mathrm{2}} \theta \\ $$

Question Number 83035 Answers: 1 Comments: 1

Question Number 83032 Answers: 1 Comments: 0

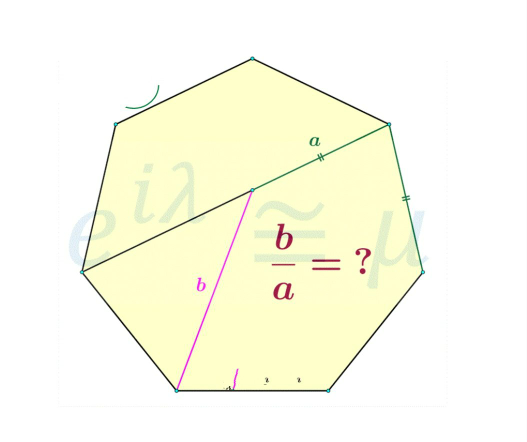
Question Number 83030 Answers: 1 Comments: 0

Question Number 83028 Answers: 0 Comments: 3

(dy/dx) + y sec x = tan x

$$\frac{\mathrm{dy}}{\mathrm{dx}}\:+\:\mathrm{y}\:\mathrm{sec}\:\mathrm{x}\:=\:\mathrm{tan}\:\mathrm{x} \\ $$

Question Number 83026 Answers: 0 Comments: 0

Prove that 123456..201820192020 divided by 13 , the remainder is 5 .

$${Prove}\:\:{that} \\ $$$$\:\:\:\:\:\mathrm{123456}..\mathrm{201820192020}\:\:{divided}\:\:{by}\:\:\mathrm{13}\:\:,\:\:{the} \\ $$$${remainder}\:\:{is}\:\:\mathrm{5}\:. \\ $$

Question Number 83021 Answers: 0 Comments: 0

find the sequence v_n wich verify v_n +v_(n+1) =(((−1)^n )/(√n)) (n≥1) is (v_n ) convergente?

$${find}\:{the}\:{sequence}\:{v}_{{n}} \:{wich}\:{verify}\:{v}_{{n}} +{v}_{{n}+\mathrm{1}} =\frac{\left(−\mathrm{1}\right)^{{n}} }{\sqrt{{n}}}\:\:\left({n}\geqslant\mathrm{1}\right) \\ $$$${is}\:\left({v}_{{n}} \right)\:{convergente}? \\ $$

Question Number 83020 Answers: 0 Comments: 0

find the sequence u_n wich verify u_n +u_(n+1) =((sin(n))/n) ∀n>0

$${find}\:{the}\:{sequence}\:{u}_{{n}} \:{wich}\:{verify}\:{u}_{{n}} +{u}_{{n}+\mathrm{1}} =\frac{{sin}\left({n}\right)}{{n}}\:\:\forall{n}>\mathrm{0} \\ $$

Question Number 83019 Answers: 1 Comments: 1

find lim_(x→0) ((1−(√(1+x+x^2 ))cos(2x))/x^3 )

$${find}\:{lim}_{{x}\rightarrow\mathrm{0}} \:\:\frac{\mathrm{1}−\sqrt{\mathrm{1}+{x}+{x}^{\mathrm{2}} }{cos}\left(\mathrm{2}{x}\right)}{{x}^{\mathrm{3}} } \\ $$

Question Number 83010 Answers: 0 Comments: 0

calculate ∫_0 ^∞ ((ch(cos(2x)))/((x^2 +1)^2 ))dx

$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{ch}\left({cos}\left(\mathrm{2}{x}\right)\right)}{\left({x}^{\mathrm{2}} \:+\mathrm{1}\right)^{\mathrm{2}} }{dx} \\ $$

Question Number 83009 Answers: 0 Comments: 1

calculate ∫_0 ^∞ ((cos(chx))/(x^2 +3))dx

$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{{cos}\left({chx}\right)}{{x}^{\mathrm{2}} \:+\mathrm{3}}{dx} \\ $$

Pg 1297 Pg 1298 Pg 1299 Pg 1300 Pg 1301 Pg 1302 Pg 1303 Pg 1304 Pg 1305 Pg 1306

Contact: info@tinkutara.com