
AllQuestion and Answers: Page 1271
Question Number 84879 Answers: 2 Comments: 1
Question Number 84873 Answers: 2 Comments: 1
Question Number 84871 Answers: 1 Comments: 1
Question Number 84868 Answers: 1 Comments: 0
Question Number 84862 Answers: 2 Comments: 1
Question Number 84859 Answers: 0 Comments: 0
Question Number 84849 Answers: 0 Comments: 5
Question Number 84845 Answers: 1 Comments: 1
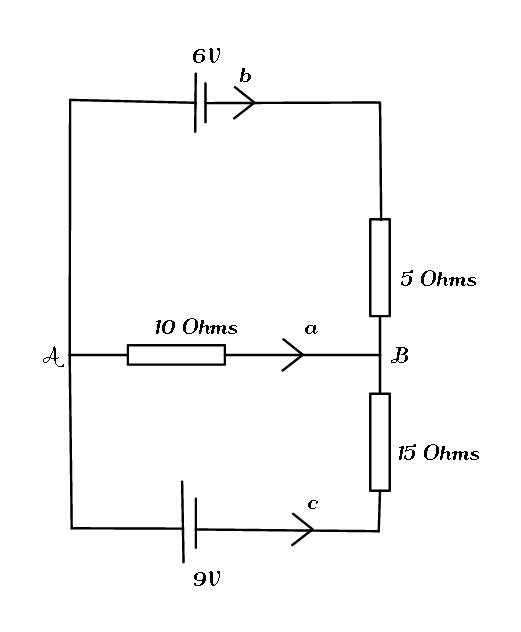
Question Number 84843 Answers: 0 Comments: 2
$$\int\frac{{sin}\left(\mathrm{7}{x}\right)}{{cos}\left(\mathrm{3}{x}\right)}\:{dx} \\ $$
Question Number 84835 Answers: 0 Comments: 1

Question Number 84834 Answers: 1 Comments: 1
Question Number 84830 Answers: 1 Comments: 0
Question Number 84828 Answers: 0 Comments: 1
Question Number 84826 Answers: 0 Comments: 1
Question Number 84820 Answers: 1 Comments: 0

Question Number 84814 Answers: 0 Comments: 1
Question Number 84810 Answers: 0 Comments: 1
Question Number 84809 Answers: 1 Comments: 1
Question Number 84861 Answers: 0 Comments: 1
Question Number 84792 Answers: 0 Comments: 0
Question Number 84783 Answers: 1 Comments: 6

Question Number 84782 Answers: 1 Comments: 8
$${Find}\:{the}\:{last}\:{three}\:{digits}\:{of}\:\mathrm{2019}^{\mathrm{2019}} . \\ $$
Question Number 84778 Answers: 0 Comments: 0
Question Number 84770 Answers: 2 Comments: 1
Question Number 84767 Answers: 1 Comments: 0
Question Number 84766 Answers: 1 Comments: 2
Pg 1266 Pg 1267 Pg 1268 Pg 1269 Pg 1270 Pg 1271 Pg 1272 Pg 1273 Pg 1274 Pg 1275
