
AllQuestion and Answers: Page 1265
Question Number 87378 Answers: 1 Comments: 1
Question Number 87371 Answers: 1 Comments: 3
Question Number 87369 Answers: 1 Comments: 4

Question Number 87358 Answers: 0 Comments: 0
Question Number 87352 Answers: 1 Comments: 6
Question Number 87340 Answers: 3 Comments: 3
Question Number 87335 Answers: 1 Comments: 0
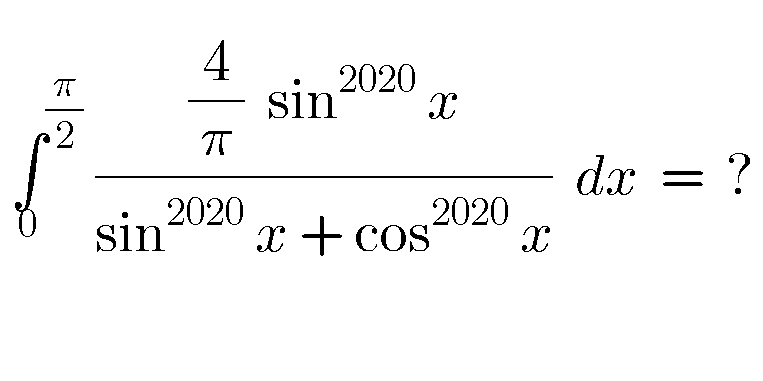
Question Number 87325 Answers: 1 Comments: 3
Question Number 87322 Answers: 0 Comments: 0
Question Number 87321 Answers: 0 Comments: 0
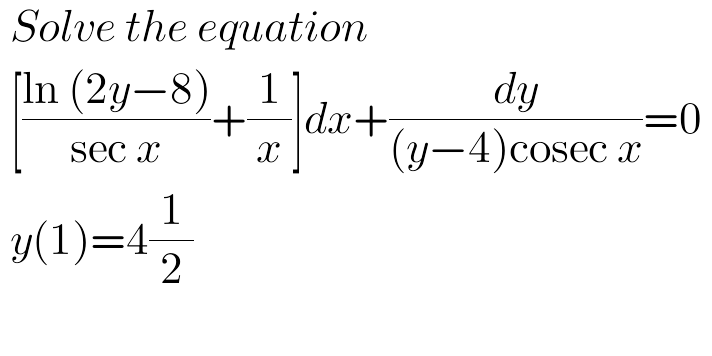
Question Number 87316 Answers: 0 Comments: 0

Question Number 87313 Answers: 1 Comments: 4
Question Number 87308 Answers: 0 Comments: 0
Question Number 87306 Answers: 1 Comments: 9
Question Number 87302 Answers: 1 Comments: 3
Question Number 87301 Answers: 0 Comments: 3

Question Number 87298 Answers: 1 Comments: 0
Question Number 87296 Answers: 1 Comments: 0
Question Number 87285 Answers: 1 Comments: 1
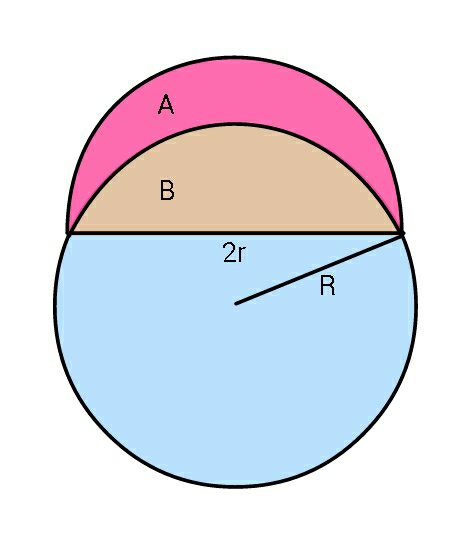
Question Number 87279 Answers: 3 Comments: 2
$$\int\frac{{x}^{\mathrm{2}} }{\mathrm{1}+{x}^{\mathrm{4}} }{dx} \\ $$
Question Number 87275 Answers: 1 Comments: 0
Question Number 87274 Answers: 0 Comments: 0
Question Number 87273 Answers: 0 Comments: 0
Question Number 87272 Answers: 1 Comments: 0
Question Number 87253 Answers: 1 Comments: 0
Question Number 87250 Answers: 0 Comments: 0
Pg 1260 Pg 1261 Pg 1262 Pg 1263 Pg 1264 Pg 1265 Pg 1266 Pg 1267 Pg 1268 Pg 1269
