
AllQuestion and Answers: Page 1241
Question Number 90195 Answers: 0 Comments: 0

Question Number 90194 Answers: 1 Comments: 0
Question Number 90196 Answers: 0 Comments: 0
Question Number 90192 Answers: 1 Comments: 1
Question Number 90178 Answers: 1 Comments: 1

Question Number 90171 Answers: 2 Comments: 0
Question Number 90168 Answers: 1 Comments: 4
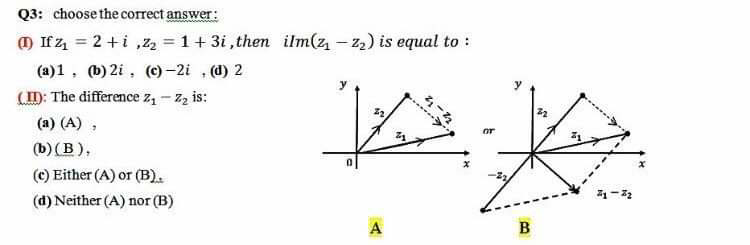
Question Number 90158 Answers: 1 Comments: 2

Question Number 90157 Answers: 0 Comments: 0
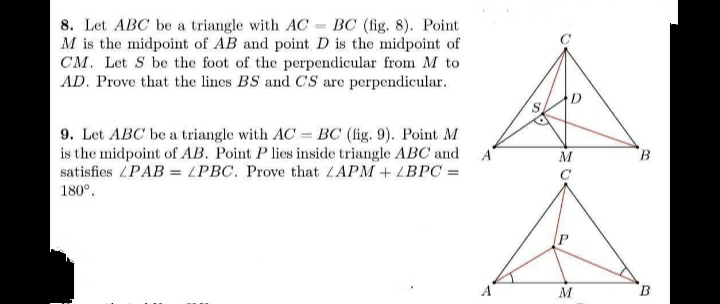
Question Number 90151 Answers: 1 Comments: 1
Question Number 90148 Answers: 0 Comments: 1

Question Number 90139 Answers: 0 Comments: 3
Question Number 90138 Answers: 0 Comments: 2
$$\int{x}\sqrt{\mathrm{3}{x}^{\mathrm{3}} +\mathrm{7}}\:{dx} \\ $$
Question Number 90135 Answers: 1 Comments: 0
Question Number 90134 Answers: 0 Comments: 0

Question Number 90122 Answers: 0 Comments: 1
Question Number 90162 Answers: 1 Comments: 0

Question Number 90160 Answers: 1 Comments: 0
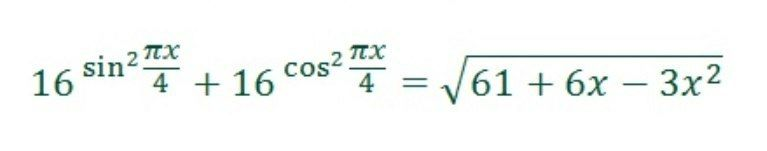
Question Number 90161 Answers: 1 Comments: 0
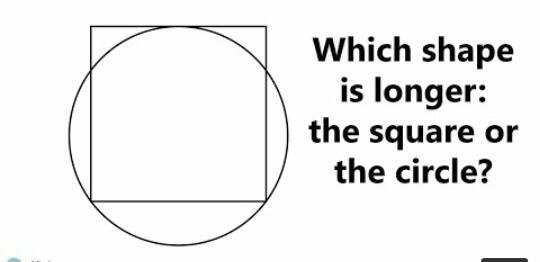
Question Number 90113 Answers: 1 Comments: 0
Question Number 90110 Answers: 0 Comments: 2
Question Number 90103 Answers: 0 Comments: 1

Question Number 90100 Answers: 1 Comments: 0
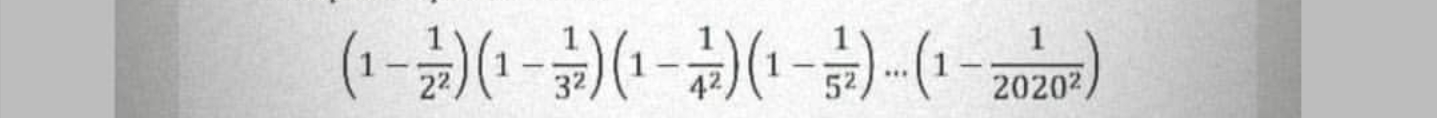
Question Number 90099 Answers: 0 Comments: 2
Question Number 90097 Answers: 0 Comments: 1
Question Number 90090 Answers: 1 Comments: 0
Pg 1236 Pg 1237 Pg 1238 Pg 1239 Pg 1240 Pg 1241 Pg 1242 Pg 1243 Pg 1244 Pg 1245
