Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1231

Question Number 90810 Answers: 1 Comments: 2

(dy/dx) = ((2x^2 +y^2 )/(3x^2 +2xy))

$$\frac{{dy}}{{dx}}\:=\:\frac{\mathrm{2}{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }{\mathrm{3}{x}^{\mathrm{2}} +\mathrm{2}{xy}}\: \\ $$

Question Number 90806 Answers: 0 Comments: 1

log_((x+4)) (x^2 −8x+12) < (1/2)log_(∣x−2∣) (2−x)^2

$$\mathrm{log}_{\left({x}+\mathrm{4}\right)} \left({x}^{\mathrm{2}} −\mathrm{8}{x}+\mathrm{12}\right)\:<\:\frac{\mathrm{1}}{\mathrm{2}}\mathrm{log}_{\mid{x}−\mathrm{2}\mid} \left(\mathrm{2}−{x}\right)^{\mathrm{2}} \\ $$

Question Number 90803 Answers: 0 Comments: 0

U=ax−bx^c +dy is subject to I=mx+ny. What happens to the maximum point of x, when a increases? Explain your answer. (Use Lagrange method)

$${U}={ax}−{bx}^{{c}} +{dy}\:\mathrm{is}\:\mathrm{subject}\:\mathrm{to}\:\mathrm{I}={mx}+{ny}. \\ $$$$ \\ $$$$\mathrm{What}\:\mathrm{happens}\:\mathrm{to}\:\mathrm{the}\:\mathrm{maximum}\:\mathrm{point}\:\mathrm{of}\:\boldsymbol{{x}}, \\ $$$${when}\:\boldsymbol{{a}}\:\mathrm{increases}?\:\mathrm{Explain}\:\mathrm{your}\:\mathrm{answer}. \\ $$$$ \\ $$$$\left(\mathrm{Use}\:\mathrm{Lagrange}\:\mathrm{method}\right) \\ $$

Question Number 90802 Answers: 0 Comments: 2

1) ∫_(ln 2) ^∞ (1/(√(e^x −1))) dx 2) ∫_(ln 2) ^∞ (1/(e^x −1)) dx

$$\left.\mathrm{1}\right)\:\underset{\mathrm{ln}\:\mathrm{2}} {\overset{\infty} {\int}}\:\frac{\mathrm{1}}{\sqrt{\mathrm{e}^{{x}} −\mathrm{1}}}\:{dx}\: \\ $$$$\left.\mathrm{2}\right)\:\underset{\mathrm{ln}\:\mathrm{2}} {\overset{\infty} {\int}}\:\frac{\mathrm{1}}{{e}^{{x}} −\mathrm{1}}\:{dx}\: \\ $$

Question Number 90801 Answers: 0 Comments: 3

what are the next two number of this series 4,7,9,2,5,6,3,8 ?

$${what}\:{are}\:{the}\:{next}\:{two}\:{number}\: \\ $$$${of}\:{this}\:{series}\:\mathrm{4},\mathrm{7},\mathrm{9},\mathrm{2},\mathrm{5},\mathrm{6},\mathrm{3},\mathrm{8}\:? \\ $$

Question Number 90796 Answers: 2 Comments: 3

∫_0 ^(√3) (1/(1+x^2 ))sin^(−1) (((2x)/(1+x^2 )))dx

$$\int_{\mathrm{0}} ^{\sqrt{\mathrm{3}}} \frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{2}} }{sin}^{−\mathrm{1}} \left(\frac{\mathrm{2}{x}}{\mathrm{1}+{x}^{\mathrm{2}} }\right){dx} \\ $$

Question Number 90793 Answers: 1 Comments: 4

a+b+c+d=4 a^2 +b^2 +c^2 +d^2 =10 a^3 +b^3 +c^3 +d^3 =22 a^4 +b^4 +c^4 +d^4 = ?

$${a}+{b}+{c}+{d}=\mathrm{4} \\ $$$${a}^{\mathrm{2}} +{b}^{\mathrm{2}} +{c}^{\mathrm{2}} +{d}^{\mathrm{2}} =\mathrm{10} \\ $$$${a}^{\mathrm{3}} +{b}^{\mathrm{3}} +{c}^{\mathrm{3}} +{d}^{\mathrm{3}} =\mathrm{22} \\ $$$${a}^{\mathrm{4}} +{b}^{\mathrm{4}} +{c}^{\mathrm{4}} +{d}^{\mathrm{4}} =\:? \\ $$

Question Number 90788 Answers: 0 Comments: 1

lim_(x→−∞) (((x+2)/(x+1)))^(x/2)

$$\underset{{x}\rightarrow−\infty} {\mathrm{lim}}\:\left(\frac{{x}+\mathrm{2}}{{x}+\mathrm{1}}\right)^{\frac{{x}}{\mathrm{2}}} \\ $$

Question Number 90787 Answers: 1 Comments: 3

Find the infinite sum Σ_(n=1) ^∞ (1/((2n−1)(2n+1)(2n+3)))

$${Find}\:{the}\:{infinite}\:{sum} \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{\left(\mathrm{2}{n}−\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{3}\right)} \\ $$

Question Number 90784 Answers: 0 Comments: 2

∫_0 ^2 (√((4−x)/x))−(√(x/(4−x))) dx ?

$$\underset{\mathrm{0}} {\overset{\mathrm{2}} {\int}}\:\sqrt{\frac{\mathrm{4}−{x}}{{x}}}−\sqrt{\frac{{x}}{\mathrm{4}−{x}}}\:{dx}\:? \\ $$

Question Number 90783 Answers: 0 Comments: 1

can inflection point be a max or min ?

$${can}\:{inflection}\:{point}\: \\ $$$${be}\:{a}\:{max}\:{or}\:{min}\:?\: \\ $$

Question Number 90777 Answers: 0 Comments: 0

Question Number 90773 Answers: 1 Comments: 1

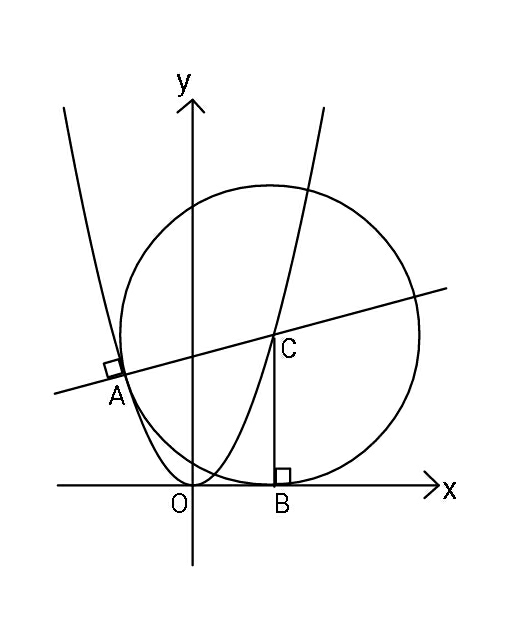
Question Number 90772 Answers: 0 Comments: 2

show that the roots of the equation x^2 −2x=(b−c)^2 −1 are rational if b and c are rational numbers.

$${show}\:{that}\:{the}\:{roots}\:{of}\:{the}\:{equation} \\ $$$${x}^{\mathrm{2}} −\mathrm{2}{x}=\left({b}−{c}\right)^{\mathrm{2}} −\mathrm{1}\:{are}\:{rational}\:{if} \\ $$$${b}\:{and}\:{c}\:{are}\:{rational}\:{numbers}. \\ $$

Question Number 90770 Answers: 1 Comments: 0

(ax+by+c)dx+(px+qy+r)dy=0

$$\left({ax}+{by}+{c}\right){dx}+\left({px}+{qy}+{r}\right){dy}=\mathrm{0} \\ $$

Question Number 90762 Answers: 1 Comments: 0

2^x +2^(3x) =16 solve for x

$$\mathrm{2}^{\mathrm{x}} +\mathrm{2}^{\mathrm{3x}} =\mathrm{16} \\ $$$$\mathrm{solve}\:\mathrm{for}\:\mathrm{x} \\ $$

Question Number 90759 Answers: 0 Comments: 1

1/∫_0 ^∞ ∫_x ^∞ ((cos(t) ln^2 (x))/(t(√x)))dt dx 2/∫_(1/2) ^(3/2) ln(Γ(x))dx=((ln(π)−1)/2) 3/∫_0 ^(π/2) cos(nt) cos^m (t) dt=((πΓ(m+1))/(2^(m+1) Γ(((n+m+2)/2))Γ(((2−n+m)/2)))) 4/∫_0 ^(+∞) ((x exp(πcos(πx) sin(πsin(πx)))/(x^2 +π^2 ))dx=(π/2)exp(πexp(−π^2 ))−1)

$$\mathrm{1}/\int_{\mathrm{0}} ^{\infty} \int_{{x}} ^{\infty} \frac{{cos}\left({t}\right)\:{ln}^{\mathrm{2}} \left({x}\right)}{{t}\sqrt{{x}}}{dt}\:{dx} \\ $$$$ \\ $$$$\mathrm{2}/\int_{\mathrm{1}/\mathrm{2}} ^{\mathrm{3}/\mathrm{2}} {ln}\left(\Gamma\left({x}\right)\right){dx}=\frac{{ln}\left(\pi\right)−\mathrm{1}}{\mathrm{2}} \\ $$$$ \\ $$$$\mathrm{3}/\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} {cos}\left({nt}\right)\:{cos}^{{m}} \left({t}\right)\:{dt}=\frac{\pi\Gamma\left({m}+\mathrm{1}\right)}{\mathrm{2}^{{m}+\mathrm{1}} \Gamma\left(\frac{{n}+{m}+\mathrm{2}}{\mathrm{2}}\right)\Gamma\left(\frac{\mathrm{2}−{n}+{m}}{\mathrm{2}}\right)} \\ $$$$ \\ $$$$\left.\mathrm{4}/\int_{\mathrm{0}} ^{+\infty} \frac{{x}\:{exp}\left(\pi{cos}\left(\pi{x}\right)\:{sin}\left(\pi{sin}\left(\pi{x}\right)\right)\right.}{{x}^{\mathrm{2}} +\pi^{\mathrm{2}} }{dx}=\frac{\pi}{\mathrm{2}}{exp}\left(\pi{exp}\left(−\pi^{\mathrm{2}} \right)\right)−\mathrm{1}\right) \\ $$

Question Number 90765 Answers: 0 Comments: 2

if ∫ (ln(x))^2 dx = x( ln^2 (x)+a ln(x)+b) +C a,b , C are constant. find the value of a and b

$${if}\:\int\:\left(\mathrm{ln}\left({x}\right)\right)^{\mathrm{2}} {dx}\:=\: \\ $$$${x}\left(\:\mathrm{ln}^{\mathrm{2}} \left({x}\right)+{a}\:\mathrm{ln}\left({x}\right)+{b}\right)\:+{C} \\ $$$${a},{b}\:,\:{C}\:{are}\:{constant}.\: \\ $$$${find}\:{the}\:{value}\:{of}\:{a}\:{and}\:{b}\: \\ $$

Question Number 90752 Answers: 1 Comments: 0

find ∫(1+x^2 )(√(1−x^2 ))dx

$${find}\:\int\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }{dx} \\ $$

Question Number 90751 Answers: 0 Comments: 1

calculste lim_(n→∞) (1/n^2 )Σ_(k=1) ^n karctan((k/n))

$$\:{calculste}\:{lim}_{{n}\rightarrow\infty} \:\:\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\sum_{{k}=\mathrm{1}} ^{{n}} \:{karctan}\left(\frac{{k}}{{n}}\right) \\ $$

Question Number 90750 Answers: 0 Comments: 0

let α and β roots of x^2 −x+2=0 calculate A_n =Σ_(k=0) ^n (α^k +β^k ) B_n = Σ_(k=0) ^n (α^k −β^k )

$${let}\:\:\alpha\:{and}\:\beta\:{roots}\:{of}\:\:{x}^{\mathrm{2}} −{x}+\mathrm{2}=\mathrm{0}\:\:{calculate} \\ $$$${A}_{{n}} =\sum_{{k}=\mathrm{0}} ^{{n}} \:\left(\alpha^{{k}} \:+\beta^{{k}} \right) \\ $$$${B}_{{n}} =\:\sum_{{k}=\mathrm{0}} ^{{n}} \left(\alpha^{{k}} −\beta^{{k}} \right) \\ $$

Question Number 90749 Answers: 0 Comments: 1

find Σ_(k=0) ^n C_n ^k cos(((kπ)/n))

$${find}\:\sum_{{k}=\mathrm{0}} ^{{n}} \:{C}_{{n}} ^{{k}} \:{cos}\left(\frac{{k}\pi}{{n}}\right) \\ $$

Question Number 90747 Answers: 1 Comments: 0

solve: t^(1/3) + t^(1/2) = 12

$$\mathrm{solve}:\:\:\:\mathrm{t}^{\mathrm{1}/\mathrm{3}} \:\:\:+\:\:\:\mathrm{t}^{\mathrm{1}/\mathrm{2}} \:\:\:=\:\:\:\mathrm{12} \\ $$

Question Number 90743 Answers: 1 Comments: 0

find nature of the serie Σ (−1)^n U_n with U_(n+1) =(e^(−U_n ) /(n+1)) (U_0 =1)

$${find}\:{nature}\:{of}\:{the}\:{serie}\:\Sigma\:\left(−\mathrm{1}\right)^{{n}} \:{U}_{{n}} \\ $$$${with}\:\:{U}_{{n}+\mathrm{1}} =\frac{{e}^{−{U}_{{n}} } }{{n}+\mathrm{1}}\:\:\:\:\:\left({U}_{\mathrm{0}} =\mathrm{1}\right) \\ $$

Question Number 90739 Answers: 1 Comments: 2

solve for x and y tan^2 [π(x+y)]+cot^2 [π(x+y)] =1+(√((2x)/(x^2 +1)))

$${solve}\:{for}\:{x}\:{and}\:{y} \\ $$$$\mathrm{tan}\:^{\mathrm{2}} \left[\pi\left({x}+{y}\right)\right]+\mathrm{cot}\:^{\mathrm{2}} \left[\pi\left({x}+{y}\right)\right] \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:=\mathrm{1}+\sqrt{\frac{\mathrm{2}{x}}{{x}^{\mathrm{2}} +\mathrm{1}}} \\ $$

Question Number 90730 Answers: 0 Comments: 0

let a ,b integer and C=a^2 +b^2 Prove that there exist a_n and b_n all integers such as C^n =a_n ^2 +b_n ^2 explicit a_5 and b_5 interm of a and b

$${let}\:{a}\:,{b}\:{integer}\:{and}\:\:{C}={a}^{\mathrm{2}} +{b}^{\mathrm{2}} \: \\ $$$${Prove}\:{that}\:{there}\:{exist}\:{a}_{{n}} \:{and}\:{b}_{{n}} \:{all}\: \\ $$$${integers}\:{such}\:{as}\:{C}^{{n}} ={a}_{{n}} ^{\mathrm{2}} \:+{b}_{{n}} ^{\mathrm{2}} \: \\ $$$${explicit}\:{a}_{\mathrm{5}} \:{and}\:{b}_{\mathrm{5}} \:{interm}\:{of}\:{a}\:{and}\:\:{b} \\ $$

Pg 1226 Pg 1227 Pg 1228 Pg 1229 Pg 1230 Pg 1231 Pg 1232 Pg 1233 Pg 1234 Pg 1235

Terms of Service

Contact: info@tinkutara.com