
AllQuestion and Answers: Page 1230
Question Number 91460 Answers: 1 Comments: 0
Question Number 91452 Answers: 2 Comments: 1
Question Number 91448 Answers: 0 Comments: 3
Question Number 91446 Answers: 0 Comments: 1
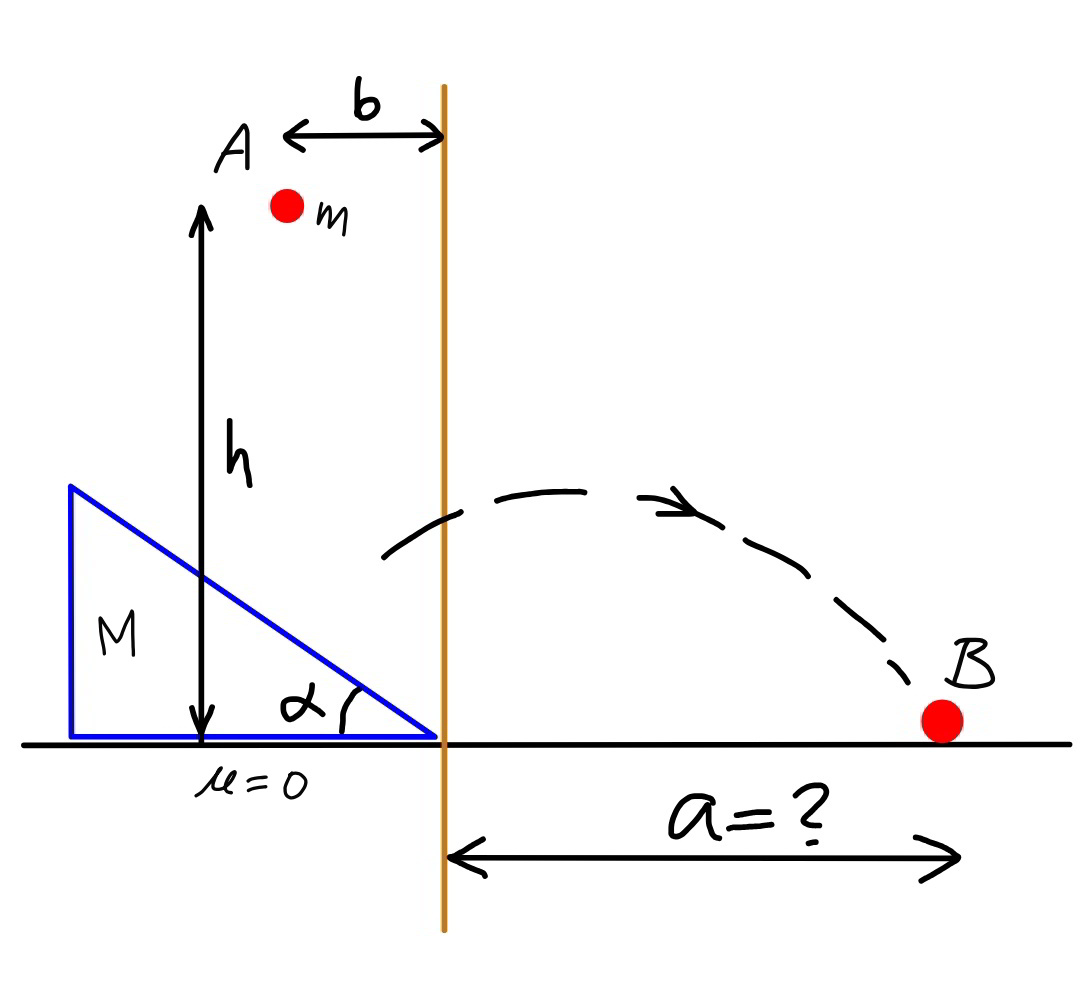
Question Number 91439 Answers: 1 Comments: 2
Question Number 91671 Answers: 0 Comments: 2
$${show}\:{that} \\ $$$$\sqrt[{{ln}\left({x}\right)}]{{x}}={e} \\ $$
Question Number 91419 Answers: 0 Comments: 1
Question Number 91417 Answers: 0 Comments: 1
Question Number 91422 Answers: 0 Comments: 0
Question Number 91399 Answers: 2 Comments: 13

Question Number 91395 Answers: 0 Comments: 0
Question Number 91390 Answers: 1 Comments: 3
Question Number 91421 Answers: 2 Comments: 2
Question Number 91384 Answers: 0 Comments: 4
Question Number 91378 Answers: 1 Comments: 6

Question Number 91377 Answers: 0 Comments: 1

Question Number 91375 Answers: 0 Comments: 3
Question Number 91374 Answers: 0 Comments: 0
Question Number 91371 Answers: 0 Comments: 1
Question Number 91362 Answers: 1 Comments: 3

Question Number 91354 Answers: 0 Comments: 3
Question Number 91353 Answers: 1 Comments: 0
$$\frac{{dy}}{{dx}}\:=\:{e}^{\mathrm{3}{x}−{y}^{\mathrm{2}} } \: \\ $$
Question Number 91336 Answers: 0 Comments: 9
Question Number 91329 Answers: 0 Comments: 3
Question Number 91328 Answers: 0 Comments: 9
Question Number 91326 Answers: 2 Comments: 1

Pg 1225 Pg 1226 Pg 1227 Pg 1228 Pg 1229 Pg 1230 Pg 1231 Pg 1232 Pg 1233 Pg 1234
