
AllQuestion and Answers: Page 1229
Question Number 91508 Answers: 0 Comments: 1
Question Number 91507 Answers: 0 Comments: 1
Question Number 91500 Answers: 0 Comments: 1
Question Number 91497 Answers: 0 Comments: 1
$${v}=\pi\int_{\mathrm{0}} ^{\mathrm{2}} {x}^{\mathrm{2}} {dx} \\ $$
Question Number 91496 Answers: 0 Comments: 1
Question Number 91494 Answers: 1 Comments: 0
Question Number 91493 Answers: 1 Comments: 1
Question Number 91484 Answers: 0 Comments: 2

Question Number 91491 Answers: 1 Comments: 0
Question Number 91479 Answers: 1 Comments: 4
Question Number 91476 Answers: 0 Comments: 2
Question Number 91474 Answers: 0 Comments: 0
Question Number 91473 Answers: 1 Comments: 0

Question Number 91471 Answers: 1 Comments: 0
Question Number 91470 Answers: 0 Comments: 2
Question Number 91469 Answers: 0 Comments: 3
$$−\mathrm{5}<\frac{\mathrm{4}−\mathrm{3x}}{\mathrm{2}}<\mathrm{l} \\ $$
Question Number 91468 Answers: 0 Comments: 0

Question Number 91464 Answers: 0 Comments: 12
Question Number 91465 Answers: 1 Comments: 1

Question Number 91460 Answers: 1 Comments: 0
Question Number 91452 Answers: 2 Comments: 1
Question Number 91448 Answers: 0 Comments: 3
Question Number 91446 Answers: 0 Comments: 1
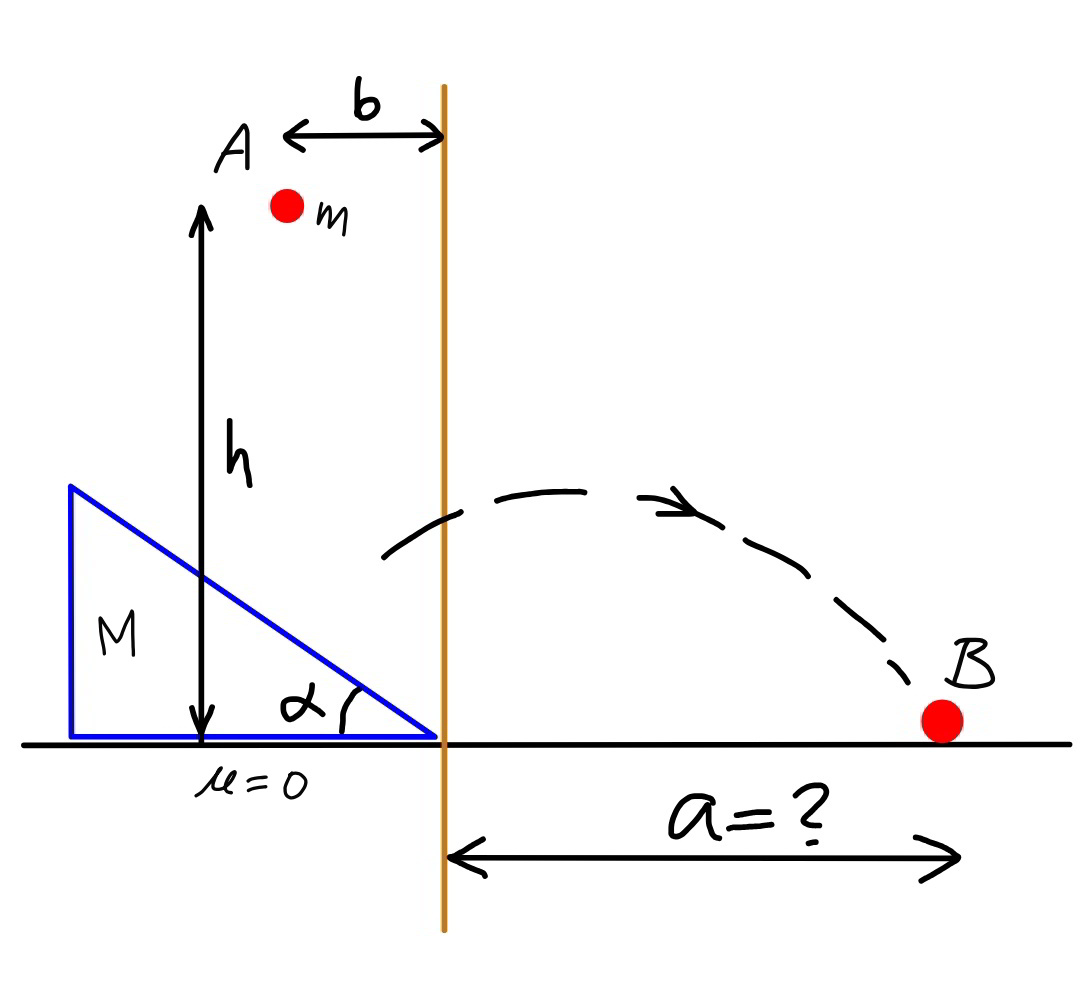
Question Number 91439 Answers: 1 Comments: 2
Question Number 91671 Answers: 0 Comments: 2
$${show}\:{that} \\ $$$$\sqrt[{{ln}\left({x}\right)}]{{x}}={e} \\ $$
Question Number 91419 Answers: 0 Comments: 1
Pg 1224 Pg 1225 Pg 1226 Pg 1227 Pg 1228 Pg 1229 Pg 1230 Pg 1231 Pg 1232 Pg 1233
