
AllQuestion and Answers: Page 1227
Question Number 91840 Answers: 0 Comments: 0
Question Number 91837 Answers: 0 Comments: 5
Question Number 91836 Answers: 1 Comments: 1
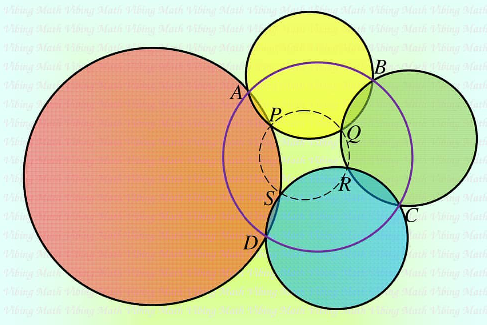
Question Number 91825 Answers: 2 Comments: 3
Question Number 92524 Answers: 0 Comments: 0
Question Number 91813 Answers: 0 Comments: 3
Question Number 91812 Answers: 0 Comments: 2
Question Number 92521 Answers: 0 Comments: 10

Question Number 92518 Answers: 1 Comments: 0

Question Number 92510 Answers: 1 Comments: 1
Question Number 92511 Answers: 0 Comments: 2
Question Number 91796 Answers: 1 Comments: 1
Question Number 91786 Answers: 0 Comments: 7
Question Number 91785 Answers: 0 Comments: 5
Question Number 91783 Answers: 0 Comments: 1
Question Number 91774 Answers: 0 Comments: 0
Question Number 91771 Answers: 0 Comments: 1
Question Number 91770 Answers: 1 Comments: 2
Question Number 91763 Answers: 0 Comments: 0

Question Number 91760 Answers: 1 Comments: 3
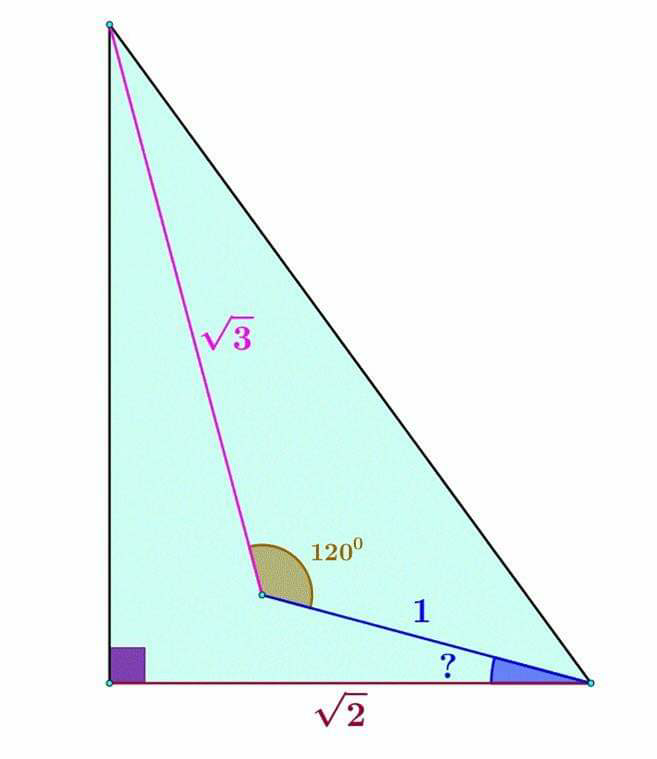
Question Number 91753 Answers: 1 Comments: 3
$$\int\mathrm{3}\:\left(\mathrm{ln}\:{x}\right)^{\mathrm{2}} \:{dx}\:=\:?? \\ $$
Question Number 91750 Answers: 0 Comments: 2
Question Number 91735 Answers: 0 Comments: 1
Question Number 91732 Answers: 0 Comments: 2
Question Number 91744 Answers: 1 Comments: 1

Question Number 91715 Answers: 0 Comments: 4
Pg 1222 Pg 1223 Pg 1224 Pg 1225 Pg 1226 Pg 1227 Pg 1228 Pg 1229 Pg 1230 Pg 1231
