Question and Answers Forum

AllQuestion and Answers: Page 1224

Question Number 82596 Answers: 0 Comments: 4

Question Number 82593 Answers: 0 Comments: 3

Question Number 82591 Answers: 0 Comments: 12

A closed surface is defined in spherical coordinates by 3<r<5 , 0.1π<θ<0.3π, 1.2π<φ<1.6π. Find the total surface area.

$${A}\:{closed}\:{surface}\:{is}\:{defined}\:{in}\:{spherical} \\ $$$${coordinates}\:{by}\:\mathrm{3}<{r}<\mathrm{5}\:,\:\mathrm{0}.\mathrm{1}\pi<\theta<\mathrm{0}.\mathrm{3}\pi, \\ $$$$\mathrm{1}.\mathrm{2}\pi<\phi<\mathrm{1}.\mathrm{6}\pi.\:\boldsymbol{{F}}{ind}\:{the}\:{total}\:{surface} \\ $$$${area}. \\ $$

Question Number 82589 Answers: 0 Comments: 1

Question Number 82583 Answers: 0 Comments: 0

Question Number 82582 Answers: 0 Comments: 2

solve x^3 −3x+1=0

$${solve} \\ $$$${x}^{\mathrm{3}} −\mathrm{3}{x}+\mathrm{1}=\mathrm{0} \\ $$

Question Number 82579 Answers: 1 Comments: 0

if y=cos(ln(x))+sin(ln(x)) show that y′′+y^′ +y=0

$${if}\:{y}={cos}\left({ln}\left({x}\right)\right)+{sin}\left({ln}\left({x}\right)\right) \\ $$$${show}\:{that} \\ $$$${y}''+{y}^{'} +{y}=\mathrm{0} \\ $$

Question Number 82577 Answers: 0 Comments: 3

∫((2−(√(x+3)))/(2+(√(x−3)))) dx

$$\int\frac{\mathrm{2}−\sqrt{{x}+\mathrm{3}}}{\mathrm{2}+\sqrt{{x}−\mathrm{3}}}\:{dx} \\ $$

Question Number 82574 Answers: 0 Comments: 0

Question Number 82573 Answers: 0 Comments: 4

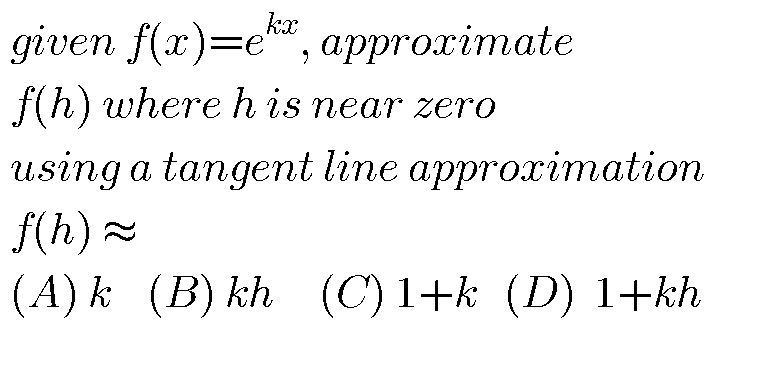
Question Number 82572 Answers: 0 Comments: 1

Question Number 82571 Answers: 0 Comments: 0

Question Number 82570 Answers: 0 Comments: 1

if f(x) = f(x+1) − x find f(x)

$${if}\:{f}\left({x}\right)\:=\:{f}\left({x}+\mathrm{1}\right)\:−\:{x}\: \\ $$$${find}\:{f}\left({x}\right)\: \\ $$

Question Number 82568 Answers: 1 Comments: 0

∫ (1/(tan x+cot x+sec x+cosec x)) dx ?

$$\int\:\frac{\mathrm{1}}{\mathrm{tan}\:{x}+\mathrm{cot}\:{x}+\mathrm{sec}\:{x}+\mathrm{cosec}\:{x}}\:{dx}\:? \\ $$

Question Number 82566 Answers: 2 Comments: 1

∫ (√((x+1)/x)) dx = ?

$$\int\:\sqrt{\frac{{x}+\mathrm{1}}{{x}}}\:{dx}\:=\:? \\ $$

Question Number 82564 Answers: 0 Comments: 0

Question Number 82562 Answers: 0 Comments: 0

Question Number 82561 Answers: 1 Comments: 0

a polynomial gives a remainder of −x−1 when divided by x^(2.) and a remainder of −1 when divided by x−1 . what is the remainder when the polynomial divided by x^2 (x−1) ?

$${a}\:{polynomial}\:{gives}\:{a}\:{remainder} \\ $$$${of}\:−{x}−\mathrm{1}\:{when}\:{divided}\:{by}\:{x}^{\mathrm{2}.} \\ $$$${and}\:\:{a}\:{remainder}\:{of}\:−\mathrm{1}\:{when}\: \\ $$$${divided}\:{by}\:{x}−\mathrm{1}\:.\:{what}\:{is}\:{the}\: \\ $$$${remainder}\:{when}\:{the}\:{polynomial} \\ $$$${divided}\:{by}\:{x}^{\mathrm{2}} \left({x}−\mathrm{1}\right)\:? \\ $$

Question Number 82560 Answers: 1 Comments: 0

Use gamma function to prove (i) ∫_0 ^(π/4) sin^4 x 2x dx = ((3𝛑−4)/(192)). (ii) ∫_0 ^(π/6) cos^4 3𝛉 sin^2 6θ = ((5π)/(192)).

$$\:\boldsymbol{\mathrm{U}}\mathrm{se}\:\mathrm{gamma}\:\mathrm{function}\:\mathrm{to}\:\mathrm{prove} \\ $$$$\:\:\left(\mathrm{i}\right)\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{4}}} \boldsymbol{\mathrm{sin}}^{\mathrm{4}} \boldsymbol{\mathrm{x}}\:\mathrm{2}\boldsymbol{\mathrm{x}}\:\boldsymbol{\mathrm{dx}}\:=\:\frac{\mathrm{3}\boldsymbol{\pi}−\mathrm{4}}{\mathrm{192}}. \\ $$$$\:\:\left(\mathrm{ii}\right)\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{6}}} \:\boldsymbol{\mathrm{cos}}^{\mathrm{4}} \mathrm{3}\boldsymbol{\theta}\:\mathrm{sin}^{\mathrm{2}} \mathrm{6}\theta\:=\:\frac{\mathrm{5}\pi}{\mathrm{192}}. \\ $$

Question Number 82546 Answers: 1 Comments: 1

show that if f(x)=a^x show that f ′(x)=a^x ln(a) by using lim_(h→0) ((f(x+h)−f(x))/h)

$${show}\:{that}\: \\ $$$${if}\:{f}\left({x}\right)={a}^{{x}} \\ $$$$ \\ $$$${show}\:{that}\:{f}\:'\left({x}\right)={a}^{{x}} \:{ln}\left({a}\right) \\ $$$$ \\ $$$${by}\:{using}\:\underset{{h}\rightarrow\mathrm{0}} {{lim}}\frac{{f}\left({x}+{h}\right)−{f}\left({x}\right)}{{h}} \\ $$

Question Number 82541 Answers: 0 Comments: 2

Lim_(x→∞) (√(4x^2 −8x+3)) −2x−4

$$\:\underset{\mathrm{x}\rightarrow\infty} {\mathrm{Lim}}\:\sqrt{\mathrm{4x}^{\mathrm{2}} −\mathrm{8x}+\mathrm{3}}\:−\mathrm{2x}−\mathrm{4} \\ $$

Question Number 82540 Answers: 1 Comments: 0

The arithmetic mean between two numbers is A, and S is the sum of n arithmetic means between these numbers, then

$$\mathrm{The}\:\mathrm{arithmetic}\:\mathrm{mean}\:\mathrm{between}\:\mathrm{two}\:\mathrm{numbers} \\ $$$$\mathrm{is}\:{A},\:\mathrm{and}\:{S}\:\mathrm{is}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:{n}\:\mathrm{arithmetic}\:\mathrm{means} \\ $$$$\mathrm{between}\:\mathrm{these}\:\mathrm{numbers},\:\mathrm{then} \\ $$

Question Number 82531 Answers: 1 Comments: 1

Question Number 82528 Answers: 0 Comments: 1

Question Number 82521 Answers: 0 Comments: 7

given (dy/dx) = xy^3 Determine the concavity of all solution curves for the given differential equation in quadrant iv.

$${given}\:\frac{{dy}}{{dx}}\:=\:{xy}^{\mathrm{3}} \\ $$$${Determine}\:{the}\:{concavity}\:{of}\:{all} \\ $$$${solution}\:{curves}\:{for}\:{the}\:{given}\: \\ $$$${differential}\:{equation}\:{in}\: \\ $$$${quadrant}\:{iv}.\: \\ $$

Question Number 82520 Answers: 1 Comments: 1

Pg 1219 Pg 1220 Pg 1221 Pg 1222 Pg 1223 Pg 1224 Pg 1225 Pg 1226 Pg 1227 Pg 1228

Contact: info@tinkutara.com