Question and Answers Forum

AllQuestion and Answers: Page 1223

Question Number 92301 Answers: 1 Comments: 2

given eq of line (1) [ x,y ] = [3,−2] + t [4,−5] (2) [x,y] = [1,1] + s [ 7,k ] find t and s if (1) ∥ (2) if (1) ⊥ (2)

$$\mathrm{given}\:\mathrm{eq}\:\mathrm{of}\:\mathrm{line}\: \\ $$$$\left(\mathrm{1}\right)\:\left[\:\mathrm{x},\mathrm{y}\:\right]\:=\:\left[\mathrm{3},−\mathrm{2}\right]\:+\:\mathrm{t}\:\left[\mathrm{4},−\mathrm{5}\right]\: \\ $$$$\left(\mathrm{2}\right)\:\left[\mathrm{x},\mathrm{y}\right]\:=\:\left[\mathrm{1},\mathrm{1}\right]\:+\:\mathrm{s}\:\left[\:\mathrm{7},\mathrm{k}\:\right]\: \\ $$$$\mathrm{find}\:\mathrm{t}\:\mathrm{and}\:\mathrm{s}\:\mathrm{if}\:\left(\mathrm{1}\right)\:\parallel\:\left(\mathrm{2}\right) \\ $$$$\mathrm{if}\:\left(\mathrm{1}\right)\:\bot\:\left(\mathrm{2}\right) \\ $$

Question Number 92291 Answers: 0 Comments: 1

lim_(x→1^− ) (1−x)^(ln x) =?

$$ \\ $$$$\underset{{x}\rightarrow\mathrm{1}^{−} } {\mathrm{lim}}\:\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{ln}\:\mathrm{x}} \:=?\: \\ $$

Question Number 92289 Answers: 0 Comments: 1

lim_(x→∞) ln((((3+e)^x )/(2x))) ?

$$ \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\mathrm{ln}\left(\frac{\left(\mathrm{3}+\mathrm{e}\right)^{\mathrm{x}} }{\mathrm{2x}}\right)\:? \\ $$

Question Number 92283 Answers: 0 Comments: 3

9^x +3^x = 25^x −5^x find (5^x /(3^x +1)) ?

$$\mathrm{9}^{\mathrm{x}} +\mathrm{3}^{\mathrm{x}} \:=\:\mathrm{25}^{\mathrm{x}} −\mathrm{5}^{\mathrm{x}} \: \\ $$$$\mathrm{find}\:\frac{\mathrm{5}^{\mathrm{x}} }{\mathrm{3}^{\mathrm{x}} +\mathrm{1}}\:? \\ $$

Question Number 92279 Answers: 0 Comments: 2

7sin(θ)+2cos^2 (θ)=5 0≤θ≤2π

$$\mathrm{7}{sin}\left(\theta\right)+\mathrm{2}{cos}^{\mathrm{2}} \left(\theta\right)=\mathrm{5} \\ $$$$ \\ $$$$\mathrm{0}\leqslant\theta\leqslant\mathrm{2}\pi \\ $$

Question Number 92277 Answers: 1 Comments: 4

find a,b,c,d if f(x)=ax^3 +bx^2 +cx+d (3,3)is maximum value (5,1) is minimum value (4,2) is inflection point

$${find}\:{a},{b},{c},{d}\:\: \\ $$$${if}\:\:\:\:{f}\left({x}\right)={ax}^{\mathrm{3}} +{bx}^{\mathrm{2}} +{cx}+{d} \\ $$$$\left(\mathrm{3},\mathrm{3}\right){is}\:{maximum}\:{value} \\ $$$$\left(\mathrm{5},\mathrm{1}\right)\:{is}\:{minimum}\:{value} \\ $$$$\left(\mathrm{4},\mathrm{2}\right)\:{is}\:{inflection}\:{point} \\ $$

Question Number 92275 Answers: 1 Comments: 0

Make R the subject of: P= ((RE^2 )/((R+b)^2 ))

$$\mathrm{Make}\:\mathrm{R}\:\mathrm{the}\:\mathrm{subject}\:\mathrm{of}: \\ $$$$\:\:\mathrm{P}=\:\frac{\mathrm{RE}^{\mathrm{2}} }{\left(\mathrm{R}+\mathrm{b}\right)^{\mathrm{2}} } \\ $$

Question Number 92269 Answers: 1 Comments: 0

Question Number 92267 Answers: 0 Comments: 1

give ∫_0 ^1 ((ln(1−x))/(1+x))dx at form of serie

$${give}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}−{x}\right)}{\mathrm{1}+{x}}{dx}\:{at}\:{form}\:{of}\:{serie} \\ $$

Question Number 92256 Answers: 1 Comments: 5

lim_(x→0) (1/x)−(1/(ln(1+x))) ?

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}}{\mathrm{x}}−\frac{\mathrm{1}}{\mathrm{ln}\left(\mathrm{1}+\mathrm{x}\right)}\:? \\ $$

Question Number 92255 Answers: 2 Comments: 0

7x = 3 (mod 18 )

$$\mathrm{7x}\:=\:\mathrm{3}\:\left(\mathrm{mod}\:\mathrm{18}\:\right)\: \\ $$

Question Number 92252 Answers: 1 Comments: 0

{ ((x(√y) +y(√x) = 6)),((x+y = 5 )) :} find x^3 + (1/y) =

$$\begin{cases}{\mathrm{x}\sqrt{\mathrm{y}}\:+\mathrm{y}\sqrt{\mathrm{x}}\:=\:\mathrm{6}}\\{\mathrm{x}+\mathrm{y}\:=\:\mathrm{5}\:}\end{cases} \\ $$$$\mathrm{find}\:\mathrm{x}^{\mathrm{3}} +\:\frac{\mathrm{1}}{\mathrm{y}}\:=\: \\ $$

Question Number 92248 Answers: 0 Comments: 4

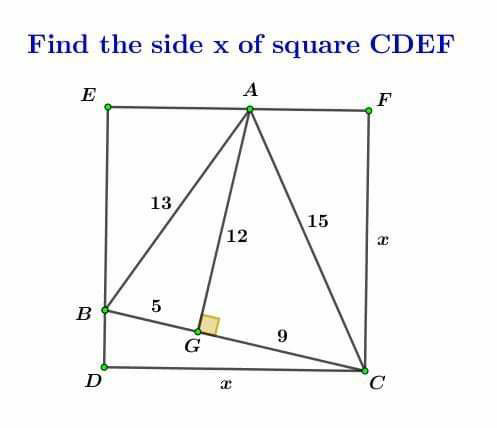
Question Number 92247 Answers: 0 Comments: 1

How to convert the non−linear equations to linear form? y=(x/(c+mx)) y=ce^(mx)

$$\mathrm{How}\:\mathrm{to}\:\mathrm{convert}\:\mathrm{the}\:\mathrm{non}−\mathrm{linear}\:\mathrm{equation}{s} \\ $$$$\mathrm{to}\:\mathrm{linear}\:\mathrm{form}? \\ $$$$ \\ $$$${y}=\frac{{x}}{{c}+{mx}} \\ $$$$ \\ $$$${y}={ce}^{{mx}} \\ $$

Question Number 92242 Answers: 0 Comments: 2

((8^x +27^x )/(12^x +18^x )) = (7/6) x = ?

$$\frac{\mathrm{8}^{{x}} +\mathrm{27}^{{x}} }{\mathrm{12}^{{x}} +\mathrm{18}^{{x}} }\:=\:\frac{\mathrm{7}}{\mathrm{6}}\: \\ $$$${x}\:=\:? \\ $$

Question Number 92239 Answers: 0 Comments: 5

lim_(x→0) ((1−∣cos 7x∣)/(1−∣tan 5x∣)) =

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}−\mid\mathrm{cos}\:\mathrm{7x}\mid}{\mathrm{1}−\mid\mathrm{tan}\:\mathrm{5x}\mid}\:=\: \\ $$

Question Number 92235 Answers: 0 Comments: 0

let 0<p<1 and x>0 prove that x^2 ≤ (1−p)( ^((1−p)) (√x) ) +p (^p (√x))

$${let}\:\:\mathrm{0}<{p}<\mathrm{1}\:\:{and}\:\:{x}>\mathrm{0} \\ $$$${prove}\:{that}\:\:\:\:{x}^{\mathrm{2}} \leqslant\:\left(\mathrm{1}−{p}\right)\left(\:\:\:^{\left(\mathrm{1}−{p}\right)} \sqrt{{x}}\:\right)\:+{p}\:\left(\:^{{p}} \sqrt{{x}}\right) \\ $$$$ \\ $$$$ \\ $$

Question Number 92231 Answers: 0 Comments: 0

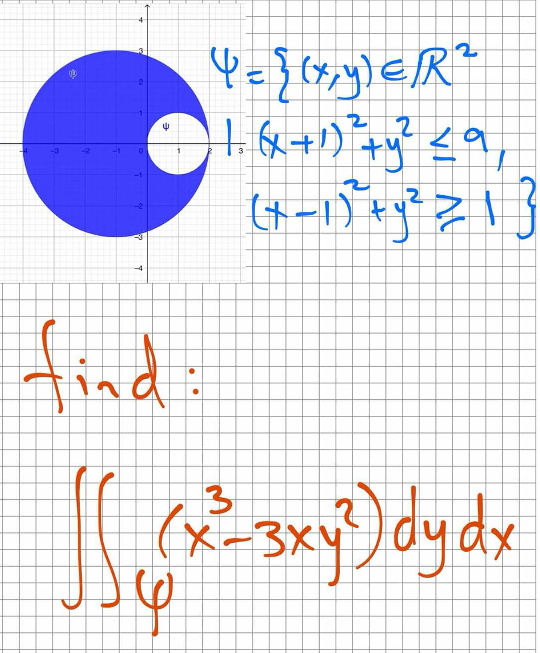
Question Number 92232 Answers: 0 Comments: 1

Question Number 92228 Answers: 0 Comments: 0

Are there infinite prime number p such as p!≡1[p+2]

$$\:{Are}\:{there}\:{infinite}\:{prime}\:{number}\:{p}\:{such}\:{as} \\ $$$$\:\:\:{p}!\equiv\mathrm{1}\left[{p}+\mathrm{2}\right]\: \\ $$

Question Number 92226 Answers: 0 Comments: 6

Question Number 92225 Answers: 1 Comments: 0

if tanh(x)=((72)/(161))(√5) prove that sinh(x)∈Q Q={rational numbdrs}

$${if}\:\:\:{tanh}\left({x}\right)=\frac{\mathrm{72}}{\mathrm{161}}\sqrt{\mathrm{5}} \\ $$$${prove}\:{that}\:{sinh}\left({x}\right)\in{Q}\: \\ $$$$ \\ $$$$ \\ $$$${Q}=\left\{{rational}\:{numbdrs}\right\} \\ $$$$ \\ $$

Question Number 92219 Answers: 0 Comments: 5

Question Number 92214 Answers: 0 Comments: 1

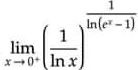
Question Number 92211 Answers: 1 Comments: 1

4x = 2 (mod 3 )

$$\mathrm{4x}\:=\:\mathrm{2}\:\left(\mathrm{mod}\:\mathrm{3}\:\right)\: \\ $$

Question Number 92197 Answers: 0 Comments: 1

Given L(n) = { ((0 , if n = 1)),((L ⌊(n/2)⌋ +1 , if n > 1)) :} find L(25)

$$\mathrm{Given}\:\mathrm{L}\left(\mathrm{n}\right)\:=\:\begin{cases}{\mathrm{0}\:,\:\mathrm{if}\:\mathrm{n}\:=\:\mathrm{1}}\\{\mathrm{L}\:\lfloor\frac{\mathrm{n}}{\mathrm{2}}\rfloor\:+\mathrm{1}\:,\:\mathrm{if}\:\mathrm{n}\:>\:\mathrm{1}}\end{cases} \\ $$$$\mathrm{find}\:\mathrm{L}\left(\mathrm{25}\right)\: \\ $$

Pg 1218 Pg 1219 Pg 1220 Pg 1221 Pg 1222 Pg 1223 Pg 1224 Pg 1225 Pg 1226 Pg 1227

Contact: info@tinkutara.com