Question and Answers Forum

AllQuestion and Answers: Page 1222

Question Number 92390 Answers: 0 Comments: 2

What is the meaning of this symbol (ε) in limit please. or as used in convergent/divergent series

$$\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{meaning}\:\mathrm{of}\:\mathrm{this}\:\mathrm{symbol}\:\:\left(\varepsilon\right)\:\mathrm{in}\:\mathrm{limit}\:\mathrm{please}. \\ $$$$\mathrm{or}\:\mathrm{as}\:\mathrm{used}\:\mathrm{in}\:\mathrm{convergent}/\mathrm{divergent}\:\mathrm{series} \\ $$

Question Number 92366 Answers: 0 Comments: 3

Question Number 92356 Answers: 0 Comments: 7

Question Number 92379 Answers: 1 Comments: 5

f(x) and g(x) are functions with no constants. if f ′(x)=g ′(x) is that mean f(x)=g(x) ??

$${f}\left({x}\right)\:{and}\:{g}\left({x}\right)\:{are}\:{functions}\:{with}\:{no} \\ $$$${constants}. \\ $$$${if}\:{f}\:'\left({x}\right)={g}\:'\left({x}\right)\:{is}\:{that}\:{mean}\:{f}\left({x}\right)={g}\left({x}\right) \\ $$$$?? \\ $$

Question Number 92347 Answers: 1 Comments: 3

Question Number 92346 Answers: 3 Comments: 3

solve ((1+(√x)))^(1/3) +((1−(√x)))^(1/3) =(5)^(1/3)

$${solve} \\ $$$$\sqrt[{\mathrm{3}}]{\mathrm{1}+\sqrt{{x}}}+\sqrt[{\mathrm{3}}]{\mathrm{1}−\sqrt{{x}}}=\sqrt[{\mathrm{3}}]{\mathrm{5}} \\ $$

Question Number 92344 Answers: 0 Comments: 3

∫_0 ^1 (dx/((√(1+3x))−(√(1−3x))))

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{\mathrm{dx}}{\sqrt{\mathrm{1}+\mathrm{3x}}−\sqrt{\mathrm{1}−\mathrm{3x}}} \\ $$

Question Number 92340 Answers: 0 Comments: 2

Π_(i = 1) ^∞ ((5^(((1/2))^i ) +3^(((1/2))^i ) )/2) =

$$\underset{\mathrm{i}\:=\:\mathrm{1}} {\overset{\infty} {\prod}}\:\frac{\mathrm{5}^{\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{i}} } +\mathrm{3}^{\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{i}} } }{\mathrm{2}}\:=\: \\ $$

Question Number 92337 Answers: 0 Comments: 0

Question Number 92335 Answers: 0 Comments: 0

(√({x})) = 1+ ln(x)

$$\sqrt{\left\{\mathrm{x}\right\}}\:=\:\mathrm{1}+\:\mathrm{ln}\left(\mathrm{x}\right)\: \\ $$

Question Number 92334 Answers: 0 Comments: 0

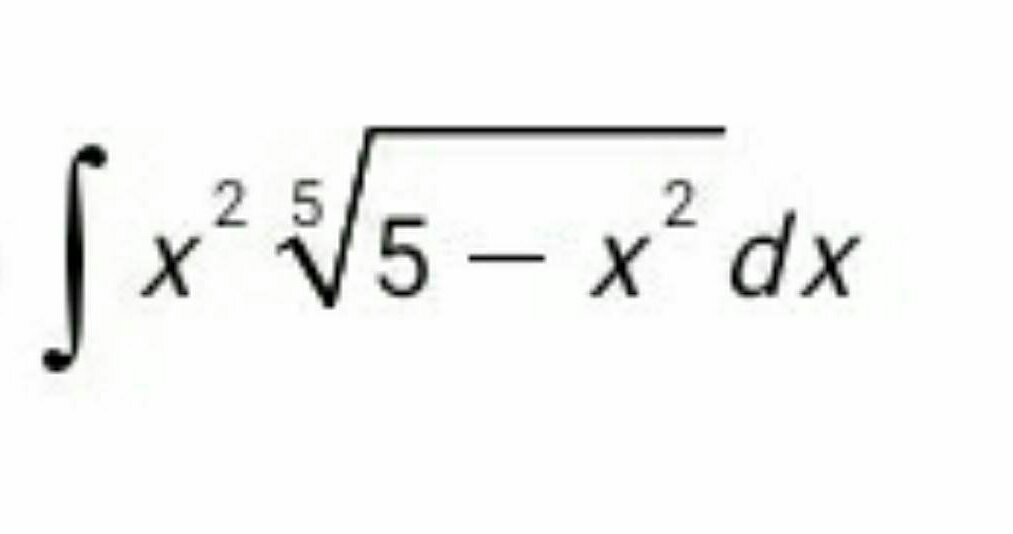
Question Number 92324 Answers: 1 Comments: 0

Find the value of x for which Σ_(n = 0) ^(n = ∞) 16((3/4)x + 1)^n (a) Is convergent (b) Is equal to 10(2/3)

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\:\mathrm{x}\:\:\mathrm{for}\:\mathrm{which}\:\:\:\:\underset{\mathrm{n}\:\:=\:\:\mathrm{0}} {\overset{\mathrm{n}\:\:=\:\:\infty} {\sum}}\:\mathrm{16}\left(\frac{\mathrm{3}}{\mathrm{4}}\mathrm{x}\:\:+\:\:\mathrm{1}\right)^{\mathrm{n}} \\ $$$$\left(\mathrm{a}\right)\:\:\:\mathrm{Is}\:\mathrm{convergent} \\ $$$$\left(\mathrm{b}\right)\:\:\:\mathrm{Is}\:\mathrm{equal}\:\mathrm{to}\:\:\mathrm{10}\frac{\mathrm{2}}{\mathrm{3}} \\ $$

Question Number 92323 Answers: 2 Comments: 3

Question Number 92319 Answers: 0 Comments: 3

log _9 (x+(7/2)).log _(3/4) (x^2 ) ≥ log _(3/4) (x+(7/2))

$$\mathrm{log}\:_{\mathrm{9}} \left(\mathrm{x}+\frac{\mathrm{7}}{\mathrm{2}}\right).\mathrm{log}\:_{\mathrm{3}/\mathrm{4}} \left(\mathrm{x}^{\mathrm{2}} \right)\:\geqslant\: \\ $$$$\mathrm{log}\:_{\mathrm{3}/\mathrm{4}} \left(\mathrm{x}+\frac{\mathrm{7}}{\mathrm{2}}\right)\: \\ $$

Question Number 92301 Answers: 1 Comments: 2

given eq of line (1) [ x,y ] = [3,−2] + t [4,−5] (2) [x,y] = [1,1] + s [ 7,k ] find t and s if (1) ∥ (2) if (1) ⊥ (2)

$$\mathrm{given}\:\mathrm{eq}\:\mathrm{of}\:\mathrm{line}\: \\ $$$$\left(\mathrm{1}\right)\:\left[\:\mathrm{x},\mathrm{y}\:\right]\:=\:\left[\mathrm{3},−\mathrm{2}\right]\:+\:\mathrm{t}\:\left[\mathrm{4},−\mathrm{5}\right]\: \\ $$$$\left(\mathrm{2}\right)\:\left[\mathrm{x},\mathrm{y}\right]\:=\:\left[\mathrm{1},\mathrm{1}\right]\:+\:\mathrm{s}\:\left[\:\mathrm{7},\mathrm{k}\:\right]\: \\ $$$$\mathrm{find}\:\mathrm{t}\:\mathrm{and}\:\mathrm{s}\:\mathrm{if}\:\left(\mathrm{1}\right)\:\parallel\:\left(\mathrm{2}\right) \\ $$$$\mathrm{if}\:\left(\mathrm{1}\right)\:\bot\:\left(\mathrm{2}\right) \\ $$

Question Number 92291 Answers: 0 Comments: 1

lim_(x→1^− ) (1−x)^(ln x) =?

$$ \\ $$$$\underset{{x}\rightarrow\mathrm{1}^{−} } {\mathrm{lim}}\:\left(\mathrm{1}−\mathrm{x}\right)^{\mathrm{ln}\:\mathrm{x}} \:=?\: \\ $$

Question Number 92289 Answers: 0 Comments: 1

lim_(x→∞) ln((((3+e)^x )/(2x))) ?

$$ \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\:\mathrm{ln}\left(\frac{\left(\mathrm{3}+\mathrm{e}\right)^{\mathrm{x}} }{\mathrm{2x}}\right)\:? \\ $$

Question Number 92283 Answers: 0 Comments: 3

9^x +3^x = 25^x −5^x find (5^x /(3^x +1)) ?

$$\mathrm{9}^{\mathrm{x}} +\mathrm{3}^{\mathrm{x}} \:=\:\mathrm{25}^{\mathrm{x}} −\mathrm{5}^{\mathrm{x}} \: \\ $$$$\mathrm{find}\:\frac{\mathrm{5}^{\mathrm{x}} }{\mathrm{3}^{\mathrm{x}} +\mathrm{1}}\:? \\ $$

Question Number 92279 Answers: 0 Comments: 2

7sin(θ)+2cos^2 (θ)=5 0≤θ≤2π

$$\mathrm{7}{sin}\left(\theta\right)+\mathrm{2}{cos}^{\mathrm{2}} \left(\theta\right)=\mathrm{5} \\ $$$$ \\ $$$$\mathrm{0}\leqslant\theta\leqslant\mathrm{2}\pi \\ $$

Question Number 92277 Answers: 1 Comments: 4

find a,b,c,d if f(x)=ax^3 +bx^2 +cx+d (3,3)is maximum value (5,1) is minimum value (4,2) is inflection point

$${find}\:{a},{b},{c},{d}\:\: \\ $$$${if}\:\:\:\:{f}\left({x}\right)={ax}^{\mathrm{3}} +{bx}^{\mathrm{2}} +{cx}+{d} \\ $$$$\left(\mathrm{3},\mathrm{3}\right){is}\:{maximum}\:{value} \\ $$$$\left(\mathrm{5},\mathrm{1}\right)\:{is}\:{minimum}\:{value} \\ $$$$\left(\mathrm{4},\mathrm{2}\right)\:{is}\:{inflection}\:{point} \\ $$

Question Number 92275 Answers: 1 Comments: 0

Make R the subject of: P= ((RE^2 )/((R+b)^2 ))

$$\mathrm{Make}\:\mathrm{R}\:\mathrm{the}\:\mathrm{subject}\:\mathrm{of}: \\ $$$$\:\:\mathrm{P}=\:\frac{\mathrm{RE}^{\mathrm{2}} }{\left(\mathrm{R}+\mathrm{b}\right)^{\mathrm{2}} } \\ $$

Question Number 92269 Answers: 1 Comments: 0

Question Number 92267 Answers: 0 Comments: 1

give ∫_0 ^1 ((ln(1−x))/(1+x))dx at form of serie

$${give}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}−{x}\right)}{\mathrm{1}+{x}}{dx}\:{at}\:{form}\:{of}\:{serie} \\ $$

Question Number 92256 Answers: 1 Comments: 5

lim_(x→0) (1/x)−(1/(ln(1+x))) ?

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{1}}{\mathrm{x}}−\frac{\mathrm{1}}{\mathrm{ln}\left(\mathrm{1}+\mathrm{x}\right)}\:? \\ $$

Question Number 92255 Answers: 2 Comments: 0

7x = 3 (mod 18 )

$$\mathrm{7x}\:=\:\mathrm{3}\:\left(\mathrm{mod}\:\mathrm{18}\:\right)\: \\ $$

Question Number 92252 Answers: 1 Comments: 0

{ ((x(√y) +y(√x) = 6)),((x+y = 5 )) :} find x^3 + (1/y) =

$$\begin{cases}{\mathrm{x}\sqrt{\mathrm{y}}\:+\mathrm{y}\sqrt{\mathrm{x}}\:=\:\mathrm{6}}\\{\mathrm{x}+\mathrm{y}\:=\:\mathrm{5}\:}\end{cases} \\ $$$$\mathrm{find}\:\mathrm{x}^{\mathrm{3}} +\:\frac{\mathrm{1}}{\mathrm{y}}\:=\: \\ $$

Pg 1217 Pg 1218 Pg 1219 Pg 1220 Pg 1221 Pg 1222 Pg 1223 Pg 1224 Pg 1225 Pg 1226

Contact: info@tinkutara.com