Question and Answers Forum

AllQuestion and Answers: Page 1213

Question Number 88238 Answers: 1 Comments: 0

solve (3x^5 y^4 +4y)dx+(2x^6 y^3 +3x)dy=0

$${solve}\: \\ $$$$\left(\mathrm{3}{x}^{\mathrm{5}} {y}^{\mathrm{4}} +\mathrm{4}{y}\right){dx}+\left(\mathrm{2}{x}^{\mathrm{6}} {y}^{\mathrm{3}} +\mathrm{3}{x}\right){dy}=\mathrm{0} \\ $$

Question Number 88236 Answers: 1 Comments: 0

Evaluate ∫(((27)/(x^3 −6)))^(1/3) dx

$$\:\mathrm{Evaluate}\:\:\int\sqrt[{\mathrm{3}}]{\frac{\mathrm{27}}{{x}^{\mathrm{3}} −\mathrm{6}}}\:{dx}\: \\ $$

Question Number 88235 Answers: 0 Comments: 1

find a maclaurine series solution to the differential equation up to the term in x^4 . (dy/dx) − x = xy if y = 1 when x = 0.

$$\:\mathrm{find}\:\mathrm{a}\:\mathrm{maclaurine}\:\mathrm{series}\:\mathrm{solution}\:\mathrm{to}\:\mathrm{the}\:\mathrm{differential}\:\mathrm{equation} \\ $$$$\mathrm{up}\:\mathrm{to}\:\mathrm{the}\:\mathrm{term}\:\mathrm{in}\:{x}^{\mathrm{4}} . \\ $$$$\:\frac{{dy}}{{dx}}\:−\:{x}\:=\:{xy}\:\:\:\mathrm{if}\:\:{y}\:=\:\mathrm{1}\:\mathrm{when}\:{x}\:=\:\mathrm{0}. \\ $$

Question Number 88232 Answers: 0 Comments: 0

Question Number 88218 Answers: 1 Comments: 2

Question Number 88214 Answers: 2 Comments: 0

Question Number 88212 Answers: 0 Comments: 0

Σ[(e^s^e^s^ −x)^r ]^((s+5)((sin x)/(tan y))) =i s=5 r=2 x=90° y=45° i=?

$$\Sigma\left[\left(\mathrm{e}^{\mathrm{s}^{\mathrm{e}^{\mathrm{s}^{} } } } −\mathrm{x}\right)^{\mathrm{r}} \right]^{\left(\mathrm{s}+\mathrm{5}\right)\frac{\mathrm{sin}\:{x}}{\mathrm{tan}\:{y}}} \:={i} \\ $$$${s}=\mathrm{5} \\ $$$$\mathrm{r}=\mathrm{2} \\ $$$${x}=\mathrm{90}° \\ $$$$ \\ $$$$\mathrm{y}=\mathrm{45}° \\ $$$$\mathrm{i}=? \\ $$$$ \\ $$

Question Number 88211 Answers: 0 Comments: 0

Question Number 88210 Answers: 1 Comments: 0

If f(x)= { ((1−x, 0≤x≤1)),((0, 1≤x≤2 )),(((2−x)^2 , 2≤x≤3)) :} and φ(x)=∫_( 0) ^x f(t) dt. Then for any x ∈ [2, 3], φ(x) =

$$\mathrm{If}\:{f}\left({x}\right)=\begin{cases}{\mathrm{1}−{x},\:\:\:\:\:\:\:\:\:\mathrm{0}\leqslant{x}\leqslant\mathrm{1}}\\{\mathrm{0},\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{1}\leqslant{x}\leqslant\mathrm{2}\:\:\:\:}\\{\left(\mathrm{2}−{x}\right)^{\mathrm{2}} ,\:\:\:\mathrm{2}\leqslant{x}\leqslant\mathrm{3}}\end{cases}\:\mathrm{and}\: \\ $$$$\phi\left({x}\right)=\underset{\:\mathrm{0}} {\overset{\mathrm{x}} {\int}}\:{f}\left({t}\right)\:{dt}.\:\mathrm{Then}\:\mathrm{for}\:\mathrm{any}\:{x}\:\in\:\left[\mathrm{2},\:\mathrm{3}\right],\: \\ $$$$\phi\left({x}\right)\:= \\ $$

Question Number 88207 Answers: 1 Comments: 1

what is the biggest prime p verifying: p=Σ_(k=1) ^n [(√k)] where n∈N and [x] is floor(x)

$${what}\:{is}\:{the}\:{biggest}\:{prime}\:{p}\:{verifying}: \\ $$$${p}=\underset{{k}=\mathrm{1}} {\overset{{n}} {\sum}}\left[\sqrt{{k}}\right] \\ $$$${where}\:{n}\in\mathbb{N}\:\mathrm{and}\:\:\left[{x}\right]\:{is}\:{floor}\left({x}\right) \\ $$

Question Number 88206 Answers: 1 Comments: 0

∫ ((x+x^3 )/(1+x^4 )) dx

$$\int\:\:\frac{\mathrm{x}+\mathrm{x}^{\mathrm{3}} }{\mathrm{1}+\mathrm{x}^{\mathrm{4}} }\:\mathrm{dx}\: \\ $$

Question Number 88204 Answers: 0 Comments: 0

find radi of circle(s) that tangents to corves : { ((y=x±(1/y^2 ))),((x=y±(1/x^2 ))) :}

Question Number 88203 Answers: 0 Comments: 0

y=ax^(−3) , meets: y=e^x and y=−e^(−x) at: A and B,such that: AB is minimum. find: possible value(s) of: a and min of AB.

Question Number 88198 Answers: 1 Comments: 0

Factorize −r^2 +p^2 +q^2 −2pq .

$$\mathrm{Factorize}\:\:−{r}^{\mathrm{2}} +{p}^{\mathrm{2}} +{q}^{\mathrm{2}} −\mathrm{2}{pq}\:. \\ $$

Question Number 88197 Answers: 0 Comments: 0

prove that ∫_0 ^1 (√(((x^2 −2)/(x^2 −1)) ))dx=((π(√(2π)))/(Γ^2 ((1/4))))+((Γ^2 ((1/4)))/(4(√(2π))))

$${prove}\:{that}\: \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \sqrt{\frac{{x}^{\mathrm{2}} −\mathrm{2}}{{x}^{\mathrm{2}} −\mathrm{1}}\:}{dx}=\frac{\pi\sqrt{\mathrm{2}\pi}}{\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)}+\frac{\Gamma^{\mathrm{2}} \left(\frac{\mathrm{1}}{\mathrm{4}}\right)}{\mathrm{4}\sqrt{\mathrm{2}\pi}} \\ $$

Question Number 88196 Answers: 0 Comments: 0

Question Number 88194 Answers: 0 Comments: 4

find ∫(1/((√x)+(√(x+1))+(√(x+2)))) dx

$${find}\:\int\frac{\mathrm{1}}{\sqrt{{x}}+\sqrt{{x}+\mathrm{1}}+\sqrt{{x}+\mathrm{2}}}\:{dx} \\ $$$$ \\ $$

Question Number 88188 Answers: 1 Comments: 5

Question Number 88181 Answers: 0 Comments: 1

Question Number 88180 Answers: 0 Comments: 0

(5+4y)×dy=y^3 x

$$\left(\mathrm{5}+\mathrm{4}{y}\right)×{dy}={y}^{\mathrm{3}} {x}\: \\ $$

Question Number 88179 Answers: 1 Comments: 0

z=x^2 /y^3 dz=?

$${z}={x}^{\mathrm{2}} /{y}^{\mathrm{3}} \:\:\:\:\:\:\:\:\:\:{dz}=? \\ $$

Question Number 88178 Answers: 0 Comments: 0

$$ \\ $$

Question Number 88177 Answers: 1 Comments: 0

prove that ∫_0 ^(2020) (x−[x])(√(x−[x])) dx=808

$${prove}\:{that} \\ $$$$ \\ $$$$\int_{\mathrm{0}} ^{\mathrm{2020}} \left({x}−\left[{x}\right]\right)\sqrt{{x}−\left[{x}\right]}\:{dx}=\mathrm{808} \\ $$

Question Number 88170 Answers: 0 Comments: 2

Prove that ∫_0 ^1 tcos nπtdt=(((−1)^n −1)/(n^2 π^2 ))

$${Prove}\:{that}\: \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} {tcos}\:{n}\pi{tdt}=\frac{\left(−\mathrm{1}\right)^{{n}} −\mathrm{1}}{{n}^{\mathrm{2}} \pi^{\mathrm{2}} } \\ $$

Question Number 88169 Answers: 1 Comments: 2

find Laplace transform t^3 . cos 4t

$$\mathrm{find}\:\mathrm{Laplace}\:\mathrm{transform}\: \\ $$$$\mathrm{t}^{\mathrm{3}} .\:\mathrm{cos}\:\:\mathrm{4t} \\ $$

Question Number 88160 Answers: 2 Comments: 1

solve : x^2 = 3x + 6y ; xy = 5x + 4y

$${solve}\::\:{x}^{\mathrm{2}} \:=\:\mathrm{3}{x}\:+\:\mathrm{6}{y}\:;\:{xy}\:=\:\mathrm{5}{x}\:+\:\mathrm{4}{y} \\ $$

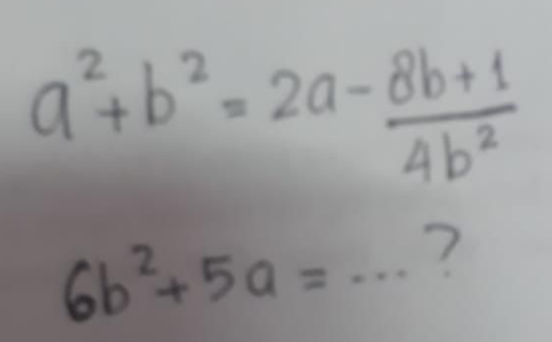
Pg 1208 Pg 1209 Pg 1210 Pg 1211 Pg 1212 Pg 1213 Pg 1214 Pg 1215 Pg 1216 Pg 1217

Contact: info@tinkutara.com