
AllQuestion and Answers: Page 1210
Question Number 93950 Answers: 2 Comments: 0
Question Number 93941 Answers: 0 Comments: 2
Question Number 93940 Answers: 0 Comments: 2
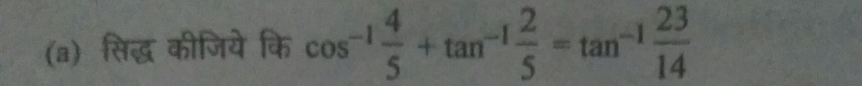
Question Number 93937 Answers: 0 Comments: 5
$$\int\frac{\mathrm{1}}{\sqrt{\mathrm{tan}\:{x}}}{dx}=? \\ $$
Question Number 93933 Answers: 0 Comments: 5
Question Number 93916 Answers: 0 Comments: 2

Question Number 93911 Answers: 0 Comments: 8
Question Number 93908 Answers: 0 Comments: 2
Question Number 93907 Answers: 2 Comments: 4
Question Number 93906 Answers: 0 Comments: 0
Question Number 93904 Answers: 1 Comments: 0
Question Number 93900 Answers: 0 Comments: 0
Question Number 93899 Answers: 0 Comments: 0
Question Number 93898 Answers: 1 Comments: 1
Question Number 93892 Answers: 0 Comments: 3
Question Number 93874 Answers: 1 Comments: 4
Question Number 93873 Answers: 1 Comments: 1
Question Number 93869 Answers: 0 Comments: 2
$$\int\:\frac{\mathrm{dx}}{\mathrm{1}−\mathrm{tan}\:^{\mathrm{2}} \mathrm{x}}\:? \\ $$
Question Number 93860 Answers: 0 Comments: 5
Question Number 93859 Answers: 1 Comments: 0
Question Number 93855 Answers: 1 Comments: 0
Question Number 93853 Answers: 1 Comments: 0
Question Number 93847 Answers: 0 Comments: 2
Question Number 93844 Answers: 1 Comments: 0
Question Number 93843 Answers: 1 Comments: 0
Question Number 93837 Answers: 0 Comments: 5

Pg 1205 Pg 1206 Pg 1207 Pg 1208 Pg 1209 Pg 1210 Pg 1211 Pg 1212 Pg 1213 Pg 1214
