Question and Answers Forum

AllQuestion and Answers: Page 1209

Question Number 94336 Answers: 2 Comments: 0

let f(x) =arctan(2x) e^(−3x) 1) determine f^((n)) (x) and f^((n)) (0) 2)developp f at integr serie

$$\mathrm{let}\:\mathrm{f}\left(\mathrm{x}\right)\:=\mathrm{arctan}\left(\mathrm{2x}\right)\:\mathrm{e}^{−\mathrm{3x}} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{determine}\:\mathrm{f}^{\left(\mathrm{n}\right)} \left(\mathrm{x}\right)\:\mathrm{and}\:\mathrm{f}^{\left(\mathrm{n}\right)} \left(\mathrm{0}\right) \\ $$$$\left.\mathrm{2}\right)\mathrm{developp}\:\mathrm{f}\:\mathrm{at}\:\mathrm{integr}\:\mathrm{serie} \\ $$

Question Number 94335 Answers: 2 Comments: 0

calculate Σ_(n=0) ^∞ n^((−1)^n ) x^n

$${calculate}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:{n}^{\left(−\mathrm{1}\right)^{{n}} } {x}^{{n}} \\ $$

Question Number 94334 Answers: 1 Comments: 0

let f(x) =((sinx)/x)if x≠0 and f(0)=1 1) findf^((n)) (x) and f^((n)) (0) 2)developp f at integr serie st x_0 =0 and x_0 =(π/2)

$${let}\:{f}\left({x}\right)\:=\frac{{sinx}}{{x}}{if}\:{x}\neq\mathrm{0}\:\:{and}\:{f}\left(\mathrm{0}\right)=\mathrm{1} \\ $$$$\left.\mathrm{1}\right)\:{findf}^{\left({n}\right)} \left({x}\right)\:{and}\:{f}^{\left({n}\right)} \left(\mathrm{0}\right) \\ $$$$\left.\mathrm{2}\right){developp}\:{f}\:{at}\:{integr}\:{serie}\:{st}\:{x}_{\mathrm{0}} =\mathrm{0}\:{and}\:{x}_{\mathrm{0}} =\frac{\pi}{\mathrm{2}} \\ $$

Question Number 94333 Answers: 1 Comments: 0

developp at integr serie ∫_(−∞) ^x (dt/(t^4 +t^2 +1))

$${developp}\:{at}\:{integr}\:{serie}\:\int_{−\infty} ^{{x}} \:\frac{{dt}}{{t}^{\mathrm{4}} \:+{t}^{\mathrm{2}} \:+\mathrm{1}} \\ $$

Question Number 94332 Answers: 0 Comments: 0

developp at integr serie f(x)=(arcsinx)^2

$${developp}\:{at}\:{integr}\:{serie}\:{f}\left({x}\right)=\left({arcsinx}\right)^{\mathrm{2}} \\ $$

Question Number 94331 Answers: 2 Comments: 0

1) calculate Σ_(n=0) ^∞ (x^n /(4n^2 −1)) with ∣x∣<1 2) find the value of Σ_(n=0) ^∞ (1/(4n^2 −1)) and Σ_(n=0) ^∞ (((−1)^n )/(4n^2 −1))

$$\left.\mathrm{1}\right)\:{calculate}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{{x}^{{n}} }{\mathrm{4}{n}^{\mathrm{2}} −\mathrm{1}}\:\:{with}\:\mid{x}\mid<\mathrm{1} \\ $$$$\left.\mathrm{2}\right)\:{find}\:{the}\:{value}\:{of}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\mathrm{1}}{\mathrm{4}{n}^{\mathrm{2}} −\mathrm{1}}\:{and}\:\sum_{{n}=\mathrm{0}} ^{\infty} \:\frac{\left(−\mathrm{1}\right)^{{n}} }{\mathrm{4}{n}^{\mathrm{2}} −\mathrm{1}} \\ $$$$ \\ $$

Question Number 94328 Answers: 2 Comments: 0

y′ + xy = x

$$\mathrm{y}'\:+\:\mathrm{xy}\:=\:\mathrm{x}\: \\ $$

Question Number 94324 Answers: 0 Comments: 0

Question Number 94319 Answers: 0 Comments: 4

Question Number 94318 Answers: 0 Comments: 2

Given f(xy) = f(x+y) and f(7) = 7. find f(1008)

$$\mathrm{Given}\:\mathrm{f}\left(\mathrm{xy}\right)\:=\:\mathrm{f}\left(\mathrm{x}+\mathrm{y}\right)\:\mathrm{and}\: \\ $$$$\mathrm{f}\left(\mathrm{7}\right)\:=\:\mathrm{7}.\:\mathrm{find}\:\mathrm{f}\left(\mathrm{1008}\right)\: \\ $$

Question Number 94341 Answers: 0 Comments: 0

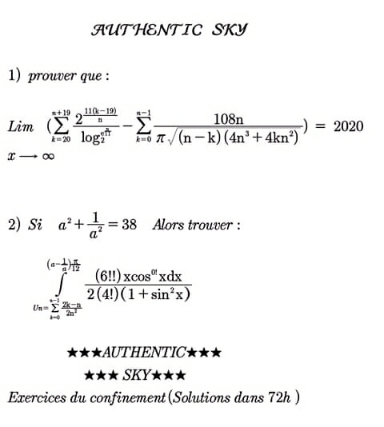
Question Number 94314 Answers: 0 Comments: 0

Question Number 94313 Answers: 0 Comments: 0

Question Number 94312 Answers: 0 Comments: 3

∫_0 ^a ((a^2 −x^2 )/((a^2 +x^2 )^2 )) dx ?

$$\underset{\mathrm{0}} {\overset{{a}} {\int}}\:\frac{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }{\left({a}^{\mathrm{2}} +{x}^{\mathrm{2}} \right)^{\mathrm{2}} }\:{dx}\:? \\ $$

Question Number 94311 Answers: 0 Comments: 0

explicit f(a) =∫_0 ^1 ((ln(1−ax^2 ))/x^2 )dx with 0<a<1

$${explicit}\:{f}\left({a}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}−{ax}^{\mathrm{2}} \right)}{{x}^{\mathrm{2}} }{dx}\:{with}\:\mathrm{0}<{a}<\mathrm{1} \\ $$

Question Number 94310 Answers: 2 Comments: 0

calculate ∫_0 ^1 ((ln(1−x^2 ))/x^2 )dx

$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{{x}^{\mathrm{2}} }{dx} \\ $$$$ \\ $$

Question Number 94309 Answers: 0 Comments: 0

Question Number 94298 Answers: 1 Comments: 0

Question Number 94297 Answers: 0 Comments: 1

Question Number 94296 Answers: 0 Comments: 0

Exercise ABC is a triangle. A′ ; B′ ; C′ are respec− tively middles of sides: [BC]; [AC] and [AB]. G is isobarycenter( situated at equal distance ) of A, G , and C. 1) By using the theorem of medians, show that: GB^2 +GC^2 =(1/2)GA^2 +(1/2)BC^2

$$\mathrm{Exercise} \\ $$$$\mathrm{ABC}\:\mathrm{is}\:\mathrm{a}\:\mathrm{triangle}.\:\mathrm{A}'\:;\:\mathrm{B}'\:;\:\mathrm{C}'\:\mathrm{are}\:\mathrm{respec}− \\ $$$$\mathrm{tively}\:\mathrm{middles}\:\mathrm{of}\:\mathrm{sides}:\:\left[\mathrm{BC}\right];\:\left[\mathrm{AC}\right]\:\mathrm{and}\:\left[\mathrm{AB}\right]. \\ $$$$\mathrm{G}\:\mathrm{is}\:\mathrm{isobarycenter}\left(\:\mathrm{situated}\:\mathrm{at}\:\mathrm{equal}\:\mathrm{distance}\right. \\ $$$$\left.\right)\:\mathrm{of}\:\mathrm{A},\:\mathrm{G}\:,\:\mathrm{and}\:\mathrm{C}. \\ $$$$\left.\mathrm{1}\right)\:\mathrm{By}\:\mathrm{using}\:\mathrm{the}\:\mathrm{theorem}\:\mathrm{of}\:\mathrm{medians}, \\ $$$$\mathrm{show}\:\mathrm{that}: \\ $$$$\mathrm{GB}^{\mathrm{2}} +\mathrm{GC}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}}\mathrm{GA}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}\mathrm{BC}^{\mathrm{2}} \\ $$

Question Number 94291 Answers: 1 Comments: 2

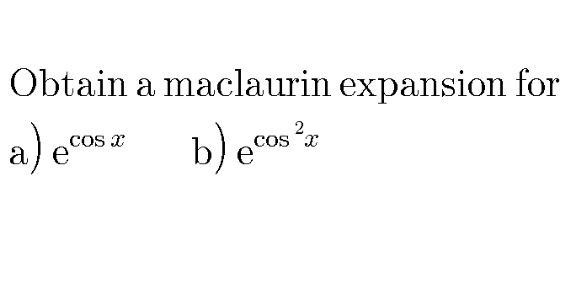
Question Number 94286 Answers: 0 Comments: 0

Question Number 94285 Answers: 1 Comments: 3

approximate ∫_0 ^(π/2) (x/(sinx))dx by simpsom method

$${approximate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{x}}{{sinx}}{dx}\:{by}\:{simpsom}\:{method} \\ $$

Question Number 94278 Answers: 0 Comments: 5

∫_0 ^1 (e^(√x) /(√x))dx

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{e}^{\sqrt{{x}}} }{\sqrt{{x}}}{dx} \\ $$

Question Number 94273 Answers: 0 Comments: 1

Question Number 94269 Answers: 0 Comments: 2

Pg 1204 Pg 1205 Pg 1206 Pg 1207 Pg 1208 Pg 1209 Pg 1210 Pg 1211 Pg 1212 Pg 1213

Contact: info@tinkutara.com