
AllQuestion and Answers: Page 1206
Question Number 94432 Answers: 0 Comments: 3
Question Number 95190 Answers: 1 Comments: 1

Question Number 94424 Answers: 1 Comments: 4
$$\mathrm{y}=\mathrm{x}^{\mathrm{x}} \\ $$$$\mathrm{y}^{'} =? \\ $$
Question Number 94419 Answers: 0 Comments: 0
Question Number 94418 Answers: 3 Comments: 0
Question Number 94416 Answers: 0 Comments: 0
Question Number 94397 Answers: 1 Comments: 0
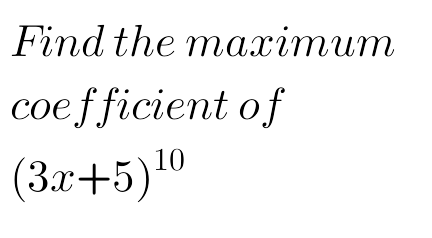
Question Number 94406 Answers: 0 Comments: 5

Question Number 94382 Answers: 1 Comments: 2
Question Number 94374 Answers: 0 Comments: 2
Question Number 94366 Answers: 1 Comments: 9
Question Number 94360 Answers: 1 Comments: 3

Question Number 94359 Answers: 0 Comments: 1
Question Number 94356 Answers: 0 Comments: 2
Question Number 94354 Answers: 1 Comments: 3
Question Number 94352 Answers: 0 Comments: 4

Question Number 94344 Answers: 1 Comments: 0
Question Number 94342 Answers: 0 Comments: 2
Question Number 94340 Answers: 1 Comments: 0
Question Number 94339 Answers: 0 Comments: 0
Question Number 94338 Answers: 1 Comments: 0
Question Number 94337 Answers: 3 Comments: 0
Question Number 94336 Answers: 2 Comments: 0
Question Number 94335 Answers: 2 Comments: 0
Question Number 94334 Answers: 1 Comments: 0
Question Number 94333 Answers: 1 Comments: 0
Pg 1201 Pg 1202 Pg 1203 Pg 1204 Pg 1205 Pg 1206 Pg 1207 Pg 1208 Pg 1209 Pg 1210
