Question and Answers Forum

AllQuestion and Answers: Page 1205

Question Number 94654 Answers: 0 Comments: 0

prove that Σ_(i=1) ^n x_i y_i ≤(Σ_(i=1) ^n x_i ^2 )^(1/2) (Σ_(i=1) ^n y_i ^2 )^(1/2) x_i and y_i reals ≥0

$${prove}\:{that}\:\sum_{{i}=\mathrm{1}} ^{{n}} \:{x}_{{i}} {y}_{{i}} \leqslant\left(\sum_{{i}=\mathrm{1}} ^{{n}} {x}_{{i}} ^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{2}}} \left(\sum_{{i}=\mathrm{1}} ^{{n}} {y}_{{i}} ^{\mathrm{2}} \right)^{\frac{\mathrm{1}}{\mathrm{2}}} \\ $$$${x}_{{i}} \:{and}\:{y}_{{i}} \:{reals}\:\geqslant\mathrm{0} \\ $$

Question Number 94652 Answers: 0 Comments: 0

find lim_(ξ→0) ∫_0 ^1 ((sin(ξx))/((√(1+ξx^2 ))−(√(1−ξx^2 ))))dx

$${find}\:{lim}_{\xi\rightarrow\mathrm{0}} \:\:\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\:\:\:\:\frac{{sin}\left(\xi{x}\right)}{\sqrt{\mathrm{1}+\xi{x}^{\mathrm{2}} }−\sqrt{\mathrm{1}−\xi{x}^{\mathrm{2}} }}{dx} \\ $$

Question Number 94650 Answers: 1 Comments: 1

let f(x) =(x+1)^9 e^(−3x) calculstr f^((7)) (0) and f^((5)) (1)

$${let}\:{f}\left({x}\right)\:=\left({x}+\mathrm{1}\right)^{\mathrm{9}} \:{e}^{−\mathrm{3}{x}} \\ $$$${calculstr}\:{f}^{\left(\mathrm{7}\right)} \left(\mathrm{0}\right)\:{and}\:{f}^{\left(\mathrm{5}\right)} \left(\mathrm{1}\right) \\ $$

Question Number 94649 Answers: 0 Comments: 0

calculate ∫_0 ^∞ e^(−x) lnx dx

$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{x}} {lnx}\:{dx} \\ $$

Question Number 94648 Answers: 0 Comments: 0

find a equivslent for u_n =1 +2^α +3^α +....+n^α n→+∞ (α>0)

$${find}\:{a}\:{equivslent}\:{for} \\ $$$${u}_{{n}} =\mathrm{1}\:+\mathrm{2}^{\alpha} \:+\mathrm{3}^{\alpha} \:+....+{n}^{\alpha} \\ $$$${n}\rightarrow+\infty\:\:\:\:\:\:\:\:\:\left(\alpha>\mathrm{0}\right) \\ $$

Question Number 94635 Answers: 1 Comments: 0

A father with 8 children takes them 3 at a time to the Gardens, as often as he can without taking the same 3 children together more than once. The number of times each child will go to the garden is

$$\mathrm{A}\:\mathrm{father}\:\mathrm{with}\:\mathrm{8}\:\mathrm{children}\:\mathrm{takes}\:\mathrm{them} \\ $$$$\mathrm{3}\:\mathrm{at}\:\mathrm{a}\:\mathrm{time}\:\mathrm{to}\:\mathrm{the}\:\mathrm{Gardens},\:\mathrm{as}\:\mathrm{often}\:\mathrm{as} \\ $$$$\mathrm{he}\:\mathrm{can}\:\mathrm{without}\:\mathrm{taking}\:\mathrm{the}\:\mathrm{same}\:\mathrm{3}\:\mathrm{children} \\ $$$$\mathrm{together}\:\mathrm{more}\:\mathrm{than}\:\mathrm{once}.\:\mathrm{The}\:\mathrm{number} \\ $$$$\mathrm{of}\:\mathrm{times}\:\mathrm{each}\:\mathrm{child}\:\mathrm{will}\:\mathrm{go}\:\mathrm{to}\:\mathrm{the}\:\mathrm{garden} \\ $$$$\mathrm{is} \\ $$

Question Number 94632 Answers: 0 Comments: 1

The coefficient of x^m and x^n (m, n ∈ N) in the expansion of (1+x)^(m+n) are

$$\mathrm{The}\:\mathrm{coefficient}\:\mathrm{of}\:{x}^{{m}} \:\mathrm{and}\:{x}^{{n}} \left({m},\:{n}\:\in\:{N}\right)\:\mathrm{in} \\ $$$$\mathrm{the}\:\mathrm{expansion}\:\mathrm{of}\:\left(\mathrm{1}+{x}\right)^{{m}+{n}} \:\mathrm{are} \\ $$

Question Number 94629 Answers: 1 Comments: 0

In △ABC, a=(√3)+1, B=30°, C=45°, then c = ____.

$$\mathrm{In}\:\bigtriangleup{ABC},\:{a}=\sqrt{\mathrm{3}}+\mathrm{1},\:{B}=\mathrm{30}°,\:{C}=\mathrm{45}°, \\ $$$$\mathrm{then}\:{c}\:=\:\_\_\_\_. \\ $$

Question Number 94628 Answers: 1 Comments: 0

The perimeter of a △ ABC is 6 times the arithmetic mean of the sines of its angles. If the side a is 1, then the angle A is

$$\mathrm{The}\:\mathrm{perimeter}\:\mathrm{of}\:\mathrm{a}\:\bigtriangleup\:{ABC}\:\mathrm{is}\:\mathrm{6}\:\mathrm{times} \\ $$$$\mathrm{the}\:\mathrm{arithmetic}\:\mathrm{mean}\:\mathrm{of}\:\mathrm{the}\:\mathrm{sines}\:\mathrm{of} \\ $$$$\mathrm{its}\:\mathrm{angles}.\:\mathrm{If}\:\mathrm{the}\:\mathrm{side}\:{a}\:\mathrm{is}\:\:\mathrm{1},\:\mathrm{then}\:\mathrm{the} \\ $$$$\mathrm{angle}\:{A}\:\:\mathrm{is} \\ $$

Question Number 94625 Answers: 0 Comments: 3

∫_0 ^1 ln (Γ(x) dx =? note Γ(x) :Gamma function

$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\mathrm{ln}\:\left(\Gamma\left({x}\right)\:{dx}\:=?\right. \\ $$$${note}\:\Gamma\left({x}\right)\::\mathrm{Gamma}\:\mathrm{function} \\ $$

Question Number 94622 Answers: 0 Comments: 0

Question Number 94616 Answers: 1 Comments: 0

S=(√(((fx^(^ 2) )/n)−(((fx)/n))^(^ 2) ))

$${S}=\sqrt{\frac{{f}\overset{\hat {}\mathrm{2}} {{x}}}{{n}}−\left(\frac{{fx}}{{n}}\overset{\hat {}\mathrm{2}} {\right)}} \\ $$

Question Number 94609 Answers: 2 Comments: 0

∫((x^2 −1)/((√(x+1))+(√(2x+3))))dx

$$\int\frac{{x}^{\mathrm{2}} −\mathrm{1}}{\sqrt{{x}+\mathrm{1}}+\sqrt{\mathrm{2}{x}+\mathrm{3}}}{dx} \\ $$

Question Number 94613 Answers: 1 Comments: 3

Question Number 94603 Answers: 1 Comments: 1

List the elements in C={x:x is an x^2 ≤4, integer}

$$\mathrm{List}\:\mathrm{the}\:\mathrm{elements}\:\mathrm{in}\: \\ $$$${C}=\left\{{x}:{x}\:\mathrm{is}\:\mathrm{an}\:{x}^{\mathrm{2}} \leqslant\mathrm{4},\:\mathrm{integer}\right\} \\ $$

Question Number 94602 Answers: 1 Comments: 0

Question Number 94601 Answers: 1 Comments: 0

Question Number 94589 Answers: 0 Comments: 2

covert the point(p,θ,ϕ)=[4(√3),(π/6),(π/3)] to cartesian coordinates.

$${covert}\:{the}\:{point}\left({p},\theta,\varphi\right)=\left[\mathrm{4}\sqrt{\mathrm{3}},\frac{\pi}{\mathrm{6}},\frac{\pi}{\mathrm{3}}\right]\: \\ $$$${to}\:{cartesian}\:{coordinates}. \\ $$

Question Number 94581 Answers: 0 Comments: 2

Question Number 94573 Answers: 1 Comments: 2

Given a, b, and c, 3 real numbers which satisfy the equation { ((a+b+c=312)),((c+a=192)) :} Find these real numbers such that they form 3 consecutive terms of a Geometric Progression.

$$\mathrm{Given}\:\mathrm{a},\:\mathrm{b},\:\mathrm{and}\:\mathrm{c},\:\mathrm{3}\:\mathrm{real}\:\mathrm{numbers}\:\mathrm{which}\:\mathrm{satisfy} \\ $$$$\mathrm{the}\:\mathrm{equation}\:\begin{cases}{\mathrm{a}+\mathrm{b}+\mathrm{c}=\mathrm{312}}\\{\mathrm{c}+\mathrm{a}=\mathrm{192}}\end{cases} \\ $$$$\mathrm{Find}\:\mathrm{these}\:\mathrm{real}\:\mathrm{numbers}\:\mathrm{such}\:\mathrm{that}\:\mathrm{they}\:\mathrm{form} \\ $$$$\mathrm{3}\:\mathrm{consecutive}\:\mathrm{terms}\:\mathrm{of}\:\mathrm{a}\:\mathrm{Geometric}\:\mathrm{Progression}. \\ $$

Question Number 94572 Answers: 0 Comments: 0

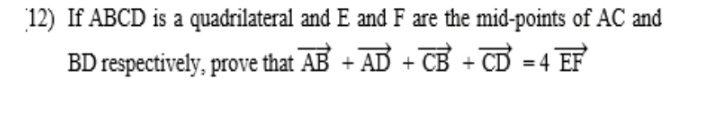
Question Number 94579 Answers: 1 Comments: 0

A study indicates that x months from now the population of a certain town will be decreasing at the rate of 5+3x^(2/3) people per month. By how much will the population of the town increase per the next 8 months. I need help with the above question, please.

$$\mathrm{A}\:\mathrm{study}\:\mathrm{indicates}\:\mathrm{that}\:{x}\:\mathrm{months}\:\mathrm{from}\:\mathrm{now} \\ $$$$\mathrm{the}\:\mathrm{population}\:\mathrm{of}\:\mathrm{a}\:\mathrm{certain}\:\mathrm{town}\:\mathrm{will}\:\mathrm{be}\: \\ $$$$\mathrm{decreasing}\:\mathrm{at}\:\mathrm{the}\:\mathrm{rate}\:\mathrm{of}\:\mathrm{5}+\mathrm{3}{x}^{\frac{\mathrm{2}}{\mathrm{3}}} \:\mathrm{people} \\ $$$$\mathrm{per}\:\mathrm{month}.\:\mathrm{By}\:\mathrm{how}\:\mathrm{much}\:\mathrm{will}\:\mathrm{the}\:\mathrm{population} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{town}\:\mathrm{increase}\:\mathrm{per}\:\mathrm{the}\:\mathrm{next}\:\mathrm{8}\:\mathrm{months}. \\ $$$$\boldsymbol{{I}}\:\boldsymbol{{need}}\:\boldsymbol{{help}}\:\boldsymbol{{with}}\:\boldsymbol{{the}}\:\boldsymbol{{above}}\:\boldsymbol{{question}},\:\boldsymbol{{please}}. \\ $$

Question Number 94559 Answers: 0 Comments: 6

Question Number 94556 Answers: 2 Comments: 1

Question Number 94553 Answers: 1 Comments: 0

solve ((∣x+2∣−x)/x) < 2

$$\mathrm{solve}\:\frac{\mid{x}+\mathrm{2}\mid−{x}}{{x}}\:<\:\mathrm{2}\: \\ $$

Question Number 94544 Answers: 0 Comments: 0

1\Show that the function f(x)=[x] is of Riemann for all segments of R 2\Show that the function f(x) defined within x∈[0,1] f(x)= { ((1 if x∈Q∩[0,1])),((0 otherwise)) :} is not of Riemann on x∈[0,1]

$$\mathrm{1}\backslash\mathrm{Show}\:\mathrm{that}\:\mathrm{the}\:\mathrm{function}\:\mathrm{f}\left(\mathrm{x}\right)=\left[\mathrm{x}\right]\:\mathrm{is}\:\mathrm{of}\:\mathrm{Riemann} \\ $$$$\mathrm{for}\:\mathrm{all}\:\mathrm{segments}\:\mathrm{of}\:\mathbb{R} \\ $$$$\mathrm{2}\backslash\mathrm{Show}\:\mathrm{that}\:\mathrm{the}\:\mathrm{function}\:\mathrm{f}\left(\mathrm{x}\right)\:\mathrm{defined}\:\mathrm{within}\:\mathrm{x}\in\left[\mathrm{0},\mathrm{1}\right] \\ $$$$\mathrm{f}\left(\mathrm{x}\right)=\begin{cases}{\mathrm{1}\:\mathrm{if}\:\mathrm{x}\in\mathbb{Q}\cap\left[\mathrm{0},\mathrm{1}\right]}\\{\mathrm{0}\:\:\mathrm{otherwise}}\end{cases}\:\:\mathrm{is}\:\mathrm{not}\:\mathrm{of}\:\mathrm{Riemann}\:\mathrm{on}\:\mathrm{x}\in\left[\mathrm{0},\mathrm{1}\right] \\ $$

Pg 1200 Pg 1201 Pg 1202 Pg 1203 Pg 1204 Pg 1205 Pg 1206 Pg 1207 Pg 1208 Pg 1209

Contact: info@tinkutara.com