
AllQuestion and Answers: Page 1204
Question Number 94753 Answers: 1 Comments: 0

Question Number 94730 Answers: 2 Comments: 0
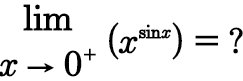
Question Number 94723 Answers: 2 Comments: 0
Question Number 94718 Answers: 0 Comments: 4
Question Number 94710 Answers: 1 Comments: 2

Question Number 94708 Answers: 1 Comments: 1

Question Number 94706 Answers: 2 Comments: 0

Question Number 94705 Answers: 0 Comments: 1
Question Number 94701 Answers: 0 Comments: 3
Question Number 94698 Answers: 1 Comments: 0

Question Number 94693 Answers: 0 Comments: 1

Question Number 94690 Answers: 0 Comments: 4

Question Number 94679 Answers: 2 Comments: 2
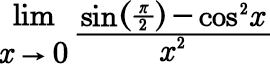
Question Number 94676 Answers: 0 Comments: 0
Question Number 94664 Answers: 0 Comments: 9
Question Number 94662 Answers: 1 Comments: 3
$$\int\:\sqrt{\mathrm{tan}\:\mathrm{x}+\mathrm{cot}\:\mathrm{x}}\:\mathrm{dx}\:=\:? \\ $$
Question Number 94661 Answers: 0 Comments: 0
Question Number 94660 Answers: 0 Comments: 0
Question Number 94659 Answers: 1 Comments: 0
Question Number 94658 Answers: 0 Comments: 0
Question Number 94657 Answers: 0 Comments: 0
Question Number 94655 Answers: 0 Comments: 0
Question Number 94654 Answers: 0 Comments: 0
Question Number 94652 Answers: 0 Comments: 0
Question Number 94650 Answers: 1 Comments: 1
Question Number 94649 Answers: 0 Comments: 0
$${calculate}\:\int_{\mathrm{0}} ^{\infty} \:{e}^{−{x}} {lnx}\:{dx} \\ $$
Pg 1199 Pg 1200 Pg 1201 Pg 1202 Pg 1203 Pg 1204 Pg 1205 Pg 1206 Pg 1207 Pg 1208
