Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1203

Question Number 94328 Answers: 2 Comments: 0

y′ + xy = x

$$\mathrm{y}'\:+\:\mathrm{xy}\:=\:\mathrm{x}\: \\ $$

Question Number 94324 Answers: 0 Comments: 0

Question Number 94319 Answers: 0 Comments: 4

Question Number 94318 Answers: 0 Comments: 2

Given f(xy) = f(x+y) and f(7) = 7. find f(1008)

$$\mathrm{Given}\:\mathrm{f}\left(\mathrm{xy}\right)\:=\:\mathrm{f}\left(\mathrm{x}+\mathrm{y}\right)\:\mathrm{and}\: \\ $$$$\mathrm{f}\left(\mathrm{7}\right)\:=\:\mathrm{7}.\:\mathrm{find}\:\mathrm{f}\left(\mathrm{1008}\right)\: \\ $$

Question Number 94341 Answers: 0 Comments: 0

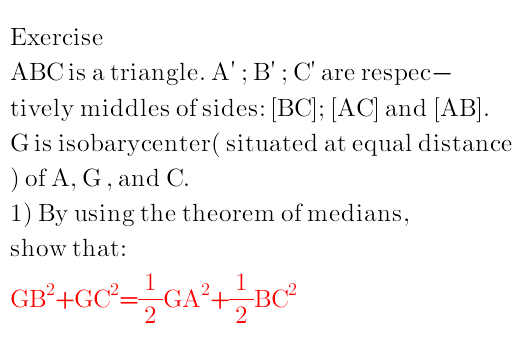
Question Number 94314 Answers: 0 Comments: 0

Question Number 94313 Answers: 0 Comments: 0

Question Number 94312 Answers: 0 Comments: 3

∫_0 ^a ((a^2 −x^2 )/((a^2 +x^2 )^2 )) dx ?

$$\underset{\mathrm{0}} {\overset{{a}} {\int}}\:\frac{{a}^{\mathrm{2}} −{x}^{\mathrm{2}} }{\left({a}^{\mathrm{2}} +{x}^{\mathrm{2}} \right)^{\mathrm{2}} }\:{dx}\:? \\ $$

Question Number 94311 Answers: 0 Comments: 0

explicit f(a) =∫_0 ^1 ((ln(1−ax^2 ))/x^2 )dx with 0<a<1

$${explicit}\:{f}\left({a}\right)\:=\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}−{ax}^{\mathrm{2}} \right)}{{x}^{\mathrm{2}} }{dx}\:{with}\:\mathrm{0}<{a}<\mathrm{1} \\ $$

Question Number 94310 Answers: 2 Comments: 0

calculate ∫_0 ^1 ((ln(1−x^2 ))/x^2 )dx

$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{1}} \:\frac{{ln}\left(\mathrm{1}−{x}^{\mathrm{2}} \right)}{{x}^{\mathrm{2}} }{dx} \\ $$$$ \\ $$

Question Number 94309 Answers: 0 Comments: 0

Question Number 94298 Answers: 1 Comments: 0

Question Number 94297 Answers: 0 Comments: 1

Question Number 94296 Answers: 0 Comments: 0

Exercise ABC is a triangle. A′ ; B′ ; C′ are respec− tively middles of sides: [BC]; [AC] and [AB]. G is isobarycenter( situated at equal distance ) of A, G , and C. 1) By using the theorem of medians, show that: GB^2 +GC^2 =(1/2)GA^2 +(1/2)BC^2

$$\mathrm{Exercise} \\ $$$$\mathrm{ABC}\:\mathrm{is}\:\mathrm{a}\:\mathrm{triangle}.\:\mathrm{A}'\:;\:\mathrm{B}'\:;\:\mathrm{C}'\:\mathrm{are}\:\mathrm{respec}− \\ $$$$\mathrm{tively}\:\mathrm{middles}\:\mathrm{of}\:\mathrm{sides}:\:\left[\mathrm{BC}\right];\:\left[\mathrm{AC}\right]\:\mathrm{and}\:\left[\mathrm{AB}\right]. \\ $$$$\mathrm{G}\:\mathrm{is}\:\mathrm{isobarycenter}\left(\:\mathrm{situated}\:\mathrm{at}\:\mathrm{equal}\:\mathrm{distance}\right. \\ $$$$\left.\right)\:\mathrm{of}\:\mathrm{A},\:\mathrm{G}\:,\:\mathrm{and}\:\mathrm{C}. \\ $$$$\left.\mathrm{1}\right)\:\mathrm{By}\:\mathrm{using}\:\mathrm{the}\:\mathrm{theorem}\:\mathrm{of}\:\mathrm{medians}, \\ $$$$\mathrm{show}\:\mathrm{that}: \\ $$$$\mathrm{GB}^{\mathrm{2}} +\mathrm{GC}^{\mathrm{2}} =\frac{\mathrm{1}}{\mathrm{2}}\mathrm{GA}^{\mathrm{2}} +\frac{\mathrm{1}}{\mathrm{2}}\mathrm{BC}^{\mathrm{2}} \\ $$

Question Number 94291 Answers: 1 Comments: 2

Question Number 94286 Answers: 0 Comments: 0

Question Number 94285 Answers: 1 Comments: 3

approximate ∫_0 ^(π/2) (x/(sinx))dx by simpsom method

$${approximate}\:\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{{x}}{{sinx}}{dx}\:{by}\:{simpsom}\:{method} \\ $$

Question Number 94278 Answers: 0 Comments: 5

∫_0 ^1 (e^(√x) /(√x))dx

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{e}^{\sqrt{{x}}} }{\sqrt{{x}}}{dx} \\ $$

Question Number 94273 Answers: 0 Comments: 1

Question Number 94269 Answers: 0 Comments: 2

Question Number 94257 Answers: 2 Comments: 0

If 9y^2 + (1/y^2 ) =3, then find the value of 27y^3 + (1/y^3 )

$$\mathrm{If}\:\mathrm{9y}^{\mathrm{2}} +\:\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{2}} }\:=\mathrm{3},\:\mathrm{then}\:\mathrm{find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of} \\ $$$$\mathrm{27y}^{\mathrm{3}} \:+\:\frac{\mathrm{1}}{\mathrm{y}^{\mathrm{3}} } \\ $$

Question Number 94245 Answers: 1 Comments: 0

find the function f(x) satisfying the given conditions (i)f^′ (x)=4x^2 −1 , f(0)=3 ? (ii)f^(′′) (x)=12 , f^′ (0)=2 , f(0)=3 ? (iii)f^(′′) (x)=2x , f^′ (0)=−3 , f(0)=2 ? help me sir pleas ?

$${find}\:{the}\:{function}\:{f}\left({x}\right)\:{satisfying}\:{the}\:{given}\:{conditions} \\ $$$$\left({i}\right){f}^{'} \left({x}\right)=\mathrm{4}{x}^{\mathrm{2}} −\mathrm{1}\:\:\:,\:{f}\left(\mathrm{0}\right)=\mathrm{3}\:? \\ $$$$\left({ii}\right){f}^{''} \left({x}\right)=\mathrm{12}\:\:,\:{f}^{'} \left(\mathrm{0}\right)=\mathrm{2}\:\:,\:{f}\left(\mathrm{0}\right)=\mathrm{3}\:? \\ $$$$\left({iii}\right){f}^{''} \left({x}\right)=\mathrm{2}{x}\:\:,\:\:{f}^{'} \left(\mathrm{0}\right)=−\mathrm{3}\:\:,\:{f}\left(\mathrm{0}\right)=\mathrm{2}\:? \\ $$$$ \\ $$$${help}\:{me}\:{sir}\:{pleas}\:? \\ $$

Question Number 94241 Answers: 1 Comments: 1

Prove that arctan(x)+2arctan((√(1+x^2 ))−x)=(π/2)

$$\mathrm{Prove}\:\mathrm{that}\: \\ $$$$\mathrm{arctan}\left(\mathrm{x}\right)+\mathrm{2arctan}\left(\sqrt{\mathrm{1}+\mathrm{x}^{\mathrm{2}} }−\mathrm{x}\right)=\frac{\pi}{\mathrm{2}} \\ $$

Question Number 94239 Answers: 1 Comments: 0

x^2 (dy/dx) = x^2 +xy+y^2

$$\mathrm{x}^{\mathrm{2}} \:\frac{\mathrm{dy}}{\mathrm{dx}}\:=\:\mathrm{x}^{\mathrm{2}} +\mathrm{xy}+\mathrm{y}^{\mathrm{2}} \\ $$

Question Number 94237 Answers: 1 Comments: 1

Question Number 94243 Answers: 0 Comments: 3

By using the double integral find the area of the regiin bounded by the curve y=x^2 +2x , y=x^2 −2x and x−axis (sketch the region of integration) pleas sir help me

$${By}\:{using}\:{the}\:{double}\:{integral}\:{find}\:{the}\:{area}\:{of}\:{the}\:{regiin}\: \\ $$$${bounded}\:{by}\:{the}\:{curve}\:{y}={x}^{\mathrm{2}} +\mathrm{2}{x}\:,\:{y}={x}^{\mathrm{2}} −\mathrm{2}{x}\:{and}\:{x}−{axis}\: \\ $$$$\left({sketch}\:{the}\:{region}\:{of}\:{integration}\right) \\ $$$${pleas}\:{sir}\:{help}\:{me}\: \\ $$

Pg 1198 Pg 1199 Pg 1200 Pg 1201 Pg 1202 Pg 1203 Pg 1204 Pg 1205 Pg 1206 Pg 1207

Contact: info@tinkutara.com