Question and Answers Forum

AllQuestion and Answers: Page 1202

Question Number 94992 Answers: 4 Comments: 0

1. Show that: ∫_0 ^( π) ((xdx)/((a^2 sin^2 x+b^2 cos^2 x)^2 )) = ((π^2 (a^2 +b^2 ))/(4a^3 b^3 )) 2.The density at the point (x,y) of a lamina bounded by the circle x^2 +y^2 −2ax=0 is ϱ =x find its mass. 3.∗ 4. If z= ((cos y)/x) and x=u^2 −v , y=e^x find (dz/dv).

$$\:\mathrm{1}.\:\mathrm{Show}\:\:\mathrm{that}: \\ $$$$\:\:\:\int_{\mathrm{0}} ^{\:\:\pi} \:\:\:\frac{\mathrm{xdx}}{\left(\mathrm{a}^{\mathrm{2}} \mathrm{sin}^{\mathrm{2}} \mathrm{x}+\mathrm{b}^{\mathrm{2}} \mathrm{cos}^{\mathrm{2}} \mathrm{x}\right)^{\mathrm{2}} }\:=\:\frac{\pi^{\mathrm{2}} \left(\mathrm{a}^{\mathrm{2}} +\mathrm{b}^{\mathrm{2}} \right)}{\mathrm{4a}^{\mathrm{3}} \mathrm{b}^{\mathrm{3}} } \\ $$$$\:\: \\ $$$$\:\mathrm{2}.\mathrm{The}\:\mathrm{density}\:\mathrm{at}\:\mathrm{the}\:\mathrm{point}\:\left(\mathrm{x},\mathrm{y}\right)\:\mathrm{of}\:\mathrm{a}\:\mathrm{lamina}\:\mathrm{bounded}\:\mathrm{by}\:\mathrm{the}\:\mathrm{circle} \\ $$$$\:\:\:\:\:\:\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} −\mathrm{2ax}=\mathrm{0}\:\mathrm{is}\:\varrho\:=\mathrm{x}\:\:\:\mathrm{find}\:\mathrm{its}\:\mathrm{mass}. \\ $$$$\:\mathrm{3}.\ast \\ $$$$\:\mathrm{4}.\:\mathrm{If}\:\:\mathrm{z}=\:\frac{\mathrm{cos}\:\mathrm{y}}{\mathrm{x}}\:\mathrm{and}\:\mathrm{x}=\mathrm{u}^{\mathrm{2}} −\mathrm{v}\:,\:\mathrm{y}=\mathrm{e}^{\mathrm{x}} \:\mathrm{find}\:\frac{\mathrm{dz}}{\mathrm{dv}}. \\ $$$$\:\: \\ $$

Question Number 94984 Answers: 1 Comments: 2

(d/dx) [(1/x) +(√x) ] ?

$$\frac{\mathrm{d}}{\mathrm{dx}}\:\left[\frac{\mathrm{1}}{\mathrm{x}}\:+\sqrt{\mathrm{x}}\:\right]\:? \\ $$

Question Number 94969 Answers: 1 Comments: 0

y′′−3y′+2y=0 ; y=0,y′=3 for x=0

$$\mathrm{y}''−\mathrm{3y}'+\mathrm{2y}=\mathrm{0}\:;\:\mathrm{y}=\mathrm{0},\mathrm{y}'=\mathrm{3}\:\mathrm{for}\:\mathrm{x}=\mathrm{0} \\ $$

Question Number 94960 Answers: 2 Comments: 0

Question Number 94956 Answers: 1 Comments: 7

Question Number 94955 Answers: 0 Comments: 0

Prove by set theory or otherwise lcm( gcd(x,y),gcd(y,z),gcd(z,x) ) is equal to gcd( lcm(x,y),lcm(y,z),lcm(z,x) ) Or give a counter example.

$${Prove}\:{by}\:{set}\:{theory}\:{or}\:{otherwise} \\ $$$$\:\:\mathrm{lcm}\left(\:\mathrm{gcd}\left(\mathrm{x},\mathrm{y}\right),\mathrm{gcd}\left(\mathrm{y},\mathrm{z}\right),\mathrm{gcd}\left(\mathrm{z},\mathrm{x}\right)\:\right) \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{is}\:\mathrm{equal}\:\mathrm{to} \\ $$$$\:\mathrm{gcd}\left(\:\mathrm{lcm}\left(\mathrm{x},\mathrm{y}\right),\mathrm{lcm}\left(\mathrm{y},\mathrm{z}\right),\mathrm{lcm}\left(\mathrm{z},\mathrm{x}\right)\:\right) \\ $$$$\:\:{Or}\:{give}\:{a}\:{counter}\:{example}. \\ $$

Question Number 94954 Answers: 1 Comments: 0

For any curve, prove that: ((d^2 x/ds^2 ))^2 +((d^2 y/dx^2 ))^2 = (1/ρ^2 )

$$\:\:\boldsymbol{\mathrm{For}}\:\boldsymbol{\mathrm{any}}\:\boldsymbol{\mathrm{curve}},\:\boldsymbol{\mathrm{prove}}\:\boldsymbol{\mathrm{that}}: \\ $$$$\:\:\:\left(\frac{\mathrm{d}^{\mathrm{2}} \mathrm{x}}{\mathrm{ds}^{\mathrm{2}} }\right)^{\mathrm{2}} +\left(\frac{\mathrm{d}^{\mathrm{2}} \mathrm{y}}{\mathrm{dx}^{\mathrm{2}} }\right)^{\mathrm{2}} =\:\frac{\mathrm{1}}{\rho^{\mathrm{2}} } \\ $$$$\:\: \\ $$

Question Number 94945 Answers: 0 Comments: 2

How do you make p the subject of equation q = (m/((√p) )) + (p^2 /m)

$$\mathrm{How}\:\mathrm{do}\:\mathrm{you}\:\mathrm{make}\:\mathrm{p}\:\mathrm{the}\:\mathrm{subject} \\ $$$$\mathrm{of}\:\mathrm{equation}\:{q}\:=\:\frac{{m}}{\sqrt{{p}}\:}\:+\:\frac{{p}^{\mathrm{2}} }{{m}}\: \\ $$

Question Number 94934 Answers: 3 Comments: 1

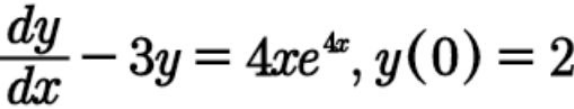
Question Number 94931 Answers: 1 Comments: 0

f is a 2(×) derivable function and L laplace transfom determine L(f^′ ) a L(f^(′′) )

$$\mathrm{f}\:\mathrm{is}\:\mathrm{a}\:\mathrm{2}\left(×\right)\:\mathrm{derivable}\:\mathrm{function}\:\:\mathrm{and}\:\mathrm{L}\:\mathrm{laplace}\:\mathrm{transfom} \\ $$$$\mathrm{determine}\:\mathrm{L}\left(\mathrm{f}^{'} \right)\:\mathrm{a}\:\mathrm{L}\left(\mathrm{f}^{''} \right) \\ $$

Question Number 94925 Answers: 1 Comments: 2

Question Number 94923 Answers: 1 Comments: 0

Question Number 94922 Answers: 1 Comments: 0

Question Number 94921 Answers: 2 Comments: 0

Question Number 94915 Answers: 2 Comments: 0

We suppose in R^2 the base (i^→ ;j^→ ). we have these vectors: u^→ =(m^2 −m)i^→ +2mj^→ ; v^→ =(m−1)i^→ +(m+1)j^→ m ∈ R^∗ 1)Determinate m for which the system (u^→ ;v^→ ) is linear dependant( det(u^→ ;v^→ )=0)

Question Number 94914 Answers: 1 Comments: 0