
AllQuestion and Answers: Page 1199
Question Number 95396 Answers: 0 Comments: 2
Question Number 95394 Answers: 0 Comments: 31
Question Number 95378 Answers: 1 Comments: 1
Question Number 95373 Answers: 1 Comments: 2
Question Number 95371 Answers: 2 Comments: 1
Question Number 95363 Answers: 2 Comments: 1
Question Number 95328 Answers: 0 Comments: 3

Question Number 95326 Answers: 0 Comments: 4
Question Number 95325 Answers: 0 Comments: 1
Question Number 95324 Answers: 0 Comments: 2
Question Number 95323 Answers: 1 Comments: 0
Question Number 95316 Answers: 1 Comments: 0
Question Number 95309 Answers: 1 Comments: 0
Question Number 95294 Answers: 1 Comments: 2
Question Number 95277 Answers: 2 Comments: 4

Question Number 95269 Answers: 0 Comments: 3

Question Number 100664 Answers: 1 Comments: 0
Question Number 95262 Answers: 3 Comments: 0
Question Number 95260 Answers: 1 Comments: 3
Question Number 95259 Answers: 1 Comments: 1
Question Number 95246 Answers: 4 Comments: 0
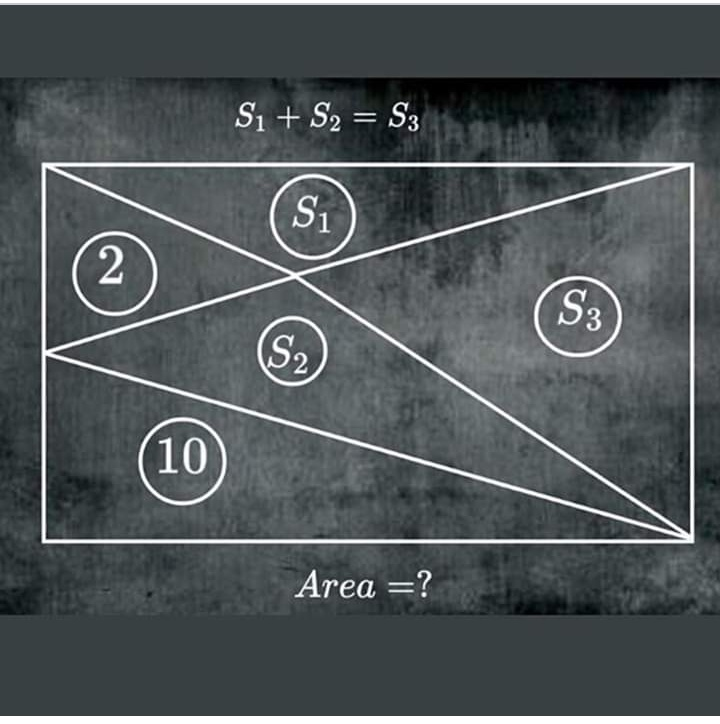
Question Number 95232 Answers: 3 Comments: 6

Question Number 95230 Answers: 1 Comments: 0
Question Number 95339 Answers: 6 Comments: 0
Question Number 95227 Answers: 1 Comments: 0
Question Number 95222 Answers: 1 Comments: 0
Pg 1194 Pg 1195 Pg 1196 Pg 1197 Pg 1198 Pg 1199 Pg 1200 Pg 1201 Pg 1202 Pg 1203
