
AllQuestion and Answers: Page 1185
Question Number 96979 Answers: 1 Comments: 0
$$\int_{\mathrm{0}} ^{\pi} {arctan}\left(\mathrm{3}^{{cos}\left({x}\right)} \right){dx} \\ $$
Question Number 96977 Answers: 1 Comments: 0
Question Number 96962 Answers: 1 Comments: 0
Question Number 96959 Answers: 1 Comments: 0
Question Number 96958 Answers: 0 Comments: 0
Question Number 96957 Answers: 2 Comments: 0
Question Number 96956 Answers: 2 Comments: 0
Question Number 96955 Answers: 1 Comments: 0
Question Number 96951 Answers: 1 Comments: 5
Question Number 96947 Answers: 0 Comments: 4

Question Number 96936 Answers: 2 Comments: 1
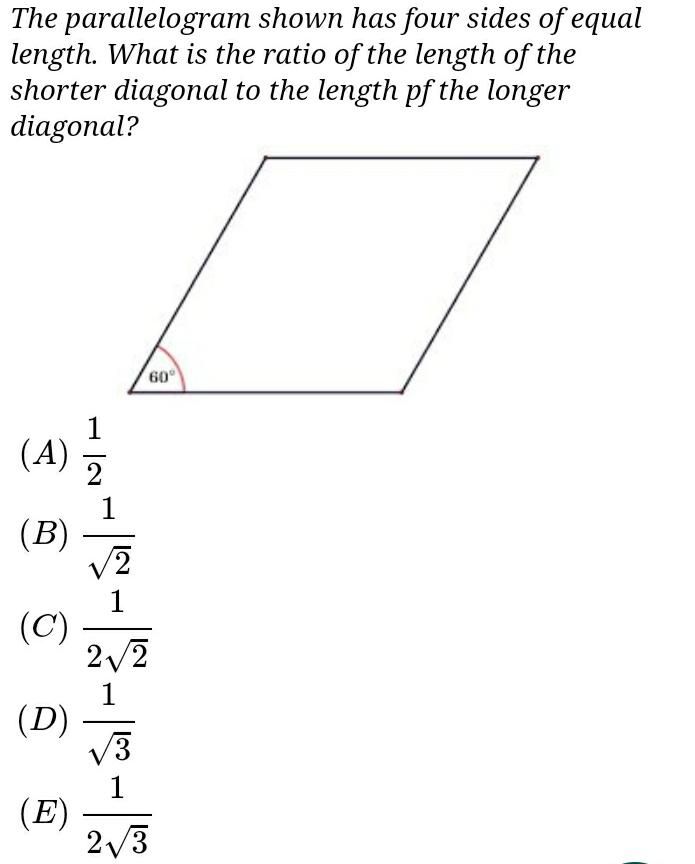
Question Number 96931 Answers: 2 Comments: 3
Question Number 96930 Answers: 0 Comments: 1
Question Number 96928 Answers: 3 Comments: 1
Question Number 96925 Answers: 2 Comments: 0
Question Number 96922 Answers: 0 Comments: 8
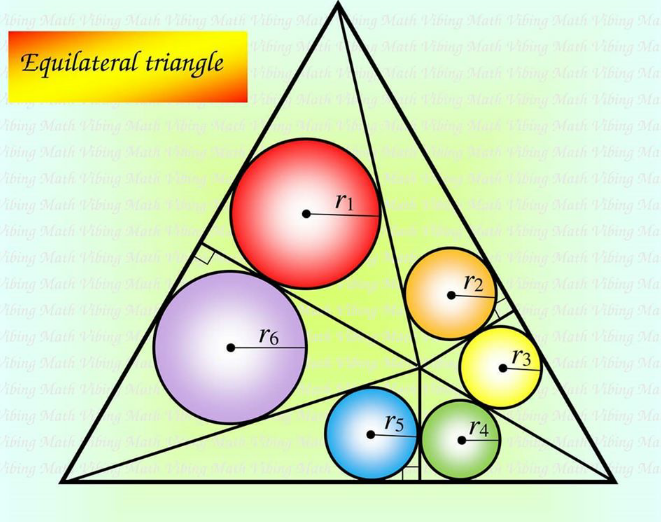
Question Number 96920 Answers: 0 Comments: 2
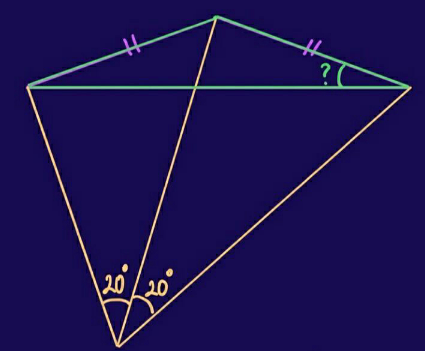
Question Number 96911 Answers: 1 Comments: 2
Question Number 96907 Answers: 0 Comments: 1
Question Number 96906 Answers: 1 Comments: 0
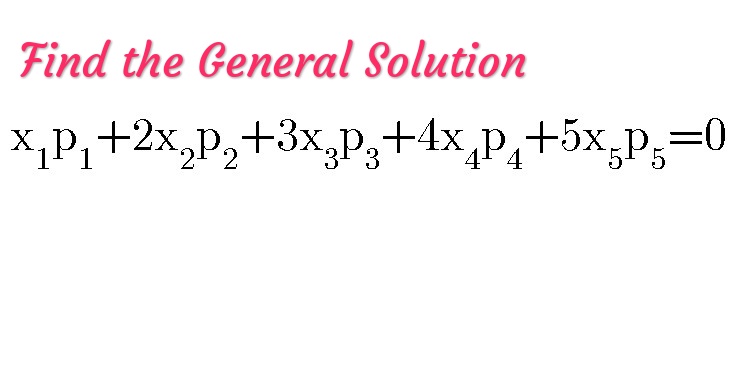
Question Number 96904 Answers: 0 Comments: 3
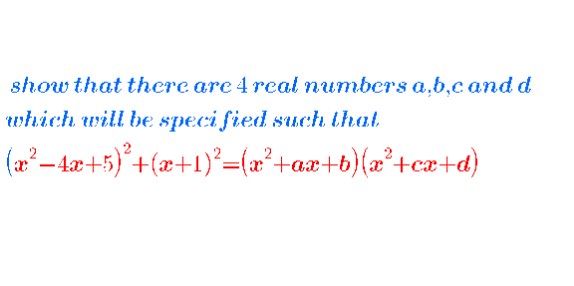
Question Number 96898 Answers: 2 Comments: 1

Question Number 96886 Answers: 0 Comments: 3
Question Number 96883 Answers: 0 Comments: 2
Question Number 96866 Answers: 1 Comments: 1

Question Number 96870 Answers: 2 Comments: 0

Pg 1180 Pg 1181 Pg 1182 Pg 1183 Pg 1184 Pg 1185 Pg 1186 Pg 1187 Pg 1188 Pg 1189
