Question and Answers Forum

AllQuestion and Answers: Page 1156

Question Number 100387 Answers: 1 Comments: 0

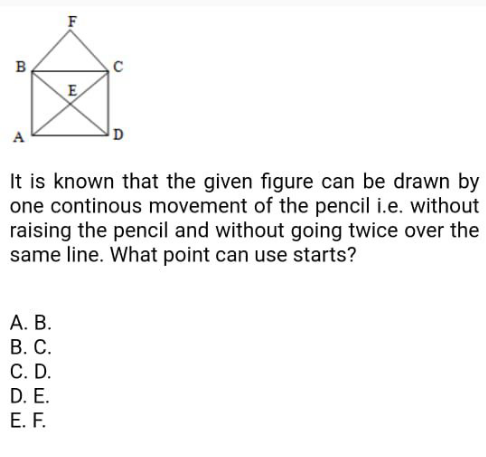
Question Number 100385 Answers: 1 Comments: 2

find the solution set of inequality (((x^2 −9)(√(x+2)))/(x+(√((x+2)^2 )))) ≤ 0

$$\mathrm{find}\:\mathrm{the}\:\mathrm{solution}\:\mathrm{set}\:\mathrm{of}\:\mathrm{inequality} \\ $$$$\frac{\left(\mathrm{x}^{\mathrm{2}} −\mathrm{9}\right)\sqrt{\mathrm{x}+\mathrm{2}}}{\mathrm{x}+\sqrt{\left(\mathrm{x}+\mathrm{2}\right)^{\mathrm{2}} }}\:\leqslant\:\mathrm{0} \\ $$

Question Number 100378 Answers: 1 Comments: 1

Question Number 100362 Answers: 3 Comments: 0

∫_0 ^1 ∫_0 ^1 e^(2x+y) dydx

$$\int_{\mathrm{0}} ^{\mathrm{1}} \int_{\mathrm{0}} ^{\mathrm{1}} {e}^{\mathrm{2}{x}+{y}} {dydx} \\ $$

Question Number 101075 Answers: 0 Comments: 1

lim_(x→0) ((arcsin (x^2 )−x^2 )/(x^4 tan^2 x)) = ?

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{arcsin}\:\left(\mathrm{x}^{\mathrm{2}} \right)−\mathrm{x}^{\mathrm{2}} }{\mathrm{x}^{\mathrm{4}} \:\mathrm{tan}\:^{\mathrm{2}} \mathrm{x}}\:=\:? \\ $$

Question Number 100344 Answers: 2 Comments: 0

solve y′′+y = sin x

$$\mathrm{solve}\:\mathrm{y}''+\mathrm{y}\:=\:\mathrm{sin}\:\mathrm{x} \\ $$

Question Number 100339 Answers: 1 Comments: 0

Suppose 1 ,2 ,4 are the roots of the equation x^4 +ax^2 +bx−c = 0 . What is the value of c ?

$$\mathrm{Suppose}\:\mathrm{1}\:,\mathrm{2}\:,\mathrm{4}\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$${x}^{\mathrm{4}} \:+{ax}^{\mathrm{2}} +{bx}−{c}\:=\:\mathrm{0}\:.\:{W}\mathrm{hat}\:\mathrm{is}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of} \\ $$$${c}\:?\: \\ $$

Question Number 100337 Answers: 2 Comments: 0

Solve: (1 + x^2 )y′′ − 4xy′ + 6y = 0

$$\mathrm{Solve}:\:\:\:\left(\mathrm{1}\:+\:\:\mathrm{x}^{\mathrm{2}} \right)\mathrm{y}''\:\:−\:\:\mathrm{4xy}'\:\:+\:\:\mathrm{6y}\:\:\:=\:\:\mathrm{0} \\ $$

Question Number 100330 Answers: 0 Comments: 1

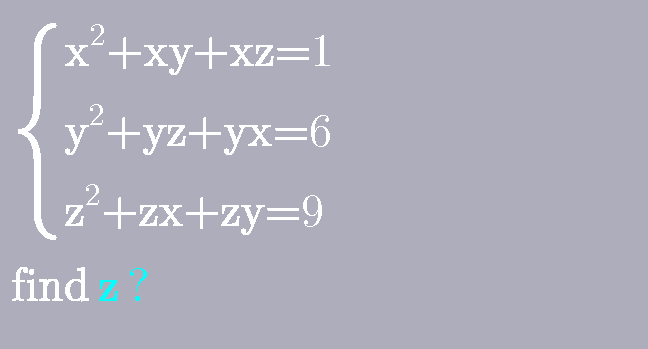
Question Number 100327 Answers: 1 Comments: 1

Solve x^2 y′′−3xy′−5y=0

$$\mathcal{S}\mathrm{olve}\:\mathrm{x}^{\mathrm{2}} \mathrm{y}''−\mathrm{3xy}'−\mathrm{5y}=\mathrm{0} \\ $$

Question Number 100323 Answers: 1 Comments: 1

Solve x^2 y′′+2xy′−2y=0

$$\mathbb{S}\mathrm{olve}\:\mathrm{x}^{\mathrm{2}} \mathrm{y}''+\mathrm{2xy}'−\mathrm{2y}=\mathrm{0} \\ $$

Question Number 100320 Answers: 1 Comments: 0

Evaluate ∫∫_s F^→ .n^ dS where F^→ =4xi^ −2y^2 j^ +z^2 k^ and S is the surface of the cylinder bounded by x^2 +y^2 =4 ,z = 0 and z=3 .

$$\mathrm{Evaluate}\:\int\underset{\mathrm{s}} {\int}\:\overset{\rightarrow} {\mathrm{F}}.\hat {\mathrm{n}}\:\mathrm{dS}\:\mathrm{where}\:\overset{\rightarrow} {\mathrm{F}}=\mathrm{4x}\hat {\mathrm{i}}\:−\mathrm{2y}^{\mathrm{2}} \hat {\mathrm{j}}\:+\mathrm{z}^{\mathrm{2}} \hat {\mathrm{k}}\: \\ $$$$\mathrm{and}\:\mathrm{S}\:\mathrm{is}\:\mathrm{the}\:\mathrm{surface}\:\mathrm{of}\:\mathrm{the}\:\mathrm{cylinder} \\ $$$$\mathrm{bounded}\:\mathrm{by}\:\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} =\mathrm{4}\:,\mathrm{z}\:=\:\mathrm{0}\:\mathrm{and}\:\mathrm{z}=\mathrm{3}\:. \\ $$

Question Number 100317 Answers: 2 Comments: 0

please help me to solve this! { ((xln3−e^(3yln3) =0)),((lnx−2lny=1)) :}

$$\boldsymbol{{please}}\:\boldsymbol{{help}}\:\boldsymbol{{me}}\:\boldsymbol{{to}}\:\boldsymbol{{solve}}\:\boldsymbol{{this}}! \\ $$$$ \\ $$$$\:\:\:\:\:\begin{cases}{\boldsymbol{{xln}}\mathrm{3}−\boldsymbol{{e}}^{\mathrm{3}\boldsymbol{{yln}}\mathrm{3}} =\mathrm{0}}\\{\boldsymbol{{lnx}}−\mathrm{2}\boldsymbol{{lny}}=\mathrm{1}}\end{cases} \\ $$$$ \\ $$

Question Number 100311 Answers: 1 Comments: 0

Question Number 100310 Answers: 0 Comments: 18

Version 2.085 is now available on playstore. Please update.

$$\mathrm{Version}\:\mathrm{2}.\mathrm{085}\:\mathrm{is}\:\mathrm{now}\:\mathrm{available}\:\mathrm{on} \\ $$$$\mathrm{playstore}.\:\mathrm{Please}\:\mathrm{update}. \\ $$

Question Number 100297 Answers: 1 Comments: 0

calulate using Riemann sums tbe limit of this sequence Σ_(k=n) ^(2n) sin ((π/k))

$${calulate}\:{using}\:{Riemann}\:{sums} \\ $$$${tbe}\:{limit}\:{of}\:{this}\:{sequence} \\ $$$$\:\:\underset{{k}={n}} {\overset{\mathrm{2}{n}} {\sum}}\mathrm{sin}\:\left(\frac{\pi}{{k}}\right) \\ $$

Question Number 100296 Answers: 1 Comments: 0

Given matrix A = [((3 1 4)),((1 2 5)),((0 2 6)) ] find: adj(adj A) ?

$$\mathcal{G}\mathrm{iven}\:\mathrm{matrix}\:\mathrm{A}\:=\:\begin{bmatrix}{\mathrm{3}\:\:\:\:\:\mathrm{1}\:\:\:\:\:\mathrm{4}}\\{\mathrm{1}\:\:\:\:\:\mathrm{2}\:\:\:\:\:\:\mathrm{5}}\\{\mathrm{0}\:\:\:\:\:\mathrm{2}\:\:\:\:\:\mathrm{6}}\end{bmatrix} \\ $$$$\mathrm{find}:\:\:\mathrm{adj}\left(\mathrm{adj}\:\mathrm{A}\right)\:? \\ $$

Question Number 100295 Answers: 0 Comments: 0

a relation R is defined on the set of real numbers by xRy if and only if x−y is a multiple of 3. show that R is transitive

$${a}\:{relation}\:{R}\:{is}\:{defined}\:{on}\:{the}\:{set}\:{of}\:{real} \\ $$$${numbers}\:{by}\:{xRy}\:{if}\:{and}\:{only}\:{if}\:{x}−{y}\:{is}\:{a}\: \\ $$$${multiple}\:{of}\:\mathrm{3}.\:{show}\:{that}\:{R}\:{is}\:{transitive} \\ $$

Question Number 100370 Answers: 1 Comments: 2

Find the maximum value of f(x) = (3/(2cosh (ln x) + 3))

$$\mathrm{Find}\:\mathrm{the}\:\mathrm{maximum}\:\mathrm{value}\:\mathrm{of}\:\:{f}\left({x}\right)\:=\:\frac{\mathrm{3}}{\mathrm{2cosh}\:\left(\mathrm{ln}\:{x}\right)\:+\:\mathrm{3}} \\ $$

Question Number 100369 Answers: 1 Comments: 0

aεZ 0<b<1 a+b=(√(20)) b^2 +4b+8(√5)=?

$${a}\epsilon\mathbb{Z}\:\:\:\mathrm{0}<{b}<\mathrm{1}\:\:\:\: \\ $$$${a}+{b}=\sqrt{\mathrm{20}} \\ $$$${b}^{\mathrm{2}} +\mathrm{4}{b}+\mathrm{8}\sqrt{\mathrm{5}}=? \\ $$

Question Number 100368 Answers: 1 Comments: 0

lim_(n→∞) ∫_(−∞) ^∞ cos (x^n ) dx =? where n=2k, k∈N, k≠0

$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\int_{−\infty} ^{\infty} \mathrm{cos}\:\left({x}^{{n}} \right)\:{dx}\:=? \\ $$$${where}\:{n}=\mathrm{2}{k},\:{k}\in\mathbb{N},\:{k}\neq\mathrm{0} \\ $$

Question Number 100289 Answers: 0 Comments: 0

lim_(n→∞) Σ_(k=n) ^(2n) sin((π/k)) Riemann′s integral may help

$$\underset{\mathrm{n}\rightarrow\infty} {\mathrm{lim}}\underset{\mathrm{k}=\mathrm{n}} {\overset{\mathrm{2n}} {\sum}}\mathrm{sin}\left(\frac{\pi}{\mathrm{k}}\right) \\ $$$${Riemann}'{s}\:{integral}\:{may}\:{help} \\ $$

Question Number 100285 Answers: 1 Comments: 0

Question Number 100290 Answers: 0 Comments: 1

Question Number 100270 Answers: 2 Comments: 0

Question Number 100302 Answers: 0 Comments: 2

(√(3^(−(1/2)) +1)) = ((√(a+1))/3^(−(1/4)) ) . find a ?

$$\sqrt{\mathrm{3}^{−\frac{\mathrm{1}}{\mathrm{2}}} +\mathrm{1}}\:=\:\frac{\sqrt{\mathrm{a}+\mathrm{1}}}{\mathrm{3}^{−\frac{\mathrm{1}}{\mathrm{4}}} }\:.\:\mathrm{find}\:\mathrm{a}\:? \\ $$

Pg 1151 Pg 1152 Pg 1153 Pg 1154 Pg 1155 Pg 1156 Pg 1157 Pg 1158 Pg 1159 Pg 1160

Contact: info@tinkutara.com