Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1153

Question Number 100189 Answers: 1 Comments: 0

∫tan^i xdx

$$\int{tan}^{{i}} {xdx} \\ $$

Question Number 100186 Answers: 0 Comments: 0

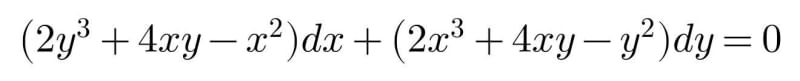
Question Number 100184 Answers: 1 Comments: 0

((ydx + xdy)/(1−x^2 y^2 )) + xdx = 0

$$\frac{\mathrm{ydx}\:+\:\mathrm{xdy}}{\mathrm{1}−\mathrm{x}^{\mathrm{2}} \mathrm{y}^{\mathrm{2}} }\:+\:\mathrm{xdx}\:=\:\mathrm{0} \\ $$

Question Number 100179 Answers: 2 Comments: 0

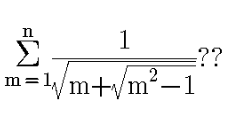
Question Number 100178 Answers: 2 Comments: 0

what is the number of ordered pairs of positif integers (x,y) that satisfy x^2 +y^2 −xy=37

$$\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{ordered}\:\mathrm{pairs}\:\mathrm{of}\:\mathrm{positif}\: \\ $$$$\mathrm{integers}\:\left(\mathrm{x},\mathrm{y}\right)\:\mathrm{that}\:\mathrm{satisfy}\:\mathrm{x}^{\mathrm{2}} +\mathrm{y}^{\mathrm{2}} −\mathrm{xy}=\mathrm{37} \\ $$

Question Number 100173 Answers: 0 Comments: 2

Updated apk with the following changes (with fixes for all reported issues so far) is available at www.tinkutara.com. • Review a post • copy all to buffer • Ability to draw diagrams

$$\mathrm{Updated}\:\mathrm{apk}\:\mathrm{with}\:\mathrm{the}\:\mathrm{following} \\ $$$$\mathrm{changes}\:\left(\mathrm{with}\:\mathrm{fixes}\:\mathrm{for}\:\mathrm{all}\:\mathrm{reported}\right. \\ $$$$\left.\mathrm{issues}\:\mathrm{so}\:\mathrm{far}\right)\:\mathrm{is}\:\mathrm{available}\:\mathrm{at} \\ $$$$\mathrm{www}.\mathrm{tinkutara}.\mathrm{com}. \\ $$$$\bullet\:\mathrm{Review}\:\mathrm{a}\:\mathrm{post} \\ $$$$\bullet\:\mathrm{copy}\:\mathrm{all}\:\mathrm{to}\:\mathrm{buffer} \\ $$$$\bullet\:\mathrm{Ability}\:\mathrm{to}\:\mathrm{draw}\:\mathrm{diagrams} \\ $$

Question Number 100343 Answers: 1 Comments: 3

l_(x→0) im ((f(x+1)^(1/x) )/(f(1))) f(1)=? help me

$$\underset{\mathrm{x}\rightarrow\mathrm{0}} {\mathrm{l}im}\:\frac{\mathrm{f}\left(\mathrm{x}+\mathrm{1}\right)^{\frac{\mathrm{1}}{\mathrm{x}}} }{\mathrm{f}\left(\mathrm{1}\right)}\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{f}\left(\mathrm{1}\right)=? \\ $$$$\mathrm{help}\:\mathrm{me} \\ $$

Question Number 100304 Answers: 0 Comments: 3

Question Number 100158 Answers: 1 Comments: 0

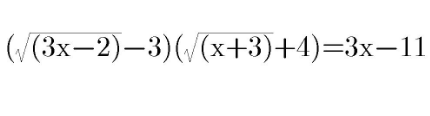
Question Number 100151 Answers: 0 Comments: 1

(√(lnx)) −ln(√x) =0 x=?

$$\sqrt{\mathrm{lnx}}\:−\mathrm{ln}\sqrt{\mathrm{x}}\:=\mathrm{0}\:\:\:\:\:\:\mathrm{x}=? \\ $$

Question Number 100150 Answers: 0 Comments: 3

x^(ln) −e^6 ∙x=0 x=? help me

$$\mathrm{x}^{\mathrm{ln}} −\mathrm{e}^{\mathrm{6}} \centerdot\mathrm{x}=\mathrm{0}\:\:\:\:\:\:\mathrm{x}=? \\ $$$$\mathrm{help}\:\mathrm{me} \\ $$

Question Number 100341 Answers: 1 Comments: 1

An open box with a square base is to be made out of a given quantity of a cardboard of area c^2 square units.show the maximum volume of the box (c^2 /(6(√3))) cubic units

$$\mathrm{An}\:\mathrm{open}\:\mathrm{box}\:\mathrm{with}\:\mathrm{a}\:\mathrm{square} \\ $$$$\mathrm{base}\:\mathrm{is}\:\mathrm{to}\:\mathrm{be}\:\mathrm{made}\:\mathrm{out} \\ $$$$\mathrm{of}\:\mathrm{a}\:\mathrm{given}\:\mathrm{quantity}\:\mathrm{of} \\ $$$$\mathrm{a}\:\mathrm{cardboard}\:\mathrm{of}\:\mathrm{area}\:\mathrm{c}^{\mathrm{2}} \\ $$$$\mathrm{square}\:\mathrm{units}.\mathrm{show}\:\mathrm{the} \\ $$$$\mathrm{maximum}\:\mathrm{volume}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{box}\:\frac{\mathrm{c}^{\mathrm{2}} }{\mathrm{6}\sqrt{\mathrm{3}}}\:\:\mathrm{cubic}\:\mathrm{units} \\ $$$$ \\ $$

Question Number 100146 Answers: 1 Comments: 3

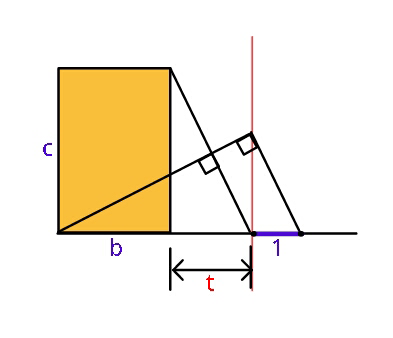
Question Number 100134 Answers: 1 Comments: 2

lim_(x→(π/2)) ((4sin x−(√(6(√(sin x))+10)))/((π/2)−x)) ?

$$\underset{{x}\rightarrow\frac{\pi}{\mathrm{2}}} {\mathrm{lim}}\:\frac{\mathrm{4sin}\:\mathrm{x}−\sqrt{\mathrm{6}\sqrt{\mathrm{sin}\:\mathrm{x}}+\mathrm{10}}}{\frac{\pi}{\mathrm{2}}−\mathrm{x}}\:? \\ $$

Question Number 100133 Answers: 1 Comments: 0

If sin^(−1) θ+sin^(−1) β=π θ+β−(2/(θ^2 +β^2 )) = ?

$$\mathrm{If}\:\mathrm{sin}^{−\mathrm{1}} \theta+\mathrm{sin}^{−\mathrm{1}} \beta=\pi\: \\ $$$$\theta+\beta−\frac{\mathrm{2}}{\theta^{\mathrm{2}} +\beta^{\mathrm{2}} }\:=\:? \\ $$

Question Number 100131 Answers: 2 Comments: 3

If 3sin x+4cos x=5 then sin x=?

$$\mathrm{If}\:\mathrm{3sin}\:\mathrm{x}+\mathrm{4cos}\:\mathrm{x}=\mathrm{5}\:\mathrm{then}\:\mathrm{sin}\:\mathrm{x}=? \\ $$

Question Number 100130 Answers: 1 Comments: 0

how many integer number satisfy the equation ((sin x−∣x+2∣)/(x^2 −4x−5)) ≥ 0

$$\mathrm{how}\:\mathrm{many}\:\mathrm{integer}\:\mathrm{number}\: \\ $$$$\mathrm{satisfy}\:\mathrm{the}\:\mathrm{equation}\: \\ $$$$\frac{\mathrm{sin}\:\mathrm{x}−\mid\mathrm{x}+\mathrm{2}\mid}{\mathrm{x}^{\mathrm{2}} −\mathrm{4x}−\mathrm{5}}\:\geqslant\:\mathrm{0}\: \\ $$

Question Number 100114 Answers: 1 Comments: 0

Question Number 100106 Answers: 0 Comments: 6

APK with the option to review a post in forum is available. Review is essentially same as comment however an editable original post is included in editor. While review post with images image is used as background and you can use shapes like ovals circle etc to highlight. This should remove to manually count lines to highlight corrections. Once in progress enhancements are this version app will updated to playstore.

$$\mathrm{APK}\:\mathrm{with}\:\mathrm{the}\:\mathrm{option}\:\mathrm{to}\:\mathrm{review} \\ $$$$\mathrm{a}\:\mathrm{post}\:\mathrm{in}\:\mathrm{forum}\:\mathrm{is}\:\mathrm{available}. \\ $$$$\mathrm{Review}\:\mathrm{is}\:\mathrm{essentially}\:\mathrm{same}\:\mathrm{as}\:\mathrm{comment} \\ $$$$\mathrm{however}\:\mathrm{an}\:\mathrm{editable}\:\mathrm{original} \\ $$$$\mathrm{post}\:\mathrm{is}\:\mathrm{included}\:\mathrm{in}\:\mathrm{editor}. \\ $$$$\mathrm{While}\:\mathrm{review}\:\mathrm{post}\:\mathrm{with}\:\mathrm{images} \\ $$$$\mathrm{image}\:\mathrm{is}\:\mathrm{used}\:\mathrm{as}\:\mathrm{background}\:\mathrm{and} \\ $$$$\mathrm{you}\:\mathrm{can}\:\mathrm{use}\:\mathrm{shapes}\:\mathrm{like}\:\mathrm{ovals} \\ $$$$\mathrm{circle}\:\mathrm{etc}\:\mathrm{to}\:\mathrm{highlight}. \\ $$$$\mathrm{This}\:\mathrm{should}\:\mathrm{remove}\:\mathrm{to}\:\mathrm{manually} \\ $$$$\mathrm{count}\:\mathrm{lines}\:\mathrm{to}\:\mathrm{highlight}\:\mathrm{corrections}. \\ $$$$\mathrm{Once}\:\mathrm{in}\:\mathrm{progress}\:\mathrm{enhancements}\:\mathrm{are} \\ $$$$\mathrm{this}\:\mathrm{version}\:\mathrm{app}\:\mathrm{will}\:\mathrm{updated}\:\mathrm{to}\:\mathrm{playstore}. \\ $$

Question Number 100097 Answers: 0 Comments: 1

∫(tanx)^e^(iπ) dx

$$\int\left({tanx}\right)^{{e}^{{i}\pi} } {dx} \\ $$

Question Number 100089 Answers: 0 Comments: 0

calculate A_n =∫_0 ^(π/2) ((sin^n (x))/(sin(nx)))dx

$$\:\mathrm{calculate}\:\mathrm{A}_{\mathrm{n}} =\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \:\frac{\mathrm{sin}^{\mathrm{n}} \left(\mathrm{x}\right)}{\mathrm{sin}\left(\mathrm{nx}\right)}\mathrm{dx}\: \\ $$

Question Number 100088 Answers: 1 Comments: 1

calculate ∫ ((cosx)/(cos(3x)))dx

$$\mathrm{calculate}\:\int\:\frac{\mathrm{cosx}}{\mathrm{cos}\left(\mathrm{3x}\right)}\mathrm{dx} \\ $$

Question Number 100087 Answers: 1 Comments: 1

use beta function to calculate ∫_0 ^π sin^3 x(2+cosx)^6 dx

$$\mathrm{use}\:\mathrm{beta}\:\mathrm{function}\:\mathrm{to}\:\mathrm{calculate}\:\int_{\mathrm{0}} ^{\pi} \:\mathrm{sin}^{\mathrm{3}} \mathrm{x}\left(\mathrm{2}+\mathrm{cosx}\right)^{\mathrm{6}} \:\mathrm{dx} \\ $$

Question Number 100074 Answers: 1 Comments: 0

Question Number 100068 Answers: 2 Comments: 0

hello all i have some questions? 1)what is the riemann hypothesis? 2)how did they determine the distance to the sun? 3)how did we measure the speed of light?

$${hello}\:{all}\:{i}\:{have}\:{some}\:{questions}? \\ $$$$ \\ $$$$\left.\mathrm{1}\right){what}\:{is}\:{the}\:{riemann}\:{hypothesis}? \\ $$$$ \\ $$$$\left.\mathrm{2}\right){how}\:{did}\:{they}\:{determine}\:{the}\:{distance} \\ $$$${to}\:{the}\:{sun}? \\ $$$$ \\ $$$$\left.\mathrm{3}\right){how}\:{did}\:{we}\:{measure}\:{the}\:{speed}\:{of}\:{light}? \\ $$$$ \\ $$

Question Number 100065 Answers: 8 Comments: 0

Pg 1148 Pg 1149 Pg 1150 Pg 1151 Pg 1152 Pg 1153 Pg 1154 Pg 1155 Pg 1156 Pg 1157

Contact: info@tinkutara.com