Question and Answers Forum

what is the absolute speed of A that moves via y = (1/(1+x)) (upper part) when it crosses y −axis meanwhile B moves at constant speed of 1 the x−axis ?

$${what}\:{is}\:{the}\:{absolute}\:{speed}\:{of}\:{A}\:{that} \\ $$$${moves}\:{via}\:{y}\:=\:\frac{\mathrm{1}}{\mathrm{1}+{x}}\:\left({upper}\:{part}\right)\:{when} \\ $$$${it}\:{crosses}\:{y}\:−{axis}\:{meanwhile}\:{B} \\ $$$${moves}\:{at}\:{constant}\:{speed}\:{of}\:\mathrm{1}\:{the} \\ $$$${x}−{axis}\:? \\ $$

Question Number 102020 Answers: 2 Comments: 0

lim_(x→0) (tan((π/4)−x))^(1/x)

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\left({tan}\left(\frac{\pi}{\mathrm{4}}−{x}\right)\right)^{\frac{\mathrm{1}}{{x}}} \\ $$

Question Number 102015 Answers: 0 Comments: 0

how many rotational symetry triangle isosceles ? 1 or 0??

$${how}\:{many}\:{rotational}\:{symetry}\:\:{triangle} \\ $$$${isosceles}\:? \\ $$$$\mathrm{1}\:{or}\:\mathrm{0}?? \\ $$

Question Number 102002 Answers: 0 Comments: 6

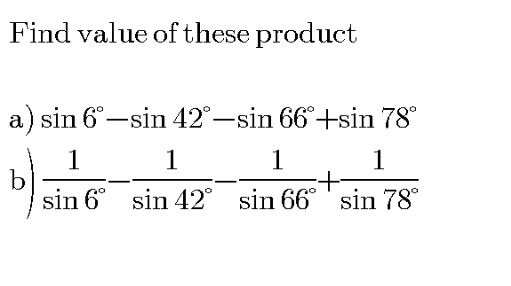
Question Number 102001 Answers: 2 Comments: 0

evaluate ∫cos^3 xsin^3 xdx.

$${evaluate}\:\int\mathrm{cos}\:^{\mathrm{3}} {x}\mathrm{sin}\:^{\mathrm{3}} {xdx}. \\ $$

Question Number 101998 Answers: 0 Comments: 0

1)solve inside C x^n −e^(−inα) =0 (α real) 2) let P(x) =x^n −e^(−inα) factorize P(x)inside C[x] 2) decompose inside C(x) thefraction F =(1/(P(x))) and deyermine ∫ F(x)dx

$$\left.\mathrm{1}\right)\mathrm{solve}\:\mathrm{inside}\:\mathrm{C}\:\:\mathrm{x}^{\mathrm{n}} −\mathrm{e}^{−\mathrm{in}\alpha} \:=\mathrm{0}\:\:\:\:\:\left(\alpha\:\mathrm{real}\right) \\ $$$$\left.\mathrm{2}\right)\:\mathrm{let}\:\mathrm{P}\left(\mathrm{x}\right)\:=\mathrm{x}^{\mathrm{n}} −\mathrm{e}^{−\mathrm{in}\alpha} \:\:\mathrm{factorize}\:\mathrm{P}\left(\mathrm{x}\right)\mathrm{inside}\:\mathrm{C}\left[\mathrm{x}\right] \\ $$$$\left.\mathrm{2}\right)\:\mathrm{decompose}\:\mathrm{inside}\:\mathrm{C}\left(\mathrm{x}\right)\:\mathrm{thefraction}\:\mathrm{F}\:=\frac{\mathrm{1}}{\mathrm{P}\left(\mathrm{x}\right)} \\ $$$$\mathrm{and}\:\mathrm{deyermine}\:\int\:\mathrm{F}\left(\mathrm{x}\right)\mathrm{dx} \\ $$

Question Number 101994 Answers: 1 Comments: 1

Question Number 101985 Answers: 1 Comments: 0

∫ ((3x^5 − x^4 + 9x^3 − 12x^2 − 2x + 1)/((x^3 − 1)^2 )) dx

$$\int\:\frac{\mathrm{3x}^{\mathrm{5}} \:−\:\mathrm{x}^{\mathrm{4}} \:+\:\mathrm{9x}^{\mathrm{3}} \:−\:\mathrm{12x}^{\mathrm{2}} \:−\:\mathrm{2x}\:\:+\:\:\mathrm{1}}{\left(\mathrm{x}^{\mathrm{3}} \:−\:\mathrm{1}\right)^{\mathrm{2}} }\:\mathrm{dx} \\ $$

Question Number 102058 Answers: 1 Comments: 0

∫_0 ^1 ((sin(logx))/(logx))dx

$$\int_{\mathrm{0}} ^{\mathrm{1}} \frac{{sin}\left({logx}\right)}{{logx}}{dx} \\ $$

Question Number 102183 Answers: 4 Comments: 0

∫ ((cos θ)/(sin θ−cos θ)) dθ ?

$$\int\:\frac{\mathrm{cos}\:\theta}{\mathrm{sin}\:\theta−\mathrm{cos}\:\theta}\:{d}\theta\:? \\ $$

Question Number 101981 Answers: 3 Comments: 0

lim_(x→0) (((tanx)/x))^(1/x^2 )

$${li}\underset{{x}\rightarrow\mathrm{0}} {{m}}\left(\frac{{tanx}}{{x}}\right)^{\frac{\mathrm{1}}{{x}^{\mathrm{2}} }} \\ $$

Question Number 101977 Answers: 0 Comments: 0

Given 2 functions, f and g, n-times derivable within the open interval, R and verify the property f(x_0 )=f^((k)) (x_0 )=0 , g(x_0 )=g^((k)) (x_0 )=0 , ∀k∈{1,2,...,n−1} Show that lim_(x→x_0 ) ((f(x))/(g(x)))=((f^((n)) (x_0 ))/(g^((n)) (x_0 )))

$$\mathrm{Given}\:\mathrm{2}\:\mathrm{functions},\:\mathrm{f}\:\mathrm{and}\:\mathrm{g},\:\mathrm{n}-\mathrm{times}\:\mathrm{derivable}\:\mathrm{within} \\ $$$$\mathrm{the}\:\mathrm{open}\:\mathrm{interval},\:\mathbb{R}\:\mathrm{and}\:\mathrm{verify}\:\mathrm{the}\:\mathrm{property} \\ $$$$\mathrm{f}\left(\mathrm{x}_{\mathrm{0}} \right)=\mathrm{f}^{\left(\mathrm{k}\right)} \left(\mathrm{x}_{\mathrm{0}} \right)=\mathrm{0}\:,\:\mathrm{g}\left(\mathrm{x}_{\mathrm{0}} \right)=\mathrm{g}^{\left(\mathrm{k}\right)} \left(\mathrm{x}_{\mathrm{0}} \right)=\mathrm{0}\:,\:\forall\mathrm{k}\in\left\{\mathrm{1},\mathrm{2},...,\mathrm{n}−\mathrm{1}\right\} \\ $$$$\mathrm{Show}\:\mathrm{that}\:\underset{\mathrm{x}\rightarrow\mathrm{x}_{\mathrm{0}} } {\mathrm{lim}}\frac{\mathrm{f}\left(\mathrm{x}\right)}{\mathrm{g}\left(\mathrm{x}\right)}=\frac{\mathrm{f}^{\left(\mathrm{n}\right)} \left(\mathrm{x}_{\mathrm{0}} \right)}{\mathrm{g}^{\left(\mathrm{n}\right)} \left(\mathrm{x}_{\mathrm{0}} \right)} \\ $$

Question Number 101974 Answers: 2 Comments: 1

Question Number 101971 Answers: 0 Comments: 2

Question Number 101970 Answers: 1 Comments: 0

∫_0 ^∞ ((Cos(ax))/(x^2 +b^2 )) dx

$$\int_{\mathrm{0}} ^{\infty} \:\frac{{Cos}\left({ax}\right)}{{x}^{\mathrm{2}} +{b}^{\mathrm{2}} }\:{dx} \\ $$

Question Number 101961 Answers: 0 Comments: 4

Question Number 101959 Answers: 1 Comments: 0

Starting from y=(4/(√π))t^((3/2) ) ∫_0 ^∞ x^3 e^(−tx^2 ) dx find (π/8)=?

$$ \\ $$$$ \\ $$$${Starting}\:{from} \\ $$$$\:\:\:\:\:\:\:\:{y}=\frac{\mathrm{4}}{\sqrt{\pi}}{t}^{\frac{\mathrm{3}}{\mathrm{2}}\:} \int_{\mathrm{0}} ^{\infty} {x}^{\mathrm{3}} {e}^{−{tx}^{\mathrm{2}} } {dx} \\ $$$${find}\:\:\:\frac{\pi}{\mathrm{8}}=? \\ $$

Question Number 101951 Answers: 0 Comments: 3

Question Number 101940 Answers: 1 Comments: 0

lim_(n→∞ ) (1/n^2 )(ne^((−1)/n^2 ) +ne^((−4)/n^2 ) +.....∞)

$$\underset{{n}\rightarrow\infty\:} {\mathrm{lim}}\frac{\mathrm{1}}{{n}^{\mathrm{2}} }\left({ne}^{\frac{−\mathrm{1}}{{n}^{\mathrm{2}} }} +{ne}^{\frac{−\mathrm{4}}{{n}^{\mathrm{2}} }} +.....\infty\right) \\ $$

Question Number 101937 Answers: 0 Comments: 0

Given a circle with the center at the point O and the radius of the length R.From a point A outside so that AO=2R,drawing two tangents AB and AC to the circle (B and C are the tangency points).Take a arbitrary point M on smaller arc BC (M differ from B and C) The tangent pass M cuts AB and AC at Pand Q respectively.The segments OP and OQ cuts BC at D and E respectively. i)Prove that PQ=2DE ii)Define the position of M such the area of the triangle ODE is smallest and expression it by R

Pg 1144 Pg 1145 Pg 1146 Pg 1147 Pg 1148 Pg 1149 Pg 1150 Pg 1151 Pg 1152 Pg 1153

Contact: info@tinkutara.com