
AllQuestion and Answers: Page 1142
Question Number 101851 Answers: 1 Comments: 0

Question Number 101568 Answers: 1 Comments: 0
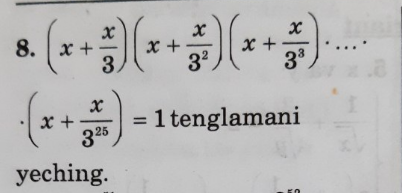
Question Number 101563 Answers: 2 Comments: 0

Question Number 101558 Answers: 1 Comments: 0
Question Number 101555 Answers: 1 Comments: 0

Question Number 101554 Answers: 3 Comments: 0
$$\frac{\mathrm{1}}{\mathrm{cos80}}−\frac{\sqrt{\mathrm{3}}}{\mathrm{sin80}}=? \\ $$
Question Number 101553 Answers: 1 Comments: 0
Question Number 101551 Answers: 2 Comments: 0

Question Number 101546 Answers: 4 Comments: 1
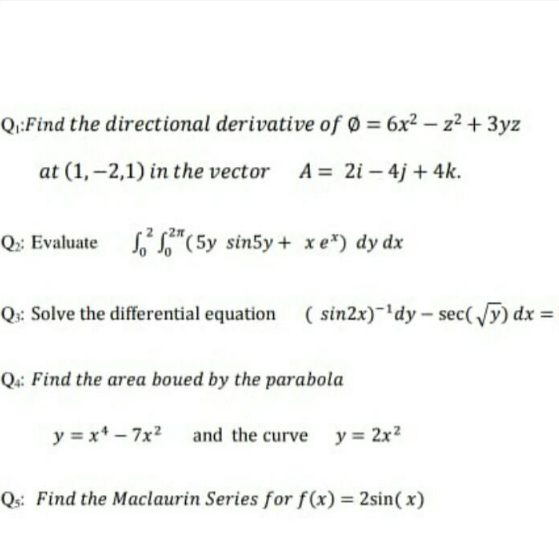
Question Number 101531 Answers: 1 Comments: 0
Question Number 105345 Answers: 1 Comments: 0
Question Number 101522 Answers: 1 Comments: 1
Question Number 101523 Answers: 0 Comments: 1
Question Number 101514 Answers: 2 Comments: 4

Question Number 101510 Answers: 0 Comments: 1
Question Number 101500 Answers: 2 Comments: 1
Question Number 101498 Answers: 1 Comments: 3
Question Number 101493 Answers: 1 Comments: 0
Question Number 101491 Answers: 0 Comments: 2

Question Number 101489 Answers: 1 Comments: 0

Question Number 101486 Answers: 0 Comments: 0

Question Number 101476 Answers: 1 Comments: 0
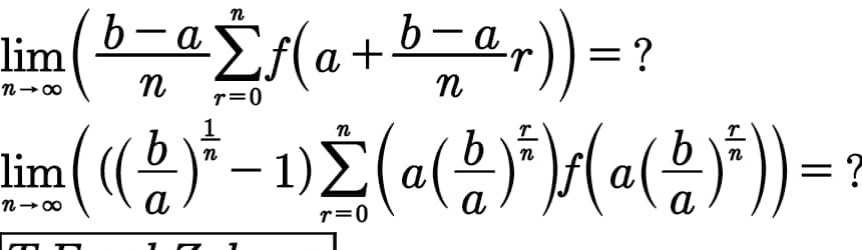
Question Number 101474 Answers: 1 Comments: 0

Question Number 101473 Answers: 1 Comments: 0

Question Number 101471 Answers: 0 Comments: 4
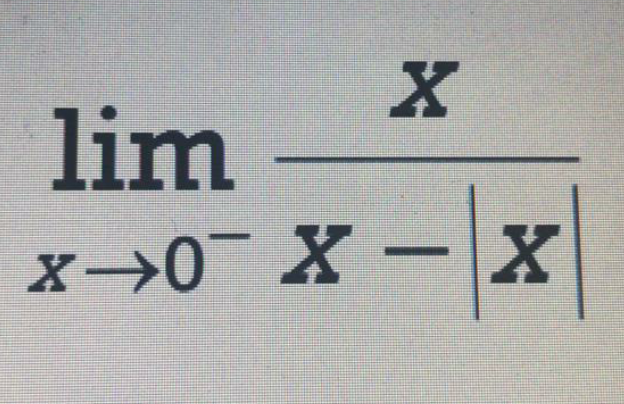
Question Number 101461 Answers: 1 Comments: 0
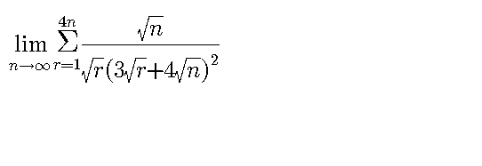
Pg 1137 Pg 1138 Pg 1139 Pg 1140 Pg 1141 Pg 1142 Pg 1143 Pg 1144 Pg 1145 Pg 1146
