
AllQuestion and Answers: Page 1129
Question Number 103691 Answers: 1 Comments: 0
Question Number 103686 Answers: 1 Comments: 0
$${x}^{{x}^{\mathrm{6}} } =\sqrt{\mathrm{2}}\:^{\sqrt{\mathrm{2}}} \:{x}=? \\ $$
Question Number 103685 Answers: 1 Comments: 0

Question Number 103683 Answers: 1 Comments: 1
Question Number 103673 Answers: 1 Comments: 0
Question Number 103672 Answers: 1 Comments: 3
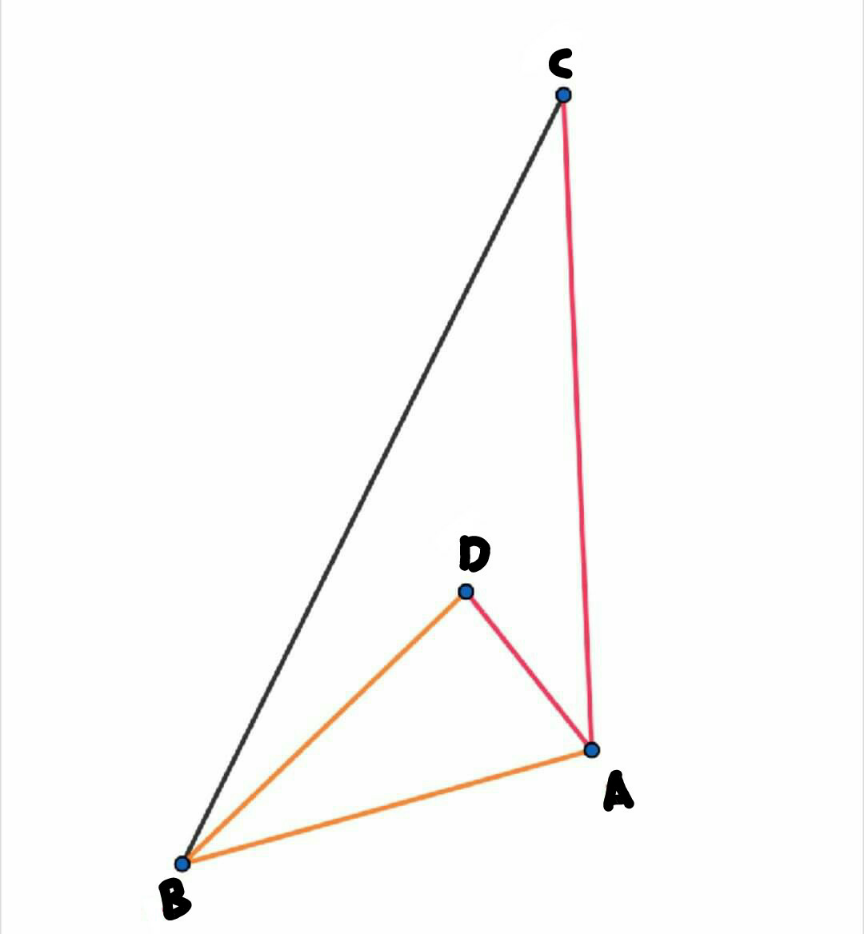
Question Number 103670 Answers: 4 Comments: 0
Question Number 103669 Answers: 2 Comments: 0
Question Number 103665 Answers: 0 Comments: 0
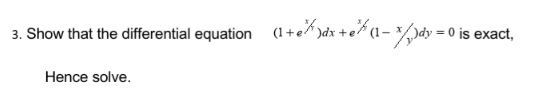
Question Number 103664 Answers: 1 Comments: 0

Question Number 103659 Answers: 2 Comments: 1
Question Number 103652 Answers: 0 Comments: 1

Question Number 103649 Answers: 0 Comments: 0

Question Number 103648 Answers: 2 Comments: 0
Question Number 103647 Answers: 0 Comments: 0

Question Number 103644 Answers: 1 Comments: 1

Question Number 103643 Answers: 4 Comments: 0
Question Number 103633 Answers: 2 Comments: 1
Question Number 103624 Answers: 0 Comments: 0

Question Number 103623 Answers: 2 Comments: 0
Question Number 103622 Answers: 2 Comments: 0
Question Number 103620 Answers: 1 Comments: 0
Question Number 103607 Answers: 1 Comments: 0
Question Number 103606 Answers: 3 Comments: 1
Question Number 103603 Answers: 0 Comments: 1

Question Number 103597 Answers: 0 Comments: 2
Pg 1124 Pg 1125 Pg 1126 Pg 1127 Pg 1128 Pg 1129 Pg 1130 Pg 1131 Pg 1132 Pg 1133
