Question and Answers Forum

AllQuestion and Answers: Page 1082

Question Number 108901 Answers: 1 Comments: 0

f(x)=x^2 (1+x)^3 Find f′′(1).

$${f}\left({x}\right)={x}^{\mathrm{2}} \left(\mathrm{1}+{x}\right)^{\mathrm{3}} \\ $$$$\mathrm{Find}\:{f}''\left(\mathrm{1}\right).\: \\ $$

Question Number 108893 Answers: 6 Comments: 0

(1)∫ (x^4 /(1−x^2 )) dx (2)∫_(−3) ^5 (√(∣x∣^3 )) dx (3) ∫_0 ^(π^2 /4) sin (√x) dx (4) ∫_(−∞) ^∞ e^(−2x^2 −5x−3) dx (5) x^3 y′′′−2x^2 y′′−2xy′+8y=0 (6)(x^4 +y^4 )dx+2x^3 y dy = 0 (7) (2(√(xy))−y)dx−xdy = 0

$$\left(\mathrm{1}\right)\int\:\frac{{x}^{\mathrm{4}} }{\mathrm{1}−{x}^{\mathrm{2}} }\:{dx}\: \\ $$$$\left(\mathrm{2}\right)\underset{−\mathrm{3}} {\overset{\mathrm{5}} {\int}}\sqrt{\mid{x}\mid^{\mathrm{3}} }\:{dx}\: \\ $$$$\left(\mathrm{3}\right)\:\underset{\mathrm{0}} {\overset{\frac{\pi^{\mathrm{2}} }{\mathrm{4}}} {\int}}\:\mathrm{sin}\:\sqrt{{x}}\:{dx}\: \\ $$$$\left(\mathrm{4}\right)\:\underset{−\infty} {\overset{\infty} {\int}}{e}^{−\mathrm{2}{x}^{\mathrm{2}} −\mathrm{5}{x}−\mathrm{3}} \:{dx}\: \\ $$$$\left(\mathrm{5}\right)\:{x}^{\mathrm{3}} {y}'''−\mathrm{2}{x}^{\mathrm{2}} {y}''−\mathrm{2}{xy}'+\mathrm{8}{y}=\mathrm{0} \\ $$$$\left(\mathrm{6}\right)\left({x}^{\mathrm{4}} +{y}^{\mathrm{4}} \right){dx}+\mathrm{2}{x}^{\mathrm{3}} {y}\:{dy}\:=\:\mathrm{0} \\ $$$$\left(\mathrm{7}\right)\:\left(\mathrm{2}\sqrt{{xy}}−{y}\right){dx}−{xdy}\:=\:\mathrm{0} \\ $$

Question Number 108891 Answers: 1 Comments: 0

((bobHans)/∦) ∫ (((x^2 −2) dx)/((x^4 +5x^2 +4) arc tan (((x^2 +2)/x))))

$$\:\:\:\frac{\boldsymbol{{bob}}\mathbb{H}{ans}}{\nparallel} \\ $$$$\int\:\frac{\left({x}^{\mathrm{2}} −\mathrm{2}\right)\:{dx}}{\left({x}^{\mathrm{4}} +\mathrm{5}{x}^{\mathrm{2}} +\mathrm{4}\right)\:\mathrm{arc}\:\mathrm{tan}\:\left(\frac{{x}^{\mathrm{2}} +\mathrm{2}}{{x}}\right)} \\ $$

Question Number 108888 Answers: 3 Comments: 0

((⋮BeMath⋮)/△) Suppose 2x^3 +3x^2 −14x−5= (Px+Q)(x+3)(x+1)+R for all value of x. Find the value of P,Q and R

$$\:\:\:\frac{\vdots\mathcal{B}{e}\mathcal{M}{ath}\vdots}{\bigtriangleup} \\ $$$${Suppose}\:\mathrm{2}{x}^{\mathrm{3}} +\mathrm{3}{x}^{\mathrm{2}} −\mathrm{14}{x}−\mathrm{5}=\:\left({Px}+{Q}\right)\left({x}+\mathrm{3}\right)\left({x}+\mathrm{1}\right)+{R}\:{for}\:{all} \\ $$$${value}\:{of}\:{x}.\:{Find}\:{the}\:{value}\:{of}\:{P},{Q}\:{and}\:{R}\: \\ $$

Question Number 108899 Answers: 0 Comments: 1

Question Number 108897 Answers: 0 Comments: 1

Question Number 108871 Answers: 0 Comments: 0

Question Number 108870 Answers: 2 Comments: 1

Question Number 108868 Answers: 2 Comments: 1

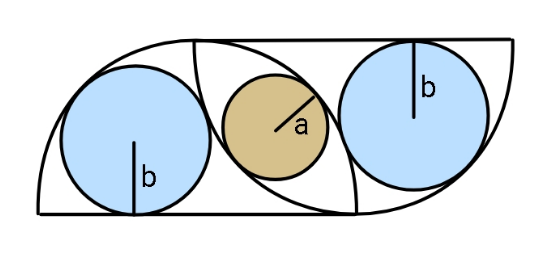
Question Number 108854 Answers: 1 Comments: 0

The sixth term of an A.P. is 2, its common difference is greater than one. Find the value of the common difference so that the product of the first, fourth and fifth terms is the greatest.

$$\mathrm{The}\:\mathrm{sixth}\:\mathrm{term}\:\mathrm{of}\:\mathrm{an}\:\mathrm{A}.\mathrm{P}.\:\mathrm{is}\:\mathrm{2},\:\mathrm{its} \\ $$$$\mathrm{common}\:\mathrm{difference}\:\mathrm{is}\:\mathrm{greater}\:\mathrm{than} \\ $$$$\mathrm{one}.\:\mathrm{Find}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{the}\:\mathrm{common} \\ $$$$\mathrm{difference}\:\mathrm{so}\:\mathrm{that}\:\mathrm{the}\:\mathrm{product}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{first},\:\mathrm{fourth}\:\mathrm{and}\:\mathrm{fifth}\:\mathrm{terms}\:\mathrm{is}\:\mathrm{the} \\ $$$$\mathrm{greatest}. \\ $$

Question Number 108864 Answers: 2 Comments: 1

Question Number 108842 Answers: 2 Comments: 3

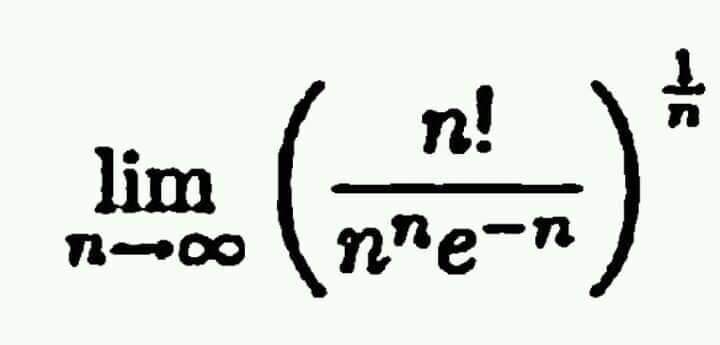
Question Number 108841 Answers: 1 Comments: 0

Question Number 108839 Answers: 0 Comments: 1

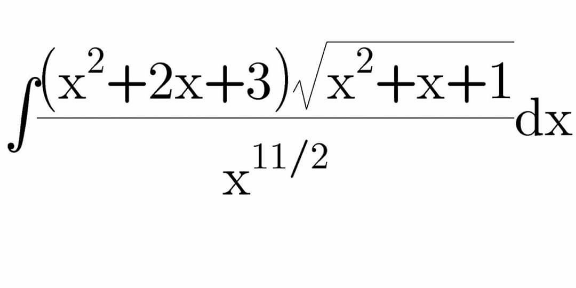
Question Number 108838 Answers: 0 Comments: 0

Question Number 108836 Answers: 1 Comments: 0

((bemath)/(⊂cooll⊃)) find the particular solution of y′′ + 4y = sin (2x)

$$\:\:\frac{\boldsymbol{{bemath}}}{\subset{cooll}\supset} \\ $$$${find}\:{the}\:{particular}\:{solution}\: \\ $$$${of}\:{y}''\:+\:\mathrm{4}{y}\:=\:\mathrm{sin}\:\left(\mathrm{2}{x}\right) \\ $$

Question Number 108825 Answers: 2 Comments: 1

Σ_(n=1) ^(10) (i^n +i^(n+1) )= ?

$$\underset{{n}=\mathrm{1}} {\overset{\mathrm{10}} {\sum}}\left({i}^{{n}} +{i}^{{n}+\mathrm{1}} \right)=\:? \\ $$

Question Number 108821 Answers: 1 Comments: 0

find ∫_0 ^∞ ((lnx)/((x^2 +1)^2 ))dx

$$\mathrm{find}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{lnx}}{\left(\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }\mathrm{dx} \\ $$

Question Number 108818 Answers: 1 Comments: 0

((Bobhans)/Δ) (1)Let n be a positive integer, and let x and y be positive real number such that x^n + y^n = 1 . Prove that (Σ_(k = 1) ^n ((1+x^(2k) )/(1+x^(4k) )) )(Σ_(k = 1) ^n ((1+y^(2k) )/(1+y^(4k) )) ) < (1/((1−x)(1−y))) (2) All the letters of the word ′EAMCOT ′ are arranged in different possible ways. The number of such arrangement in which no two vowels are adjacent to each other is ___

$$\:\:\frac{\boldsymbol{\mathcal{B}}{ob}\boldsymbol{{hans}}}{\Delta} \\ $$$$\left(\mathrm{1}\right){Let}\:{n}\:{be}\:{a}\:{positive}\:{integer},\:{and}\:{let}\:{x}\:{and}\:{y}\:{be}\:{positive}\:{real}\: \\ $$$${number}\:{such}\:{that}\:{x}^{{n}} \:+\:{y}^{{n}} \:=\:\mathrm{1}\:.\:{Prove}\:{that}\: \\ $$$$\left(\underset{{k}\:=\:\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}+{x}^{\mathrm{2}{k}} }{\mathrm{1}+{x}^{\mathrm{4}{k}} }\:\right)\left(\underset{{k}\:=\:\mathrm{1}} {\overset{{n}} {\sum}}\frac{\mathrm{1}+{y}^{\mathrm{2}{k}} }{\mathrm{1}+{y}^{\mathrm{4}{k}} }\:\right)\:<\:\frac{\mathrm{1}}{\left(\mathrm{1}−{x}\right)\left(\mathrm{1}−{y}\right)} \\ $$$$\left(\mathrm{2}\right)\:{All}\:{the}\:{letters}\:{of}\:{the}\:{word}\:'{EAMCOT}\:'\:{are}\:{arranged}\:{in}\:{different}\:\: \\ $$$${possible}\:{ways}.\:{The}\:{number}\:{of}\:{such}\:{arrangement}\:{in}\:{which}\: \\ $$$${no}\:{two}\:{vowels}\:{are}\:{adjacent}\:{to}\:{each}\:{other}\:{is}\:\_\_\_ \\ $$

Question Number 108815 Answers: 0 Comments: 0

f(x)=(1/( (√(1+x))))+(1/( (√(1+a))))+((√(ax))/( (√(ax+8)))) x>0 , a>0 , x∈R, a∈R prove:1<f(x)<2

$${f}\left({x}\right)=\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{x}}}+\frac{\mathrm{1}}{\:\sqrt{\mathrm{1}+{a}}}+\frac{\sqrt{{ax}}}{\:\sqrt{{ax}+\mathrm{8}}} \\ $$$${x}>\mathrm{0}\:,\:{a}>\mathrm{0}\:,\:{x}\in{R},\:{a}\in{R} \\ $$$${prove}:\mathrm{1}<{f}\left({x}\right)<\mathrm{2} \\ $$

Question Number 108813 Answers: 0 Comments: 0

lim_(x→∞) (−ln 2.1)^(2x) =? ??????

$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left(−\mathrm{ln}\:\mathrm{2}.\mathrm{1}\right)^{\mathrm{2x}} =? \\ $$$$?????? \\ $$

Question Number 108805 Answers: 0 Comments: 0

Let the first term and the common ratio of a geometric sequence {a_n } be 1 and r. If {a_n } satisfy ∣a_(n−1) −a_1 ∣≤∣a_n −a_1 ∣ for all n≥2, find the range of values of r.

$$\mathrm{Let}\:\mathrm{the}\:\mathrm{first}\:\mathrm{term}\:\mathrm{and}\:\mathrm{the}\:\mathrm{common} \\ $$$$\mathrm{ratio}\:\mathrm{of}\:\mathrm{a}\:\mathrm{geometric}\:\mathrm{sequence}\:\left\{{a}_{\mathrm{n}} \right\}\:\mathrm{be}\:\mathrm{1} \\ $$$$\mathrm{and}\:{r}.\: \\ $$$$\mathrm{If}\:\left\{{a}_{\mathrm{n}} \right\}\:\mathrm{satisfy}\:\mid{a}_{\mathrm{n}−\mathrm{1}} −{a}_{\mathrm{1}} \mid\leqslant\mid{a}_{\mathrm{n}} −{a}_{\mathrm{1}} \mid\:\mathrm{for} \\ $$$$\mathrm{all}\:\mathrm{n}\geqslant\mathrm{2},\:\mathrm{find}\:\mathrm{the}\:\mathrm{range}\:\mathrm{of}\:\mathrm{values}\:\mathrm{of}\:{r}. \\ $$

Question Number 108802 Answers: 1 Comments: 0

Question Number 108790 Answers: 1 Comments: 1

The values of θ lying between 0 and (π/2) and satisfying the equation determinant (((1+sin^2 θ),( cos^2 θ),(4 sin 4θ)),(( sin^2 θ),(1+cos^2 θ),(4 sin 4θ)),(( sin^2 θ),( cos^2 θ),(1+sin^4 θ)))=0 are

$$\mathrm{The}\:\mathrm{values}\:\mathrm{of}\:\theta\:\mathrm{lying}\:\mathrm{between}\:\mathrm{0}\:\mathrm{and} \\ $$$$\frac{\pi}{\mathrm{2}}\:\mathrm{and}\:\mathrm{satisfying}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\begin{vmatrix}{\mathrm{1}+\mathrm{sin}^{\mathrm{2}} \theta}&{\:\:\mathrm{cos}^{\mathrm{2}} \theta}&{\mathrm{4}\:\mathrm{sin}\:\mathrm{4}\theta}\\{\:\:\:\mathrm{sin}^{\mathrm{2}} \theta}&{\mathrm{1}+\mathrm{cos}^{\mathrm{2}} \theta}&{\mathrm{4}\:\mathrm{sin}\:\mathrm{4}\theta}\\{\:\:\:\mathrm{sin}^{\mathrm{2}} \theta}&{\:\:\:\mathrm{cos}^{\mathrm{2}} \theta}&{\mathrm{1}+\mathrm{sin}^{\mathrm{4}} \theta}\end{vmatrix}=\mathrm{0}\:\:\mathrm{are} \\ $$

Question Number 108789 Answers: 1 Comments: 0

Question Number 108787 Answers: 1 Comments: 0

Pg 1077 Pg 1078 Pg 1079 Pg 1080 Pg 1081 Pg 1082 Pg 1083 Pg 1084 Pg 1085 Pg 1086

Contact: info@tinkutara.com