Question and Answers Forum

AllQuestion and Answers: Page 1081

Question Number 108692 Answers: 2 Comments: 0

Question Number 108690 Answers: 0 Comments: 0

Question Number 108688 Answers: 0 Comments: 4

prove that Σ_(n=−∞) ^∞ (1/((ax+1)^n )) =−(π/a^n ) lim_(x→−(1/a)) (1/((n−1)!)){cotan(πx)}^((n−1))

$${prove}\:{that}\: \\ $$$$\sum_{{n}=−\infty} ^{\infty} \:\frac{\mathrm{1}}{\left({ax}+\mathrm{1}\right)^{{n}} } \\ $$$$=−\frac{\pi}{{a}^{{n}} }\:{lim}_{{x}\rightarrow−\frac{\mathrm{1}}{{a}}} \:\:\:\frac{\mathrm{1}}{\left({n}−\mathrm{1}\right)!}\left\{{cotan}\left(\pi{x}\right)\right\}^{\left({n}−\mathrm{1}\right)} \\ $$

Question Number 108680 Answers: 0 Comments: 1

Question Number 108679 Answers: 1 Comments: 0

Question Number 108678 Answers: 2 Comments: 0

Question Number 108674 Answers: 2 Comments: 0

Solve log_3 (y−2)+log_y (y+5)=2

$$\mathrm{Solve}\:\:\mathrm{log}_{\mathrm{3}} \left(\mathrm{y}−\mathrm{2}\right)+\mathrm{log}_{\mathrm{y}} \left(\mathrm{y}+\mathrm{5}\right)=\mathrm{2} \\ $$

Question Number 108667 Answers: 4 Comments: 0

Question Number 108664 Answers: 1 Comments: 0

∫_0 ^( 2) ∫_0 ^( 2) x^2 sin(xy)dxdy

$$\int_{\mathrm{0}} ^{\:\mathrm{2}} \int_{\mathrm{0}} ^{\:\mathrm{2}} {x}^{\mathrm{2}} {sin}\left({xy}\right){dxdy} \\ $$

Question Number 108663 Answers: 1 Comments: 0

Question Number 108652 Answers: 1 Comments: 1

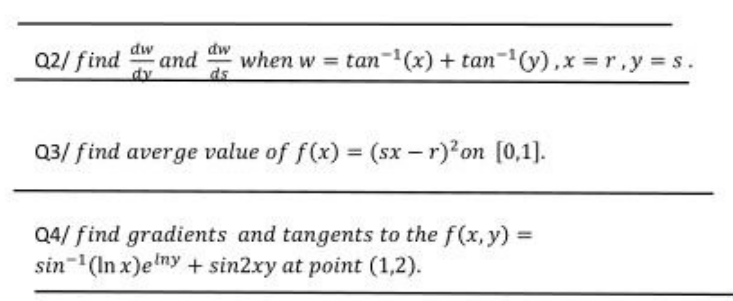
Question Number 108845 Answers: 0 Comments: 0

Question Number 108644 Answers: 4 Comments: 1

((bemath)/★) find the value of sin ((π/9))sin (((2π)/9))sin (((3π)/9))sin (((4π)/9))?

$$\:\:\:\frac{{bemath}}{\bigstar} \\ $$$${find}\:{the}\:{value}\:{of}\: \\ $$$$\mathrm{sin}\:\left(\frac{\pi}{\mathrm{9}}\right)\mathrm{sin}\:\left(\frac{\mathrm{2}\pi}{\mathrm{9}}\right)\mathrm{sin}\:\left(\frac{\mathrm{3}\pi}{\mathrm{9}}\right)\mathrm{sin}\:\left(\frac{\mathrm{4}\pi}{\mathrm{9}}\right)?\: \\ $$

Question Number 108643 Answers: 2 Comments: 0

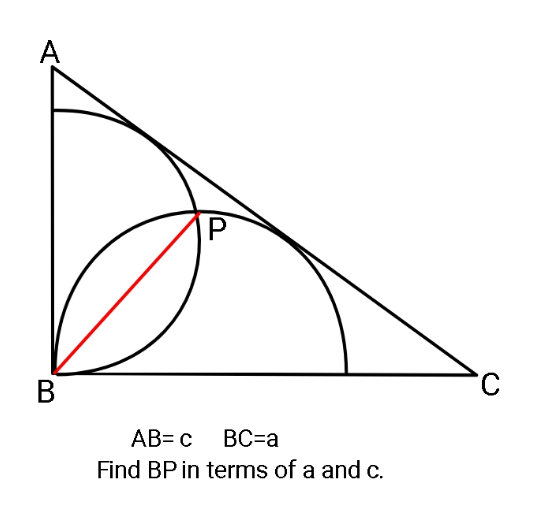
Question Number 108638 Answers: 1 Comments: 0

((bemath)/★) prove that (((n−1)),(( r)) ) + (((n−1)),((r−1)) ) = ((n),(r) )

$$\:\:\:\frac{{bemath}}{\bigstar} \\ $$$${prove}\:{that}\:\begin{pmatrix}{{n}−\mathrm{1}}\\{\:\:\:\:\:{r}}\end{pmatrix}\:+\:\begin{pmatrix}{{n}−\mathrm{1}}\\{{r}−\mathrm{1}}\end{pmatrix}\:=\:\begin{pmatrix}{{n}}\\{{r}}\end{pmatrix} \\ $$

Question Number 108637 Answers: 2 Comments: 0

Question Number 108628 Answers: 0 Comments: 4

Prove that the inequality ∣cos x∣ ≥ 1 − sin^2 x hold true for all x ∈ R

$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{the}\:\mathrm{inequality}\:\:\mid\mathrm{cos}\:\mathrm{x}\mid\:\:\geqslant\:\:\:\mathrm{1}\:\:−\:\:\mathrm{sin}^{\mathrm{2}} \mathrm{x}\:\:\:\:\mathrm{hold}\:\mathrm{true}\:\mathrm{for}\:\mathrm{all}\:\:\mathrm{x}\:\in\:\mathbb{R} \\ $$

Question Number 108626 Answers: 1 Comments: 0

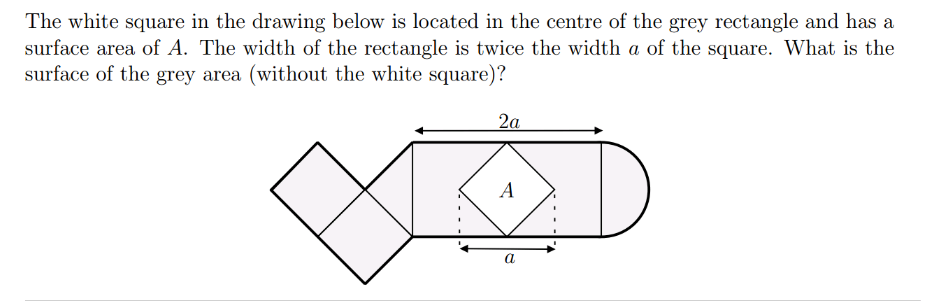
Question Number 108623 Answers: 3 Comments: 0

((⊃BeMath⊃)/★) (1)lim_(b→a) ((b(√a)−a(√b))/(a(√a)+b(√a)−2a(√a))) (2) lim_(x→0) ((3e^(2x) +e^x −4)/x)

$$\:\:\frac{\supset\mathcal{B}{e}\mathcal{M}{ath}\supset}{\bigstar} \\ $$$$\:\left(\mathrm{1}\right)\underset{{b}\rightarrow{a}} {\mathrm{lim}}\:\frac{{b}\sqrt{{a}}−{a}\sqrt{{b}}}{{a}\sqrt{{a}}+{b}\sqrt{{a}}−\mathrm{2}{a}\sqrt{{a}}} \\ $$$$\left(\mathrm{2}\right)\:\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\mathrm{3}{e}^{\mathrm{2}{x}} +{e}^{{x}} −\mathrm{4}}{{x}} \\ $$

Question Number 108619 Answers: 1 Comments: 3

Question Number 108614 Answers: 0 Comments: 0

Question Number 108612 Answers: 0 Comments: 0

Question Number 108609 Answers: 1 Comments: 1

1 + 2 + 3 + 4 + 5 + ... ∞ = ???

$$\mathrm{1}\:+\:\mathrm{2}\:+\:\mathrm{3}\:+\:\mathrm{4}\:+\:\mathrm{5}\:+\:...\:\infty\:=\:??? \\ $$

Question Number 108605 Answers: 2 Comments: 0

((BeMath)/≊) ∫ (x^(11) /((x^8 +1)^2 )) dx

$$\:\:\:\frac{\boldsymbol{{B}}{e}\boldsymbol{{M}}{ath}}{\approxeq} \\ $$$$\:\int\:\frac{{x}^{\mathrm{11}} }{\left({x}^{\mathrm{8}} +\mathrm{1}\right)^{\mathrm{2}} }\:{dx}\: \\ $$

Question Number 108597 Answers: 3 Comments: 0

((∠ BeMath∠)/∦) (1) ∫ cos (ln x) dx (2) ∫ sin (ln x) dx

$$\:\:\:\:\:\frac{\angle\:\mathcal{B}{e}\mathcal{M}{ath}\angle}{\nparallel} \\ $$$$\left(\mathrm{1}\right)\:\int\:\mathrm{cos}\:\left(\mathrm{ln}\:{x}\right)\:{dx}\: \\ $$$$\left(\mathrm{2}\right)\:\int\:\mathrm{sin}\:\left(\mathrm{ln}\:{x}\right)\:{dx}\: \\ $$

Question Number 108594 Answers: 2 Comments: 0

Pg 1076 Pg 1077 Pg 1078 Pg 1079 Pg 1080 Pg 1081 Pg 1082 Pg 1083 Pg 1084 Pg 1085

Contact: info@tinkutara.com