Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1080

Question Number 102967 Answers: 1 Comments: 0

Question Number 102944 Answers: 0 Comments: 5

Question Number 102940 Answers: 0 Comments: 1

Question Number 102927 Answers: 3 Comments: 1

If (x/(x^2 + x + 1)) = (1/4) Find x + (1/x)

$$\mathrm{If}\:\:\:\frac{\mathrm{x}}{\mathrm{x}^{\mathrm{2}} \:\:+\:\:\mathrm{x}\:\:+\:\:\mathrm{1}}\:\:\:=\:\:\frac{\mathrm{1}}{\mathrm{4}} \\ $$$$\mathrm{Find}\:\:\:\:\:\mathrm{x}\:\:+\:\:\frac{\mathrm{1}}{\mathrm{x}} \\ $$

Question Number 102926 Answers: 3 Comments: 0

∫ (dθ/(2sin^2 θ−cos^2 θ)) ?

$$\:\int\:\frac{{d}\theta}{\mathrm{2}{sin}^{\mathrm{2}} \theta−{cos}^{\mathrm{2}} \theta}\:\:? \\ $$

Question Number 102922 Answers: 1 Comments: 1

∫ (dx/(x^3 +3x−5)) ?

$$\int\:\frac{{dx}}{{x}^{\mathrm{3}} +\mathrm{3}{x}−\mathrm{5}}\:\:? \\ $$

Question Number 103071 Answers: 0 Comments: 0

∫(e^x /((1+x^2 )^2 ))∙(x^3 −x^2 +x+1)dx

$$\int\frac{\mathrm{e}^{\mathrm{x}} }{\left(\mathrm{1}+\mathrm{x}^{\mathrm{2}} \right)^{\mathrm{2}} }\centerdot\left(\mathrm{x}^{\mathrm{3}} −\mathrm{x}^{\mathrm{2}} +\mathrm{x}+\mathrm{1}\right)\mathrm{dx} \\ $$

Question Number 102918 Answers: 1 Comments: 2

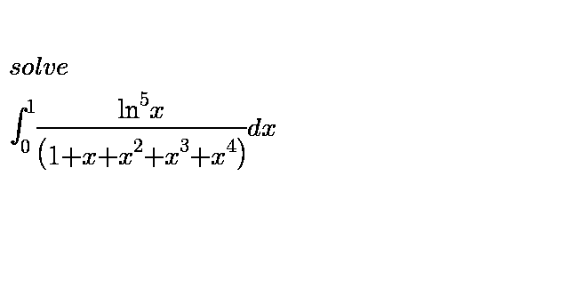
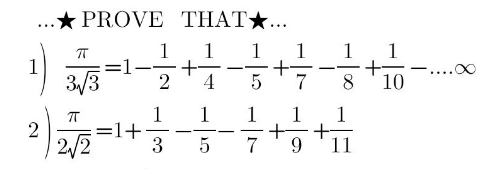
Question Number 102911 Answers: 2 Comments: 1

∫ ((sin(x))/x) dx

$$\int\:\frac{\mathrm{sin}\left(\mathrm{x}\right)}{\mathrm{x}}\:\:\mathrm{dx} \\ $$

Question Number 102910 Answers: 1 Comments: 0

(1)Σ_(m = 1) ^n tan^2 (((mπ)/(2n+1))) (2) Π_(m = 1) ^n tan^2 (((mπ)/(2n+1)))

$$\left(\mathrm{1}\right)\underset{\mathrm{m}\:=\:\mathrm{1}} {\overset{\mathrm{n}} {\sum}}\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\mathrm{m}\pi}{\mathrm{2n}+\mathrm{1}}\right) \\ $$$$\left(\mathrm{2}\right)\:\underset{\mathrm{m}\:=\:\mathrm{1}} {\overset{\mathrm{n}} {\prod}}\mathrm{tan}\:^{\mathrm{2}} \left(\frac{\mathrm{m}\pi}{\mathrm{2n}+\mathrm{1}}\right)\: \\ $$

Question Number 102905 Answers: 1 Comments: 0

I=2∫_0 ^(1/(√2)) ((sin^(−1) (x))/x) dx −∫_0 ^1 ((tan^(−1) (x))/x)dx

$${I}=\mathrm{2}\underset{\mathrm{0}} {\overset{\frac{\mathrm{1}}{\sqrt{\mathrm{2}}}} {\int}}\:\frac{\mathrm{sin}^{−\mathrm{1}} \left({x}\right)}{{x}}\:{dx}\:−\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{tan}^{−\mathrm{1}} \left({x}\right)}{{x}}{dx} \\ $$

Question Number 102880 Answers: 1 Comments: 5

Question Number 102963 Answers: 0 Comments: 0

Question Number 102857 Answers: 0 Comments: 1

Question Number 102854 Answers: 1 Comments: 0

Question Number 102883 Answers: 1 Comments: 0

If x^3 +ax^2 +bx+c = 0 has the roots are α^ β and γ . find the value of αβ^2 +βγ^2 +γα^2 in terms a,b and c

$${If}\:{x}^{\mathrm{3}} +{ax}^{\mathrm{2}} +{bx}+{c}\:=\:\mathrm{0}\:{has}\:{the}\:{roots}\:{are}\: \\ $$$$\bar {\alpha}\:\beta\:{and}\:\gamma\:.\:{find}\:{the}\:{value}\:{of} \\ $$$$\alpha\beta^{\mathrm{2}} +\beta\gamma^{\mathrm{2}} +\gamma\alpha^{\mathrm{2}} \:{in}\:{terms}\:{a},{b}\:{and}\:{c} \\ $$

Question Number 102843 Answers: 0 Comments: 8

Question Number 102840 Answers: 1 Comments: 0

y′+(√(x+y−1))=x+y+1

$${y}'+\sqrt{{x}+{y}−\mathrm{1}}={x}+{y}+\mathrm{1} \\ $$

Question Number 102838 Answers: 1 Comments: 2

Question Number 102836 Answers: 2 Comments: 1

Question Number 102822 Answers: 1 Comments: 1

Σ_(n=1) ^∞ ((n!)/n^n )

$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{{n}!}{{n}^{{n}} } \\ $$

Question Number 102819 Answers: 0 Comments: 4

Question Number 102816 Answers: 2 Comments: 28

How many 6 digit numbers exist whose digits have exactly the sum 13? for example 120505 is such a number.

$${How}\:{many}\:\mathrm{6}\:{digit}\:{numbers}\:{exist} \\ $$$${whose}\:{digits}\:{have}\:{exactly}\:{the}\:{sum}\:\mathrm{13}? \\ $$$$ \\ $$$${for}\:{example}\:\mathrm{120505}\:{is}\:{such}\:{a}\:{number}. \\ $$

Question Number 102813 Answers: 0 Comments: 0

Question Number 102804 Answers: 1 Comments: 0

The coordinates of two points A & B are (0,8) and (9,4) respectively. The point P with coordinate (p,0) lies on the x−axis where 0<p<9. Let s denotes the sum of the length of two segments PA and PB . by expressing s in terms of p otherwise, show that (ds/dp) = (p/(√(64+p^2 ))) − ((9−p)/(√(16+(9−p)^2 )))

$$\mathrm{The}\:\mathrm{coordinates}\:\mathrm{of}\:\mathrm{two}\:\mathrm{points}\:\mathrm{A} \\ $$$$\&\:\mathrm{B}\:\mathrm{are}\:\left(\mathrm{0},\mathrm{8}\right)\:\mathrm{and}\:\left(\mathrm{9},\mathrm{4}\right) \\ $$$$\mathrm{respectively}.\:\mathrm{The}\:\mathrm{point}\:\mathrm{P}\:\mathrm{with} \\ $$$$\mathrm{coordinate}\:\left(\mathrm{p},\mathrm{0}\right)\:\mathrm{lies}\:\mathrm{on}\:\mathrm{the} \\ $$$$\mathrm{x}−\mathrm{axis}\:\mathrm{where}\:\mathrm{0}<\mathrm{p}<\mathrm{9}.\:\mathrm{Let}\:\mathrm{s} \\ $$$$\mathrm{denotes}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{length}\:\mathrm{of} \\ $$$$\mathrm{two}\:\mathrm{segments}\:\mathrm{PA}\:\mathrm{and}\:\mathrm{PB}\:.\:\mathrm{by} \\ $$$$\mathrm{expressing}\:\mathrm{s}\:\mathrm{in}\:\mathrm{terms}\:\mathrm{of}\:\mathrm{p} \\ $$$$\mathrm{otherwise},\:\mathrm{show}\:\mathrm{that}\:\frac{\mathrm{ds}}{\mathrm{dp}}\:= \\ $$$$\frac{\mathrm{p}}{\sqrt{\mathrm{64}+\mathrm{p}^{\mathrm{2}} }}\:−\:\frac{\mathrm{9}−\mathrm{p}}{\sqrt{\mathrm{16}+\left(\mathrm{9}−\mathrm{p}\right)^{\mathrm{2}} }} \\ $$

Question Number 102800 Answers: 1 Comments: 0

There are 14 boys and 10 girls in a classroom. The teacher wants to form a team of 5 students . The team must have a least two boys and two girls . Find the number of ways the team can be chosen.

$$\mathrm{There}\:\mathrm{are}\:\mathrm{14}\:\mathrm{boys}\:\mathrm{and}\:\mathrm{10}\:\mathrm{girls}\:\mathrm{in} \\ $$$$\mathrm{a}\:\mathrm{classroom}.\:\mathrm{The}\:\mathrm{teacher}\:\mathrm{wants} \\ $$$$\mathrm{to}\:\mathrm{form}\:\mathrm{a}\:\mathrm{team}\:\mathrm{of}\:\mathrm{5}\:\mathrm{students}\:. \\ $$$$\mathrm{The}\:\mathrm{team}\:\mathrm{must}\:\mathrm{have}\:\mathrm{a}\:\mathrm{least}\:\mathrm{two} \\ $$$$\mathrm{boys}\:\mathrm{and}\:\mathrm{two}\:\mathrm{girls}\:.\:\mathrm{Find}\:\mathrm{the} \\ $$$$\mathrm{number}\:\mathrm{of}\:\mathrm{ways}\:\mathrm{the}\:\mathrm{team}\:\mathrm{can}\:\mathrm{be} \\ $$$$\mathrm{chosen}.\: \\ $$

Pg 1075 Pg 1076 Pg 1077 Pg 1078 Pg 1079 Pg 1080 Pg 1081 Pg 1082 Pg 1083 Pg 1084

Contact: info@tinkutara.com