
AllQuestion and Answers: Page 1048
Question Number 113354 Answers: 0 Comments: 3
Question Number 113353 Answers: 1 Comments: 0
Question Number 113346 Answers: 1 Comments: 0

Question Number 113343 Answers: 1 Comments: 1
Question Number 113341 Answers: 3 Comments: 0

Question Number 113336 Answers: 0 Comments: 0
Question Number 113333 Answers: 2 Comments: 0

Question Number 113330 Answers: 1 Comments: 0

Question Number 113323 Answers: 2 Comments: 0

Question Number 113309 Answers: 2 Comments: 4
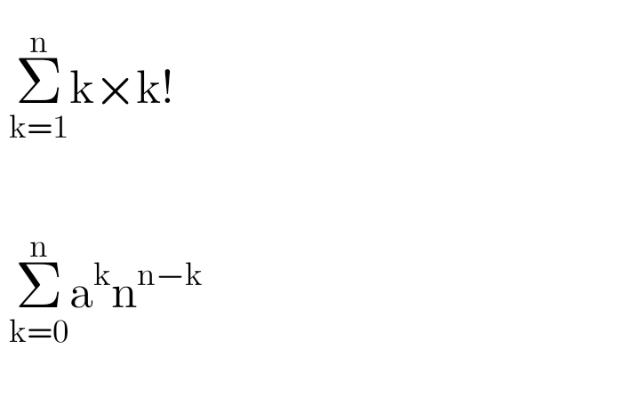
Question Number 113375 Answers: 0 Comments: 1
Question Number 113374 Answers: 1 Comments: 5
Question Number 113372 Answers: 1 Comments: 2
Question Number 113278 Answers: 2 Comments: 0
Question Number 113275 Answers: 2 Comments: 0
Question Number 113274 Answers: 4 Comments: 2
Question Number 113272 Answers: 2 Comments: 0
Question Number 113271 Answers: 0 Comments: 0
Question Number 113262 Answers: 2 Comments: 0
Question Number 113261 Answers: 1 Comments: 0
Question Number 113246 Answers: 1 Comments: 1

Question Number 113243 Answers: 1 Comments: 3
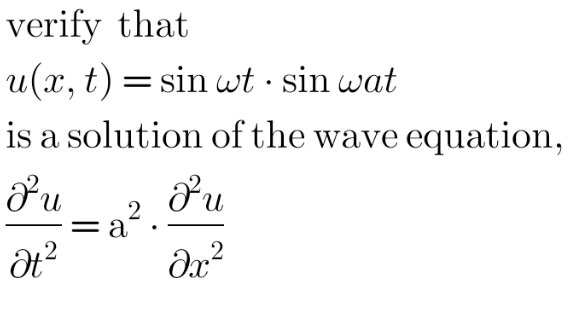
Question Number 113241 Answers: 1 Comments: 0

Question Number 113237 Answers: 1 Comments: 0
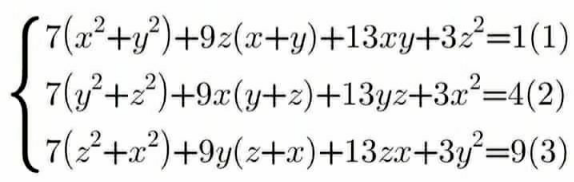
Question Number 121155 Answers: 0 Comments: 0

Question Number 113221 Answers: 0 Comments: 3
Pg 1043 Pg 1044 Pg 1045 Pg 1046 Pg 1047 Pg 1048 Pg 1049 Pg 1050 Pg 1051 Pg 1052
