
AllQuestion and Answers: Page 1046
Question Number 112992 Answers: 1 Comments: 1

Question Number 112986 Answers: 0 Comments: 0

Question Number 112974 Answers: 2 Comments: 0
Question Number 112971 Answers: 1 Comments: 1
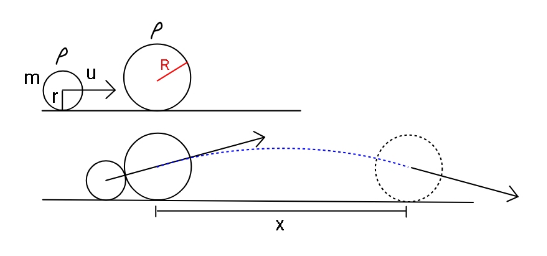
Question Number 112962 Answers: 4 Comments: 3

Question Number 112994 Answers: 1 Comments: 0
Question Number 112958 Answers: 0 Comments: 3

Question Number 112934 Answers: 1 Comments: 7
Question Number 112917 Answers: 1 Comments: 3
Question Number 112908 Answers: 2 Comments: 1
Question Number 112906 Answers: 0 Comments: 4
Question Number 112904 Answers: 1 Comments: 0
Question Number 112902 Answers: 3 Comments: 0
Question Number 112900 Answers: 1 Comments: 1
Question Number 112885 Answers: 0 Comments: 9
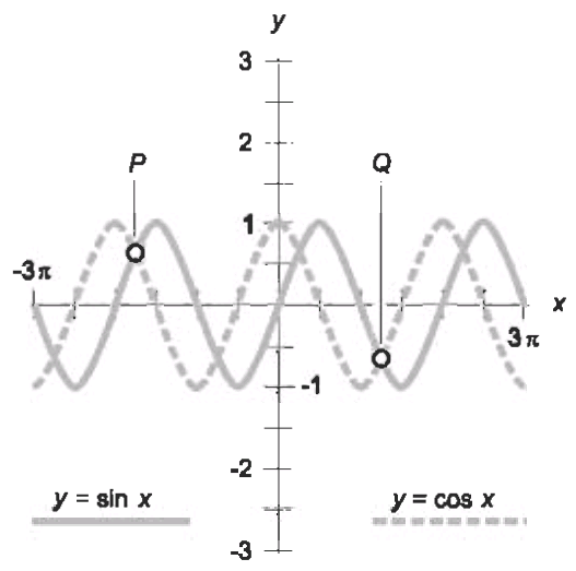
Question Number 112882 Answers: 3 Comments: 2

Question Number 112881 Answers: 1 Comments: 0
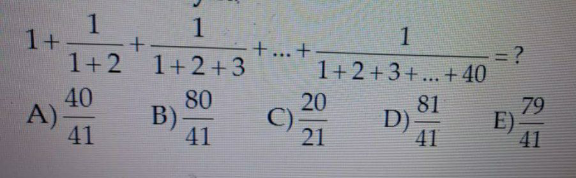
Question Number 112874 Answers: 1 Comments: 2
Question Number 112867 Answers: 2 Comments: 2
Question Number 112866 Answers: 6 Comments: 0

Question Number 112863 Answers: 4 Comments: 1
Question Number 112855 Answers: 3 Comments: 0
Question Number 112854 Answers: 0 Comments: 3
Question Number 112851 Answers: 2 Comments: 0
Question Number 112848 Answers: 0 Comments: 1
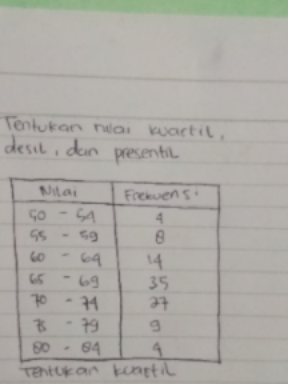
Question Number 112847 Answers: 1 Comments: 0
Pg 1041 Pg 1042 Pg 1043 Pg 1044 Pg 1045 Pg 1046 Pg 1047 Pg 1048 Pg 1049 Pg 1050
