Question and Answers Forum

AllQuestion and Answers: Page 1040

Question Number 115439 Answers: 0 Comments: 1

Question Number 115438 Answers: 2 Comments: 0

Question Number 115436 Answers: 1 Comments: 0

(√((a)^(1/3) +(b)^(1/3) ))=(1/( (√(b−a×s^3 ))))(((−s^2 ×(a^2 )^(1/3) )/2)+s((ab))^(1/3) +(b^2 )^(1/3) ) what is s?

$$\sqrt{\sqrt[{\mathrm{3}}]{{a}}+\sqrt[{\mathrm{3}}]{{b}}}=\frac{\mathrm{1}}{\:\sqrt{{b}−{a}×{s}^{\mathrm{3}} }}\left(\frac{−{s}^{\mathrm{2}} ×\sqrt[{\mathrm{3}}]{{a}^{\mathrm{2}} }}{\mathrm{2}}+{s}\sqrt[{\mathrm{3}}]{{ab}}+\sqrt[{\mathrm{3}}]{{b}^{\mathrm{2}} }\right) \\ $$$${what}\:{is}\:{s}? \\ $$

Question Number 115429 Answers: 0 Comments: 9

how many ways can you arrange 15 distinct balls into 5 cups if there has to be at least 1 ball in each cup?

$${how}\:{many}\:{ways}\:{can}\:{you}\:{arrange}\:\mathrm{15}\:{distinct} \\ $$$${balls}\:{into}\:\mathrm{5}\:{cups}\:{if}\:{there}\:{has}\:{to}\:{be}\:{at}\:{least} \\ $$$$\mathrm{1}\:{ball}\:{in}\:{each}\:{cup}? \\ $$$$ \\ $$

Question Number 115418 Answers: 3 Comments: 1

Question Number 115417 Answers: 2 Comments: 0

with the use of mathematical induction show that n!>2n^3 , ∀n≥6.

$$\mathrm{with}\:\mathrm{the}\:\mathrm{use}\:\mathrm{of}\:\mathrm{mathematical}\:\mathrm{induction} \\ $$$$\mathrm{show}\:\mathrm{that}\:\mathrm{n}!>\mathrm{2n}^{\mathrm{3}} ,\:\forall\mathrm{n}\geqslant\mathrm{6}. \\ $$

Question Number 115464 Answers: 5 Comments: 0

I= ∫_(0 ) ^1 x ln (1+x^2 ) dx ? I=∫ (√(sin x)) .cos^3 x dx ?

$${I}=\:\underset{\mathrm{0}\:} {\overset{\mathrm{1}} {\int}}\:{x}\:\mathrm{ln}\:\left(\mathrm{1}+{x}^{\mathrm{2}} \right)\:{dx}\:? \\ $$$${I}=\int\:\sqrt{\mathrm{sin}\:{x}}\:.\mathrm{cos}\:^{\mathrm{3}} {x}\:{dx}\:? \\ $$

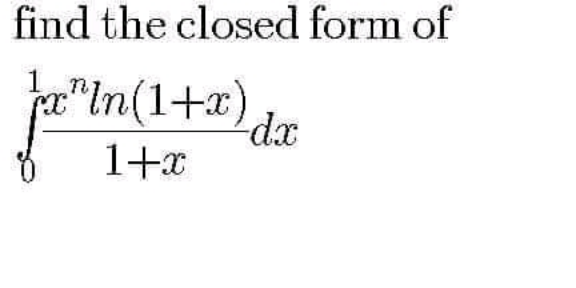
Question Number 115408 Answers: 1 Comments: 0

how many 6 digit numbers exist which are divisible by 11 and have no repeating digits?

$${how}\:{many}\:\mathrm{6}\:{digit}\:{numbers}\:{exist} \\ $$$${which}\:{are}\:{divisible}\:{by}\:\mathrm{11}\:{and}\:{have}\:{no} \\ $$$${repeating}\:{digits}? \\ $$

Question Number 115405 Answers: 1 Comments: 0

find the close form of Σ_(n=0) ^∞ (((−1)^n )/((n+1)(n+2)(2n+1)(2n+3)))

$${find}\:{the}\:{close}\:{form}\:{of} \\ $$$$\underset{{n}=\mathrm{0}} {\overset{\infty} {\sum}}\frac{\left(−\mathrm{1}\right)^{{n}} }{\left({n}+\mathrm{1}\right)\left({n}+\mathrm{2}\right)\left(\mathrm{2}{n}+\mathrm{1}\right)\left(\mathrm{2}{n}+\mathrm{3}\right)} \\ $$

Question Number 115404 Answers: 4 Comments: 0

∫_( 0) ^∞ (1/(1+x^4 )) dx =

$$\:\underset{\:\mathrm{0}} {\overset{\infty} {\int}}\:\:\frac{\mathrm{1}}{\mathrm{1}+{x}^{\mathrm{4}} }\:{dx}\:= \\ $$

Question Number 115403 Answers: 0 Comments: 0

∫ e^x ((1+n x^(n−1) −x^(2n) )/((1−x)^n (√(1−x^(2n) )))) dx =

$$\int\:{e}^{{x}} \:\frac{\mathrm{1}+{n}\:{x}^{{n}−\mathrm{1}} −{x}^{\mathrm{2}{n}} }{\left(\mathrm{1}−{x}\right)^{{n}} \:\:\sqrt{\mathrm{1}−{x}^{\mathrm{2}{n}} }}\:{dx}\:= \\ $$

Question Number 115399 Answers: 1 Comments: 0

The coefficient of the term independent of x in the expansion of (((x+1)/(x^(2/3) − x^(1/3) + 1)) − ((x−1)/(x−x^(1/2) )))^(10) is

$$\mathrm{The}\:\mathrm{coefficient}\:\mathrm{of}\:\mathrm{the}\:\mathrm{term}\:\mathrm{independent} \\ $$$$\mathrm{of}\:{x}\:\mathrm{in}\:\mathrm{the}\:\mathrm{expansion}\:\mathrm{of} \\ $$$$\left(\frac{{x}+\mathrm{1}}{{x}^{\mathrm{2}/\mathrm{3}} −\:{x}^{\mathrm{1}/\mathrm{3}} +\:\mathrm{1}}\:−\:\frac{{x}−\mathrm{1}}{{x}−{x}^{\mathrm{1}/\mathrm{2}} }\right)^{\mathrm{10}} \:\:\mathrm{is} \\ $$

Question Number 115397 Answers: 0 Comments: 0

Question Number 115396 Answers: 3 Comments: 0

If a page is torn from the middle of a book, then the sum of the remaining pages is 718797 so what is the number of torn pages?

$$\mathrm{If}\:\mathrm{a}\:\mathrm{page}\:\mathrm{is}\:\mathrm{torn}\:\mathrm{from}\:\mathrm{the}\:\mathrm{middle}\:\mathrm{of}\:\mathrm{a}\:\mathrm{book},\:\mathrm{then} \\ $$$$\mathrm{the}\:\:\mathrm{sum}\:\mathrm{of}\:\mathrm{the}\:\mathrm{remaining}\:\mathrm{pages}\:\mathrm{is}\:\mathrm{718797}\:\mathrm{so} \\ $$$$\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{torn}\:\mathrm{pages}? \\ $$

Question Number 115401 Answers: 2 Comments: 0

∫((√x)/(x^2 +1))dx=?

$$\int\frac{\sqrt{\mathrm{x}}}{\mathrm{x}^{\mathrm{2}} +\mathrm{1}}\mathrm{dx}=? \\ $$

Question Number 115391 Answers: 1 Comments: 0

Who can explain me what is “R_n [x]”? In French if possible.

$$\mathrm{Who}\:\mathrm{can}\:\mathrm{explain} \\ $$$$\mathrm{me}\:\mathrm{what}\:\mathrm{is}\:``\mathbb{R}_{{n}} \left[{x}\right]''? \\ $$$${In}\:{French}\:{if}\:{possible}. \\ $$

Question Number 115387 Answers: 0 Comments: 6

Question Number 115384 Answers: 1 Comments: 0

solve the limit problem lim_(x→∞) (((cosx)/(1−e^(−x) )))

$${solve}\:{the}\:{limit}\:{problem} \\ $$$$\underset{{x}\rightarrow\infty} {\mathrm{lim}}\left(\frac{\mathrm{cos}{x}}{\mathrm{1}−{e}^{−{x}} }\right) \\ $$

Question Number 115471 Answers: 0 Comments: 1

For angles a,b,c∈R with a+b+c=π, prove the following identities: tan^(−1) ((a/2))+tan^(−1) ((b/2))+tan^(−1) ((c/2))=(tan((a/2))tan((b/2))tan((c/2)))^(−1) Help

$${For}\:{angles}\:{a},{b},{c}\in\mathbb{R}\:{with}\:{a}+{b}+{c}=\pi,\: \\ $$$${prove}\:{the}\:{following}\:{identities}: \\ $$$$\mathrm{tan}^{−\mathrm{1}} \left(\frac{{a}}{\mathrm{2}}\right)+\mathrm{tan}^{−\mathrm{1}} \left(\frac{{b}}{\mathrm{2}}\right)+\mathrm{tan}^{−\mathrm{1}} \left(\frac{{c}}{\mathrm{2}}\right)=\left(\mathrm{tan}\left(\frac{{a}}{\mathrm{2}}\right)\mathrm{tan}\left(\frac{{b}}{\mathrm{2}}\right)\mathrm{tan}\left(\frac{{c}}{\mathrm{2}}\right)\right)^{−\mathrm{1}} \\ $$$$\mathrm{H}{elp} \\ $$$$ \\ $$

Question Number 115380 Answers: 2 Comments: 0

if a^2 +2ab+3b^2 =1,prove that (a+3b)^3 (d^2 b/da^2 )+2(a^2 +2ab+3b^2 )=0

$${if}\: \\ $$$${a}^{\mathrm{2}} +\mathrm{2}{ab}+\mathrm{3}{b}^{\mathrm{2}} =\mathrm{1},{prove}\:{that}\: \\ $$$$\left({a}+\mathrm{3}{b}\right)^{\mathrm{3}} \frac{{d}^{\mathrm{2}} {b}}{{da}^{\mathrm{2}} }+\mathrm{2}\left({a}^{\mathrm{2}} +\mathrm{2}{ab}+\mathrm{3}{b}^{\mathrm{2}} \right)=\mathrm{0} \\ $$

Question Number 115368 Answers: 1 Comments: 0

an open rectanqular container is to have a volume of 62.5cm^3 .find the least possible surface area of the material required

$${an}\:{open}\:{rectanqular}\:{container}\:{is}\:{to} \\ $$$${have}\:{a}\:{volume}\:{of}\:\mathrm{62}.\mathrm{5}{cm}^{\mathrm{3}} .{find}\:{the}\:{least} \\ $$$${possible}\:{surface}\:{area}\:{of}\:{the}\:{material} \\ $$$${required} \\ $$

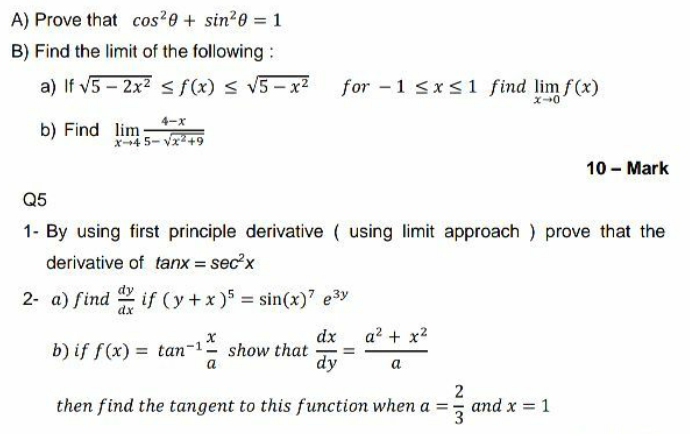
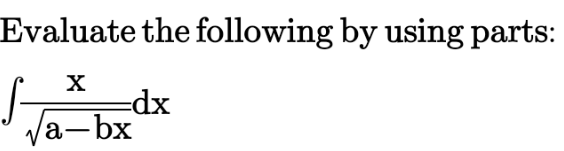
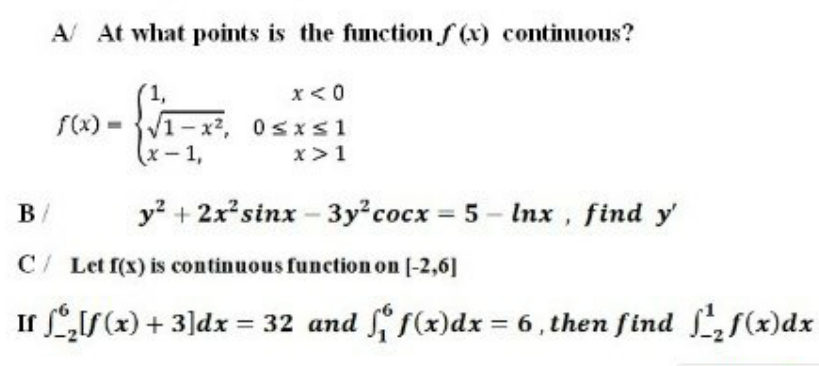
Question Number 115367 Answers: 1 Comments: 0

solve xy^(′′) −(x^2 +1)y^′ =x^2 sin(2x)

$${solve}\:{xy}^{''} −\left({x}^{\mathrm{2}} +\mathrm{1}\right){y}^{'} \:\:={x}^{\mathrm{2}} {sin}\left(\mathrm{2}{x}\right) \\ $$

Question Number 115366 Answers: 2 Comments: 0

calculate ∫_(−1) ^2 (dx/(ch^2 x +sh^2 x))

$${calculate}\:\int_{−\mathrm{1}} ^{\mathrm{2}} \:\frac{{dx}}{{ch}^{\mathrm{2}} {x}\:+{sh}^{\mathrm{2}} {x}} \\ $$

Question Number 115365 Answers: 0 Comments: 0

calculate ∫∫_([0,1]^2 ) ((arctan(xy))/( (√(x^2 +y^2 ))))dxdy

$${calculate}\:\int\int_{\left[\mathrm{0},\mathrm{1}\right]^{\mathrm{2}} } \:\:\frac{{arctan}\left({xy}\right)}{\:\sqrt{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }}{dxdy} \\ $$

Question Number 115364 Answers: 1 Comments: 0

calculate ∫∫_([0,1]^2 ) (√(xy))(x^2 +y^2 )dxdy

$${calculate}\:\int\int_{\left[\mathrm{0},\mathrm{1}\right]^{\mathrm{2}} } \:\:\sqrt{{xy}}\left({x}^{\mathrm{2}} +{y}^{\mathrm{2}} \right){dxdy} \\ $$

Question Number 115363 Answers: 1 Comments: 0

evaluate ∫_0 ^(π/3) (1/(sin^2 x+cos^2 x))dx

$${evaluate} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{3}}} \frac{\mathrm{1}}{\mathrm{sin}^{\mathrm{2}} {x}+\mathrm{cos}^{\mathrm{2}} {x}}{dx} \\ $$

Pg 1035 Pg 1036 Pg 1037 Pg 1038 Pg 1039 Pg 1040 Pg 1041 Pg 1042 Pg 1043 Pg 1044

Contact: info@tinkutara.com