Question and Answers Forum

AllQuestion and Answers: Page 1039

Question Number 115927 Answers: 2 Comments: 0

calculate ∫_1 ^(+∞) (dx/((4x^2 −1)^3 ))

$$\mathrm{calculate}\:\:\int_{\mathrm{1}} ^{+\infty} \:\:\:\:\:\frac{\mathrm{dx}}{\left(\mathrm{4x}^{\mathrm{2}} −\mathrm{1}\right)^{\mathrm{3}} } \\ $$

Question Number 115946 Answers: 1 Comments: 0

Given that the sum of infinity of geometric series a−2ar+4ar^2 −8ar^3 +...a(−2r)^(n−1) +...is 3 and the sum of infinity of geometric series a+ar+ar^2 +ar^3 +...ar^(n−1) +... is k, find the range of values of k.

$$\mathrm{Given}\:\mathrm{that}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{infinity}\:\mathrm{of}\:\mathrm{geometric} \\ $$$$\mathrm{series}\:{a}−\mathrm{2}{ar}+\mathrm{4}{ar}^{\mathrm{2}} −\mathrm{8}{ar}^{\mathrm{3}} +...{a}\left(−\mathrm{2}{r}\right)^{{n}−\mathrm{1}} \:+...\mathrm{is}\:\mathrm{3} \\ $$$$\mathrm{and}\:\mathrm{the}\:\mathrm{sum}\:\mathrm{of}\:\mathrm{infinity}\:\mathrm{of}\:\mathrm{geometric}\:\mathrm{series} \\ $$$${a}+{ar}+{ar}^{\mathrm{2}} +{ar}^{\mathrm{3}} +...{ar}^{{n}−\mathrm{1}} +...\:\mathrm{is}\:{k},\: \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{range}\:\mathrm{of}\:\mathrm{values}\:\mathrm{of}\:{k}. \\ $$

Question Number 115922 Answers: 1 Comments: 0

find the stationary points of the function U=x^2 +y^2 subjects to the constraint x^2 +y^2 +2x−2y+1=0

$${find}\:{the}\:{stationary}\:{points}\:{of}\:{the} \\ $$$${function}\:{U}={x}^{\mathrm{2}} +{y}^{\mathrm{2}} \:{subjects}\:{to} \\ $$$${the}\:{constraint}\: \\ $$$${x}^{\mathrm{2}} +{y}^{\mathrm{2}} +\mathrm{2}{x}−\mathrm{2}{y}+\mathrm{1}=\mathrm{0} \\ $$

Question Number 115932 Answers: 2 Comments: 0

13^(14) ÷4 Remaining?

$$\mathrm{13}^{\mathrm{14}} \boldsymbol{\div}\mathrm{4} \\ $$$$\mathrm{Remaining}? \\ $$

Question Number 115920 Answers: 4 Comments: 0

prove that :: ∫_0 ^( ∞) (tanh^a (x) −tanh^b (x))dx =^(???) ((ψ(((b+1)/2))−ψ(((a+1)/2)))/2) m.n.july.1970

$$\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:{prove}\:\:\:{that}\::: \\ $$$$\: \\ $$$$\:\int_{\mathrm{0}} ^{\:\infty} \left({tanh}^{{a}} \left({x}\right)\:−{tanh}^{{b}} \left({x}\right)\right){dx}\: \\ $$$$\:\:\:\:\:\:\overset{???} {=}\:\:\:\frac{\psi\left(\frac{{b}+\mathrm{1}}{\mathrm{2}}\right)−\psi\left(\frac{{a}+\mathrm{1}}{\mathrm{2}}\right)}{\mathrm{2}} \\ $$$$\:\:\:\:\:\:\:\:\:\:{m}.{n}.{july}.\mathrm{1970} \\ $$$$\: \\ $$

Question Number 115935 Answers: 0 Comments: 0

Question Number 115934 Answers: 0 Comments: 0

Question Number 115916 Answers: 1 Comments: 0

Question Number 115910 Answers: 0 Comments: 1

let x be a posative real number prove that Σ_(n=1) ^∞ (((n−1)!)/((x+1)....(x+n)))=(1/x)

$${let}\:{x}\:{be}\:{a}\:{posative}\:{real}\:{number} \\ $$$${prove}\:{that} \\ $$$$\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\left({n}−\mathrm{1}\right)!}{\left({x}+\mathrm{1}\right)....\left({x}+{n}\right)}=\frac{\mathrm{1}}{{x}} \\ $$

Question Number 115909 Answers: 1 Comments: 0

solve the system of equations x+((3x−y)/(x^2 +y^2 ))=3 , y−((x+3y)/(x^2 +y^2 ))=0

$${solve}\:{the}\:{system}\:{of}\:{equations} \\ $$$${x}+\frac{\mathrm{3}{x}−{y}}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }=\mathrm{3}\:,\:{y}−\frac{{x}+\mathrm{3}{y}}{{x}^{\mathrm{2}} +{y}^{\mathrm{2}} }=\mathrm{0} \\ $$

Question Number 115908 Answers: 1 Comments: 2

what is the cofficient of x^2 (1+x)(1+2x)(1+4x)......(1+2^n x)

$${what}\:{is}\:{the}\:{cofficient}\:{of}\:{x}^{\mathrm{2}} \\ $$$$\left(\mathrm{1}+{x}\right)\left(\mathrm{1}+\mathrm{2}{x}\right)\left(\mathrm{1}+\mathrm{4}{x}\right)......\left(\mathrm{1}+\mathrm{2}^{{n}} {x}\right) \\ $$

Question Number 115906 Answers: 1 Comments: 0

find all pairs of integers (x,y) such that x^3 +y^3 =(x+y)^2

$${find}\:{all}\:{pairs}\:{of}\:{integers}\:\left({x},{y}\right)\:{such}\:{that} \\ $$$${x}^{\mathrm{3}} +{y}^{\mathrm{3}} =\left({x}+{y}\right)^{\mathrm{2}} \\ $$

Question Number 115902 Answers: 0 Comments: 0

The secret number is made from the numbers 1,2,2,3,3,4,5. Many secret numbers can be created if the same number is not adjacent except in the first two place is _ (a)1142 (b) 1212 (c) 1246 (d) 1248 (e) 1250

$${The}\:{secret}\:{number}\:{is}\:{made}\:{from} \\ $$$${the}\:{numbers}\:\mathrm{1},\mathrm{2},\mathrm{2},\mathrm{3},\mathrm{3},\mathrm{4},\mathrm{5}.\: \\ $$$${Many}\:{secret}\:{numbers}\:{can}\:{be}\:{created} \\ $$$${if}\:{the}\:{same}\:{number}\:{is}\:{not}\:{adjacent} \\ $$$${except}\:{in}\:{the}\:{first}\:{two}\:{place}\:{is}\:\_ \\ $$$$\left({a}\right)\mathrm{1142}\:\:\:\:\left({b}\right)\:\mathrm{1212}\:\:\:\:\left({c}\right)\:\mathrm{1246} \\ $$$$\left({d}\right)\:\mathrm{1248}\:\:\:\left({e}\right)\:\mathrm{1250} \\ $$

Question Number 115898 Answers: 1 Comments: 0

If Mike has 10 blocks numbered from 1 through to 10 . What is the probability that he didn′t choose a block number 7?

$${If}\:{Mike}\:{has}\:\mathrm{10}\:{blocks}\:{numbered} \\ $$$${from}\:\mathrm{1}\:{through}\:{to}\:\mathrm{10}\:.\:{What}\:{is}\:{the} \\ $$$${probability}\:{that}\:{he}\:{didn}'{t}\:{choose} \\ $$$${a}\:{block}\:{number}\:\mathrm{7}? \\ $$

Question Number 115897 Answers: 2 Comments: 1

Given a_n = (√(1 + (1 − (1/n))^2 )) + (√(1 + (1 + (1/n))^2 )) The value of Σ_(n=1) ^(2015) ((4/a_n )) is ...

$$\mathrm{Given} \\ $$$${a}_{{n}} \:=\:\sqrt{\mathrm{1}\:+\:\left(\mathrm{1}\:−\:\frac{\mathrm{1}}{{n}}\right)^{\mathrm{2}} }\:+\:\sqrt{\mathrm{1}\:+\:\left(\mathrm{1}\:+\:\frac{\mathrm{1}}{{n}}\right)^{\mathrm{2}} } \\ $$$$\mathrm{The}\:\mathrm{value}\:\mathrm{of}\:\underset{{n}=\mathrm{1}} {\overset{\mathrm{2015}} {\sum}}\left(\frac{\mathrm{4}}{{a}_{{n}} }\right)\:\mathrm{is}\:... \\ $$

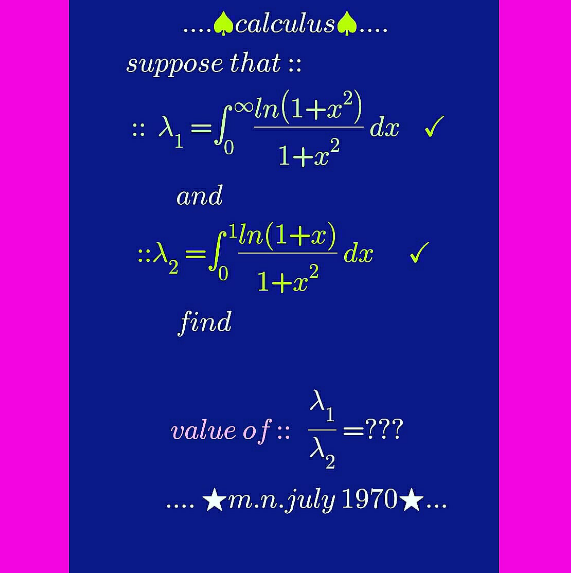
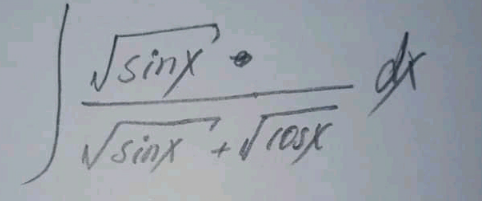
Question Number 115896 Answers: 2 Comments: 0

∫ ((sec^4 x dx)/( (√(tan^3 x)))) =?

$$\:\int\:\frac{\mathrm{sec}\:^{\mathrm{4}} {x}\:{dx}}{\:\sqrt{\mathrm{tan}\:^{\mathrm{3}} {x}}}\:=? \\ $$

Question Number 115892 Answers: 1 Comments: 0

Question Number 115891 Answers: 0 Comments: 0

sec^2 10°+cosec^2 20°+cosec^2 40°−sec^2 45°

$$\mathrm{sec}\:^{\mathrm{2}} \mathrm{10}°+\mathrm{cosec}\:^{\mathrm{2}} \mathrm{20}°+\mathrm{cosec}\:^{\mathrm{2}} \mathrm{40}°−\mathrm{sec}\:^{\mathrm{2}} \mathrm{45}° \\ $$

Question Number 115889 Answers: 1 Comments: 0

lim_(x→0) ((((1+cos 2x))^(1/(3 )) −(2)^(1/(3 )) )/(x^2 .sin 3x))

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\frac{\sqrt[{\mathrm{3}\:}]{\mathrm{1}+\mathrm{cos}\:\mathrm{2}{x}}\:−\sqrt[{\mathrm{3}\:}]{\mathrm{2}}}{{x}^{\mathrm{2}} .\mathrm{sin}\:\mathrm{3}{x}} \\ $$

Question Number 115888 Answers: 0 Comments: 1

2x+3y+4z=1 ⇒min((1/x)+(1/y)+(1/z))

$$\mathrm{2}{x}+\mathrm{3}{y}+\mathrm{4}{z}=\mathrm{1}\:\:\Rightarrow{min}\left(\frac{\mathrm{1}}{{x}}+\frac{\mathrm{1}}{{y}}+\frac{\mathrm{1}}{{z}}\right) \\ $$

Question Number 115885 Answers: 1 Comments: 0

given f((1/x))+f(1−x)=x , x≠0 find 2f(x) (a) ((1+x^2 +x^3 )/(x^2 −x)) (b) ((x^2 −1−x^3 )/(x^2 −x)) (c) ((x^2 −x^3 )/(x^2 +x)) (d) ((x−x^3 )/(x^2 −x)) (e) ((1+x^2 −x^3 )/(x^2 +x))

$${given}\:{f}\left(\frac{\mathrm{1}}{{x}}\right)+{f}\left(\mathrm{1}−{x}\right)={x}\:,\:{x}\neq\mathrm{0} \\ $$$${find}\:\mathrm{2}{f}\left({x}\right) \\ $$$$\left({a}\right)\:\frac{\mathrm{1}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} }{{x}^{\mathrm{2}} −{x}} \\ $$$$\left({b}\right)\:\frac{{x}^{\mathrm{2}} −\mathrm{1}−{x}^{\mathrm{3}} }{{x}^{\mathrm{2}} −{x}} \\ $$$$\left({c}\right)\:\frac{{x}^{\mathrm{2}} −{x}^{\mathrm{3}} }{{x}^{\mathrm{2}} +{x}} \\ $$$$\left({d}\right)\:\frac{{x}−{x}^{\mathrm{3}} }{{x}^{\mathrm{2}} −{x}} \\ $$$$\left({e}\right)\:\frac{\mathrm{1}+{x}^{\mathrm{2}} −{x}^{\mathrm{3}} }{{x}^{\mathrm{2}} +{x}} \\ $$

Question Number 115884 Answers: 0 Comments: 0

Question Number 115882 Answers: 1 Comments: 0

Question Number 115876 Answers: 1 Comments: 0

Prove that f(x)=ax^2 +bx+c has no real roots if and only if a∙[f(−(b/(2a)))]>0

$$\mathrm{Prove}\:\mathrm{that}\:{f}\left({x}\right)={ax}^{\mathrm{2}} +{bx}+{c}\:\mathrm{has}\: \\ $$$$\mathrm{no}\:\mathrm{real}\:\mathrm{roots}\:\mathrm{if}\:\mathrm{and}\:\mathrm{only}\:\mathrm{if}\:{a}\centerdot\left[{f}\left(−\frac{{b}}{\mathrm{2}{a}}\right)\right]>\mathrm{0} \\ $$

Question Number 115869 Answers: 0 Comments: 0

(dy/dx) +2y = x^3 .e^(−2y)

$$\:\frac{{dy}}{{dx}}\:+\mathrm{2}{y}\:=\:{x}^{\mathrm{3}} .{e}^{−\mathrm{2}{y}} \\ $$

Question Number 115859 Answers: 2 Comments: 0

Determine, in simplest form the smallest of the three numbers x, y and z which satisfy the system { ((log _9 (x)+log _9 (y)+log _3 (z)=2)),((log _(16) (x)+log _4 (y)+log _(16) (z)=1)),((log _5 (x)+log _(25) (y)+log _(25) (z)=0)) :}

Pg 1034 Pg 1035 Pg 1036 Pg 1037 Pg 1038 Pg 1039 Pg 1040 Pg 1041 Pg 1042 Pg 1043

Contact: info@tinkutara.com