Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1005

Question Number 117646 Answers: 2 Comments: 1

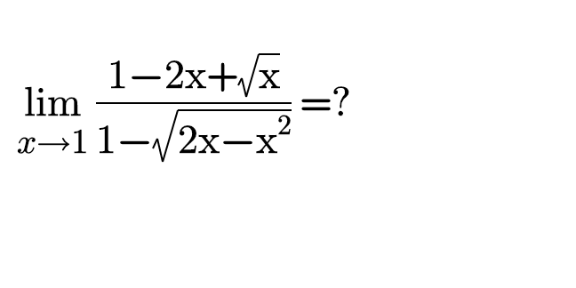
Question Number 117645 Answers: 1 Comments: 0

Question Number 117641 Answers: 3 Comments: 0

find the solution (√(6−x)) > x−4

$$\mathrm{find}\:\mathrm{the}\:\mathrm{solution}\:\sqrt{\mathrm{6}−\mathrm{x}}\:>\:\mathrm{x}−\mathrm{4} \\ $$

Question Number 117638 Answers: 2 Comments: 0

∫_0 ^1 ((2x^(12) +5x^9 )/((x^5 +x^3 +1)^3 )) dx =?

$$\underset{\mathrm{0}} {\overset{\mathrm{1}} {\int}}\:\frac{\mathrm{2x}^{\mathrm{12}} +\mathrm{5x}^{\mathrm{9}} }{\left(\mathrm{x}^{\mathrm{5}} +\mathrm{x}^{\mathrm{3}} +\mathrm{1}\right)^{\mathrm{3}} }\:\mathrm{dx}\:=?\: \\ $$

Question Number 117637 Answers: 2 Comments: 0

lim_(x→0) (cos x )^(cot x) =?

$$\underset{{x}\rightarrow\mathrm{0}} {\mathrm{lim}}\:\left(\mathrm{cos}\:\mathrm{x}\:\right)^{\mathrm{cot}\:\mathrm{x}} \:=? \\ $$

Question Number 117673 Answers: 4 Comments: 0

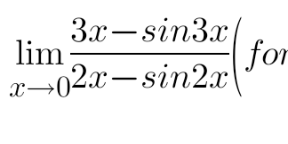
Question Number 117632 Answers: 3 Comments: 0

Solution from 2xy dy = (x^(2 ) − y^2 )dx

$${Solution}\:{from}\:\:\:\mathrm{2}{xy}\:{dy}\:=\:\left({x}^{\mathrm{2}\:} \:−\:{y}^{\mathrm{2}} \right){dx} \\ $$

Question Number 117675 Answers: 2 Comments: 1

Question Number 117620 Answers: 5 Comments: 0

x^4 −⌊5x^2 ⌋+4=0

$${x}^{\mathrm{4}} −\lfloor\mathrm{5}{x}^{\mathrm{2}} \rfloor+\mathrm{4}=\mathrm{0} \\ $$

Question Number 117608 Answers: 1 Comments: 0

solve (d^2 y/dt^2 )+w^2 x=0

$${solve} \\ $$$$\frac{{d}^{\mathrm{2}} {y}}{{dt}^{\mathrm{2}} }+{w}^{\mathrm{2}} {x}=\mathrm{0} \\ $$

Question Number 117606 Answers: 2 Comments: 0

find sina+sin(a+b)+sin(a+2b)++..+sin{a+(n−1)b}

$${find} \\ $$$${sina}+{sin}\left({a}+{b}\right)+{sin}\left({a}+\mathrm{2}{b}\right)++..+{sin}\left\{{a}+\left({n}−\mathrm{1}\right){b}\right\} \\ $$

Question Number 117602 Answers: 2 Comments: 0

(d^2 y/dx^2 )+a^2 y=cosax

$$\frac{{d}^{\mathrm{2}} {y}}{{dx}^{\mathrm{2}} }+{a}^{\mathrm{2}} {y}={cosax} \\ $$

Question Number 117603 Answers: 0 Comments: 0

Let f : R→R be a function satisfying the following : (a) f(−x)=−f(x) (b) f(x+1)=f(x)+1 (c) f((1/x))=((f(x))/x^2 ) for all x≠0 Show that (i)f(x)=x for all x,y∈R (ii) f(x+y)=f(x)+f(y) for all x,y∈R (iii) f(xy)=f(x)f(y) for all x,y∈R (iv) f((x/y))=((f(x))/(f(y))) for all x,y∈R with y≠0

$$\mathrm{Let}\:\mathrm{f}\::\:\mathbb{R}\rightarrow\mathbb{R}\:\mathrm{be}\:\mathrm{a}\:\mathrm{function}\:\mathrm{satisfying}\:\mathrm{the}\:\mathrm{following}\:: \\ $$$$\left(\mathrm{a}\right)\:{f}\left(−{x}\right)=−{f}\left({x}\right) \\ $$$$\left(\mathrm{b}\right)\:{f}\left({x}+\mathrm{1}\right)={f}\left({x}\right)+\mathrm{1} \\ $$$$\left(\mathrm{c}\right)\:{f}\left(\frac{\mathrm{1}}{{x}}\right)=\frac{{f}\left({x}\right)}{{x}^{\mathrm{2}} }\:\mathrm{for}\:\mathrm{all}\:{x}\neq\mathrm{0} \\ $$$$\mathrm{Show}\:\mathrm{that} \\ $$$$\left(\mathrm{i}\right){f}\left({x}\right)={x}\:\mathrm{for}\:\mathrm{all}\:{x},\mathrm{y}\in\mathbb{R} \\ $$$$\left(\mathrm{ii}\right)\:{f}\left({x}+{y}\right)={f}\left({x}\right)+{f}\left(\mathrm{y}\right)\:\mathrm{for}\:\mathrm{all}\:{x},\mathrm{y}\in\mathbb{R} \\ $$$$\left(\mathrm{iii}\right)\:{f}\left({xy}\right)={f}\left({x}\right){f}\left(\mathrm{y}\right)\:\mathrm{for}\:\mathrm{all}\:{x},\mathrm{y}\in\mathbb{R} \\ $$$$\left(\mathrm{iv}\right)\:{f}\left(\frac{{x}}{\mathrm{y}}\right)=\frac{{f}\left({x}\right)}{{f}\left(\mathrm{y}\right)}\:\mathrm{for}\:\mathrm{all}\:{x},\mathrm{y}\in\mathbb{R}\:\mathrm{with}\:\mathrm{y}\neq\mathrm{0} \\ $$

Question Number 117597 Answers: 0 Comments: 1

Let A, B, and C be three sets and X be the set of all elements which belong to exactly two of the sets A,B and C. Prove that X is equal to (A∪B∪C)−[AΔ(BΔC)]

$$\mathrm{Let}\:\mathrm{A},\:\mathrm{B},\:\mathrm{and}\:\mathrm{C}\:\mathrm{be}\:\mathrm{three}\:\mathrm{sets}\:\mathrm{and}\:\mathrm{X}\:\mathrm{be}\:\mathrm{the}\:\mathrm{set}\:\mathrm{of}\:\mathrm{all} \\ $$$$\mathrm{elements}\:\mathrm{which}\:\mathrm{belong}\:\mathrm{to}\:\mathrm{exactly}\:\mathrm{two}\:\mathrm{of}\:\mathrm{the}\:\mathrm{sets}\:\mathrm{A},\mathrm{B} \\ $$$$\mathrm{and}\:\mathrm{C}.\:\mathrm{Prove}\:\mathrm{that}\:\mathrm{X}\:\mathrm{is}\:\mathrm{equal}\:\mathrm{to} \\ $$$$\left(\mathrm{A}\cup\mathrm{B}\cup\mathrm{C}\right)−\left[\mathrm{A}\Delta\left(\mathrm{B}\Delta\mathrm{C}\right)\right] \\ $$

Question Number 117594 Answers: 1 Comments: 0

A rope 5m long is fastened to two hooks 4.0m apart on a horizontal ceiling.to the rope is attached a 10kg mass so that the segments of the rope are 3.0m and 2.0m.compute the tensionin each segment

$${A}\:{rope}\:\mathrm{5}{m}\:{long}\:{is}\:{fastened}\:{to}\:{two}\:{hooks}\: \\ $$$$\mathrm{4}.\mathrm{0}{m}\:{apart}\:{on}\:{a}\:{horizontal} \\ $$$${ceiling}.{to}\:{the}\:{rope}\:{is}\:{attached}\:{a}\:\mathrm{10}{kg}\: \\ $$$${mass}\:{so}\:{that}\:{the}\:{segments}\:{of}\:{the}\:{rope} \\ $$$${are}\:\mathrm{3}.\mathrm{0}{m}\:{and}\:\mathrm{2}.\mathrm{0}{m}.{compute}\:{the} \\ $$$${tensionin}\:{each}\:{segment} \\ $$

Question Number 117585 Answers: 1 Comments: 4

solution (dy/dx) = sin x + e^(2x) + x^2

$${solution}\:\:\:\:\frac{{dy}}{{dx}}\:=\:{sin}\:{x}\:+\:{e}^{\mathrm{2}{x}} \:+\:{x}^{\mathrm{2}} \\ $$

Question Number 117578 Answers: 0 Comments: 1

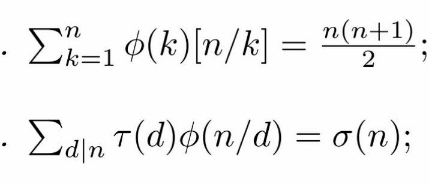
Question Number 117577 Answers: 1 Comments: 0

If f(x), g(x) and h(x) are three functions, where f(x)=2x^5 −8x^2 +1, f(x−3)=g(3x−2) and g(3x+1)=h(x+3), show h(x)=f(x−5).

$$\mathrm{If}\:{f}\left({x}\right),\:{g}\left({x}\right)\:\mathrm{and}\:{h}\left({x}\right)\:\mathrm{are}\:\mathrm{three}\:\mathrm{functions}, \\ $$$$\mathrm{where}\:{f}\left({x}\right)=\mathrm{2}{x}^{\mathrm{5}} −\mathrm{8}{x}^{\mathrm{2}} +\mathrm{1},\:{f}\left({x}−\mathrm{3}\right)={g}\left(\mathrm{3}{x}−\mathrm{2}\right) \\ $$$$\mathrm{and}\:{g}\left(\mathrm{3}{x}+\mathrm{1}\right)={h}\left({x}+\mathrm{3}\right),\:\mathrm{show}\:{h}\left({x}\right)={f}\left({x}−\mathrm{5}\right). \\ $$

Question Number 117574 Answers: 1 Comments: 0

... advanced integral... Evaluate :: I := ∫_0 ^( ∞) (( 4xln(x))/(x^4 +2x^2 +4 ))dx =?? ... m.n.1970..

$$\:\:\:\:\:\:\:\:...\:{advanced}\:\:{integral}... \\ $$$$\:\:\:\:\:\: \\ $$$$\mathscr{E}{valuate}\:::\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{I}\::=\:\int_{\mathrm{0}} ^{\:\infty} \frac{\:\mathrm{4}{xln}\left({x}\right)}{{x}^{\mathrm{4}} +\mathrm{2}{x}^{\mathrm{2}} +\mathrm{4}\:}{dx}\:=??\: \\ $$$$\:\:\:\:\:...\:{m}.{n}.\mathrm{1970}.. \\ $$$$\: \\ $$

Question Number 117568 Answers: 1 Comments: 1

Suppose the non-constant functions f and g satisfy the following two conditions: I: g(x−y)=g(x)g(y)+f(x)f(y) ∀ x,y∈R II: f(0)=0 Evaluate i. g(0) ii.[f(x)]^2 +[g(x)]^2

$$\mathrm{Suppose}\:\mathrm{the}\:\mathrm{non}-\mathrm{constant}\:\mathrm{functions}\:{f}\:\mathrm{and}\:{g} \\ $$$$\mathrm{satisfy}\:\mathrm{the}\:\mathrm{following}\:\mathrm{two}\:\mathrm{conditions}: \\ $$$$\mathrm{I}:\:{g}\left({x}−{y}\right)={g}\left({x}\right){g}\left({y}\right)+{f}\left({x}\right){f}\left({y}\right)\:\forall\:{x},{y}\in\mathbb{R} \\ $$$$\mathrm{II}:\:{f}\left(\mathrm{0}\right)=\mathrm{0} \\ $$$$\mathrm{Evaluate} \\ $$$$\mathrm{i}.\:{g}\left(\mathrm{0}\right)\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{ii}.\left[{f}\left({x}\right)\right]^{\mathrm{2}} +\left[{g}\left({x}\right)\right]^{\mathrm{2}} \\ $$

Question Number 117567 Answers: 1 Comments: 0

give A={a,b,c,d,e}; n(p(A))=?

$${give}\:{A}=\left\{{a},{b},{c},{d},{e}\right\};\:{n}\left({p}\left({A}\right)\right)=? \\ $$$$\:\:\: \\ $$

Question Number 117557 Answers: 1 Comments: 0

second derivative x^2 +3y^2 =5

$$\mathrm{second}\:\mathrm{derivative} \\ $$$${x}^{\mathrm{2}} +\mathrm{3}{y}^{\mathrm{2}} =\mathrm{5} \\ $$

Question Number 117555 Answers: 2 Comments: 0

Alternative forms { (((√x)+(√y)=((23)/(12)))),((9x+16y=29)) :}

$${Alternative}\:{forms} \\ $$$$\begin{cases}{\sqrt{{x}}+\sqrt{{y}}=\frac{\mathrm{23}}{\mathrm{12}}}\\{\mathrm{9}{x}+\mathrm{16}{y}=\mathrm{29}}\end{cases} \\ $$$$ \\ $$

Question Number 117552 Answers: 2 Comments: 1

please help cos (π/7) . cos ((2π)/7) . cos ((4π)/7) = ?