Question and Answers Forum

AllQuestion and Answers: Page 1004

Question Number 116767 Answers: 0 Comments: 1

if A×B=0 how to prove A=0 or B=0

$${if}\:\:{A}×{B}=\mathrm{0}\:{how}\:{to}\:{prove}\:{A}=\mathrm{0}\:{or}\:{B}=\mathrm{0} \\ $$

Question Number 116756 Answers: 2 Comments: 0

lim_(x→2) (((x−2))^(1/(3 )) /(x−2)) =?

$$\:\underset{{x}\rightarrow\mathrm{2}} {\mathrm{lim}}\:\frac{\sqrt[{\mathrm{3}\:}]{\mathrm{x}−\mathrm{2}}}{\mathrm{x}−\mathrm{2}}\:=? \\ $$

Question Number 116753 Answers: 1 Comments: 0

if 4^x =8x find x

$${if}\:\mathrm{4}^{{x}} =\mathrm{8}{x}\:{find}\:{x} \\ $$

Question Number 116746 Answers: 1 Comments: 0

Find an orthogonal matrix A whose first row is u_1 = ((1/3), (2/3), (2/3)).

$$\mathrm{Find}\:\mathrm{an}\:\mathrm{orthogonal}\:\mathrm{matrix}\:\mathrm{A}\:\mathrm{whose} \\ $$$$\mathrm{first}\:\mathrm{row}\:\mathrm{is}\:\mathrm{u}_{\mathrm{1}} =\:\left(\frac{\mathrm{1}}{\mathrm{3}},\:\frac{\mathrm{2}}{\mathrm{3}},\:\frac{\mathrm{2}}{\mathrm{3}}\right). \\ $$

Question Number 116744 Answers: 4 Comments: 0

... (( nice)/(calculus)) ... prove that :: ∫_(−1) ^( ∞) (e^(−4x) /( (√(x+1)))) dx =((√π)/2) e^4 ... m.n .1970...

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:...\:\frac{\:{nice}}{{calculus}}\:... \\ $$$$\:\:{prove}\:\:{that}\::: \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\int_{−\mathrm{1}} ^{\:\infty} \frac{{e}^{−\mathrm{4}{x}} }{\:\sqrt{{x}+\mathrm{1}}}\:{dx}\:=\frac{\sqrt{\pi}}{\mathrm{2}}\:{e}^{\mathrm{4}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...\:{m}.{n}\:.\mathrm{1970}... \\ $$$$ \\ $$

Question Number 116742 Answers: 0 Comments: 0

the curve y=f(x) is rotated about the x−axis to form solid.the volume of this solid is 0.5π(a−2sina cosa) for the limit of 0≤x≤a. find the value of a

$${the}\:{curve}\:{y}={f}\left({x}\right)\:{is}\:{rotated}\:{about}\:{the} \\ $$$${x}−{axis}\:{to}\:{form}\:{solid}.{the}\:{volume}\:{of}\:{this} \\ $$$${solid}\:{is}\:\mathrm{0}.\mathrm{5}\pi\left({a}−\mathrm{2}{sina}\:{cosa}\right)\:{for}\:{the}\:{limit} \\ $$$${of}\:\mathrm{0}\leqslant{x}\leqslant{a}.\:{find}\:{the}\:{value}\:{of}\:{a} \\ $$$$ \\ $$

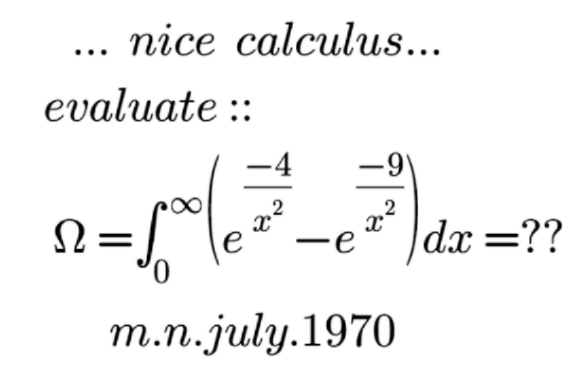
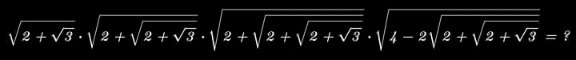
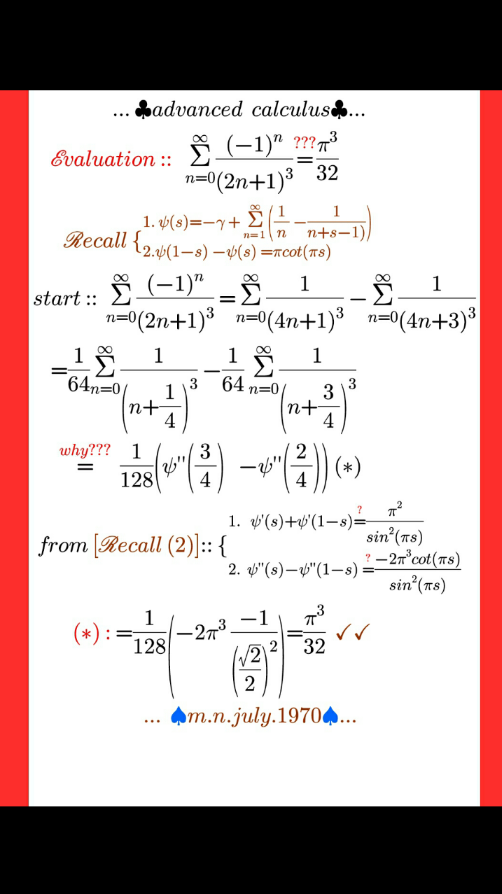
Question Number 116738 Answers: 1 Comments: 1

determine the area of the region bounded by y=(2x+6)^(0.5 ) and y=x−1

$${determine}\:{the}\:{area}\:{of}\:{the}\:{region}\:{bounded} \\ $$$${by}\:{y}=\left(\mathrm{2}{x}+\mathrm{6}\right)^{\mathrm{0}.\mathrm{5}\:} {and}\:{y}={x}−\mathrm{1} \\ $$

Question Number 116737 Answers: 1 Comments: 0

find the lenght of the function y=sinx for 0<x<π

$${find}\:{the}\:{lenght}\:{of}\:{the}\:{function}\:{y}={sinx}\: \\ $$$${for}\:\mathrm{0}<{x}<\pi \\ $$$$ \\ $$

Question Number 116710 Answers: 2 Comments: 0

Question Number 116701 Answers: 2 Comments: 2

∫ (dx/(x+x(√x))) =?

$$\int\:\frac{\mathrm{dx}}{\mathrm{x}+\mathrm{x}\sqrt{\mathrm{x}}}\:=? \\ $$

Question Number 116700 Answers: 1 Comments: 0

How many positive integral solutions does 3x+5y=300 have?

$$\mathrm{How}\:\mathrm{many}\:\mathrm{positive}\:\mathrm{integral}\: \\ $$$$\mathrm{solutions}\:\mathrm{does}\:\mathrm{3x}+\mathrm{5y}=\mathrm{300}\:\mathrm{have}? \\ $$

Question Number 116695 Answers: 2 Comments: 0

Solving by Gaussian elimination using the following system of linear equation { ((x−3y−2z=6)),((2x−4y−3z=8)),((−3x+6y+8z=−5)) :}

$$\mathrm{Solving}\:\mathrm{by}\:\mathrm{Gaussian}\:\mathrm{elimination} \\ $$$$\mathrm{using}\:\mathrm{the}\:\mathrm{following}\:\mathrm{system}\:\mathrm{of} \\ $$$$\mathrm{linear}\:\mathrm{equation}\:\begin{cases}{\mathrm{x}−\mathrm{3y}−\mathrm{2z}=\mathrm{6}}\\{\mathrm{2x}−\mathrm{4y}−\mathrm{3z}=\mathrm{8}}\\{−\mathrm{3x}+\mathrm{6y}+\mathrm{8z}=−\mathrm{5}}\end{cases} \\ $$

Question Number 116687 Answers: 2 Comments: 0

Help please, to solve this ... If f(x)=1+x^2 for x∈[−2,2] and f(x)=5 otherwise. Then what is the value of ∫_(−2) ^(+2) f(2x^2 )dx?

$${Help}\:{please},\:{to}\:{solve}\:{this}\:... \\ $$$${If}\:{f}\left({x}\right)=\mathrm{1}+{x}^{\mathrm{2}} \:\:{for}\:{x}\in\left[−\mathrm{2},\mathrm{2}\right]\:{and}\: \\ $$$$\:\:\:\:\:\:{f}\left({x}\right)=\mathrm{5}\:\:\:\:\:\:\:\:{otherwise}. \\ $$$${Then}\:{what}\:{is}\:{the}\:{value}\:{of} \\ $$$$\int_{−\mathrm{2}} ^{+\mathrm{2}} {f}\left(\mathrm{2}{x}^{\mathrm{2}} \right){dx}? \\ $$$$ \\ $$

Question Number 116685 Answers: 1 Comments: 0

Given the equality: 1+3+5+...+(2p+1)=(p+1)^(2 ) p ∈ N^∗ Show this equality is true when we replace p by p+1

$$\mathrm{Given}\:\mathrm{the}\:\mathrm{equality}: \\ $$$$\mathrm{1}+\mathrm{3}+\mathrm{5}+...+\left(\mathrm{2p}+\mathrm{1}\right)=\left(\mathrm{p}+\mathrm{1}\right)^{\mathrm{2}\:} \:\:\:\mathrm{p}\:\in\:\mathbb{N}^{\ast} \\ $$$$ \\ $$$$\mathrm{Show}\:\mathrm{this}\:\mathrm{equality}\:\mathrm{is}\:\mathrm{true}\:\mathrm{when} \\ $$$$\mathrm{we}\:\mathrm{replace}\:\mathrm{p}\:\mathrm{by}\:\mathrm{p}+\mathrm{1} \\ $$

Question Number 116683 Answers: 2 Comments: 0

Given 1+3+5+7=16 we know that 16=4^2 and 4 is the half of 8 which is the successor of 7. conjecture the result of this sum: 1+3+5+7+...+25

$$\mathrm{Given}\:\mathrm{1}+\mathrm{3}+\mathrm{5}+\mathrm{7}=\mathrm{16}\:\:\:\mathrm{we}\:\mathrm{know}\:\mathrm{that} \\ $$$$\mathrm{16}=\mathrm{4}^{\mathrm{2}} \:\:\mathrm{and}\:\mathrm{4}\:\mathrm{is}\:\mathrm{the}\:\mathrm{half}\:\mathrm{of}\:\mathrm{8}\:\mathrm{which}\:\mathrm{is}\:\mathrm{the}\: \\ $$$$\mathrm{successor}\:\mathrm{of}\:\mathrm{7}. \\ $$$$ \\ $$$$\mathrm{conjecture}\:\mathrm{the}\:\mathrm{result}\:\mathrm{of}\:\mathrm{this}\:\mathrm{sum}: \\ $$$$\mathrm{1}+\mathrm{3}+\mathrm{5}+\mathrm{7}+...+\mathrm{25} \\ $$

Question Number 116674 Answers: 2 Comments: 1

Question Number 116672 Answers: 2 Comments: 0

... nice calculus ... prove that : I = ∫_0 ^( 1) ((π/4) −Arctan(x))(dx/(1−x^2 )) = (G/2) ✓ G is catalan constant ... M.N.1970

$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...\:\:\:{nice}\:\:{calculus}\:... \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:{prove}\:\:{that}\:: \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\mathrm{I}\:=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \left(\frac{\pi}{\mathrm{4}}\:\:−\mathscr{A}{rctan}\left({x}\right)\right)\frac{{dx}}{\mathrm{1}−{x}^{\mathrm{2}} }\:=\:\frac{\mathrm{G}}{\mathrm{2}}\:\:\checkmark\:\:\:\: \\ $$$$\:\:\:\:\:\:\: \\ $$$$\mathrm{G}\:{is}\:\:\:{catalan}\:\:{constant}\:... \\ $$$$\:\:\:\:\:\:\:\mathscr{M}.\mathscr{N}.\mathrm{1970} \\ $$$$ \\ $$$$ \\ $$$$\:\:\: \\ $$

Question Number 116669 Answers: 1 Comments: 1

Question Number 116667 Answers: 1 Comments: 0

... nice calculus... very nice integral:: demonstrate::: Ω = ∫_0 ^( 1) ((1−x)/((1+x+x^2 +x^3 )log(x))) dx=^(???) log((√(1/2))) .m.n.1970.

$$\:\:\:\:\:\:\:\:\:\:\:\:...\:\:\:{nice}\:\:{calculus}... \\ $$$$ \\ $$$$\:\:\:\:\:\:{very}\:{nice}\:\:{integral}:: \\ $$$$\:\:\:\:\:\:\:{demonstrate}::: \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\Omega\:=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} \frac{\mathrm{1}−{x}}{\left(\mathrm{1}+{x}+{x}^{\mathrm{2}} +{x}^{\mathrm{3}} \right){log}\left({x}\right)}\:{dx}\overset{???} {=}{log}\left(\sqrt{\frac{\mathrm{1}}{\mathrm{2}}}\right) \\ $$$$\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:.{m}.{n}.\mathrm{1970}. \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\: \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\: \\ $$

Question Number 116666 Answers: 1 Comments: 0

Question Number 116763 Answers: 0 Comments: 1

∫(dx/( ((1+x^3 ))^(1/3) ))=?

$$ \\ $$$$\:\:\:\:\:\int\frac{{dx}}{\:\sqrt[{\mathrm{3}}]{\mathrm{1}+{x}^{\mathrm{3}} }}=? \\ $$

Question Number 116759 Answers: 2 Comments: 0

Question Number 116658 Answers: 2 Comments: 2

Question Number 116717 Answers: 2 Comments: 0

∫xdx

$$\int{xdx} \\ $$

Question Number 116725 Answers: 4 Comments: 3

what the value of log _(10) (−1) in complex number

$$\mathrm{what}\:\mathrm{the}\:\mathrm{value}\:\mathrm{of}\:\mathrm{log}\:_{\mathrm{10}} \left(−\mathrm{1}\right)\:\mathrm{in}\: \\ $$$$\mathrm{complex}\:\mathrm{number} \\ $$

Question Number 116722 Answers: 1 Comments: 0

find the range of values of k for which the equation e^x −5=k has no solution

$${find}\:{the}\:{range}\:{of}\:{values}\:{of}\:{k}\:{for} \\ $$$${which}\:{the}\:{equation}\:{e}^{{x}} −\mathrm{5}={k}\:{has}\:{no} \\ $$$${solution} \\ $$

Pg 999 Pg 1000 Pg 1001 Pg 1002 Pg 1003 Pg 1004 Pg 1005 Pg 1006 Pg 1007 Pg 1008

Contact: info@tinkutara.com