Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1002

Question Number 118094 Answers: 1 Comments: 0

∫ (dx/(sec x+2)) =?

$$\int\:\frac{\mathrm{dx}}{\mathrm{sec}\:\mathrm{x}+\mathrm{2}}\:=? \\ $$

Question Number 118065 Answers: 1 Comments: 0

Question Number 118064 Answers: 1 Comments: 0

Question Number 118062 Answers: 0 Comments: 2

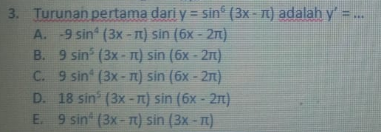
Question Number 118030 Answers: 2 Comments: 1

find ∫_0 ^∞ ((x^2 −2)/(x^4 +x^2 +1))dx

$$\mathrm{find}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{x}^{\mathrm{2}} −\mathrm{2}}{\mathrm{x}^{\mathrm{4}} +\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}\mathrm{dx} \\ $$

Question Number 118043 Answers: 1 Comments: 2

How many numbers in the range [1,10^n −1] are divisible by 9? digit repetitions are not allowed.

$$\mathrm{How}\:\mathrm{many}\:\mathrm{numbers}\:\mathrm{in}\:\mathrm{the}\:\mathrm{range} \\ $$$$\left[\mathrm{1},\mathrm{10}^{{n}} −\mathrm{1}\right]\:\mathrm{are}\:\mathrm{divisible}\:\mathrm{by}\:\mathrm{9}? \\ $$$$\mathrm{digit}\:\mathrm{repetitions}\:\mathrm{are}\:\mathrm{not}\:\mathrm{allowed}. \\ $$

Question Number 118040 Answers: 2 Comments: 0

Question Number 118023 Answers: 1 Comments: 0

..calculus.. x,y,z ∈R^+ and x^2 +y^2 +z^2 =1 find min_(x,y,z∈R^(+ ) ) ((((yz)/x)+((xz)/y)+((xy)/z)) )=? m.n.1970..

$$ \\ $$$$\:\:\:\:\:\:\:..{calculus}.. \\ $$$$\:\:{x},{y},{z}\:\in\mathbb{R}^{+} \:\:{and}\:\:{x}^{\mathrm{2}} +{y}^{\mathrm{2}} +{z}^{\mathrm{2}} \:=\mathrm{1} \\ $$$$\:\:\:\: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:{find}\:\:\:\:\:\: \\ $$$$\:\:\:\:{min}_{{x},{y},{z}\in\mathbb{R}^{+\:\:\:\:} } \left(\left(\frac{{yz}}{{x}}+\frac{{xz}}{{y}}+\frac{{xy}}{{z}}\right)\:\right)=? \\ $$$$ \\ $$$$\:\:\:\:\:\:\:{m}.{n}.\mathrm{1970}.. \\ $$

Question Number 118019 Answers: 2 Comments: 3

How many n digit integers are divisible by 9?

$$\mathrm{How}\:\mathrm{many}\:{n}\:\mathrm{digit}\:\mathrm{integers}\:\mathrm{are} \\ $$$$\mathrm{divisible}\:\mathrm{by}\:\mathrm{9}? \\ $$

Question Number 118017 Answers: 0 Comments: 0

Question Number 118011 Answers: 4 Comments: 0

Question Number 118010 Answers: 3 Comments: 1

∫(dx/(x^4 +x^2 +1))

$$\int\frac{{dx}}{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{1}} \\ $$

Question Number 118005 Answers: 0 Comments: 1

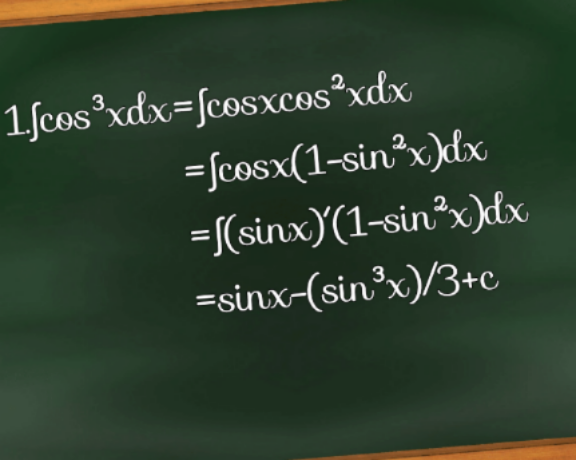
Question Number 118004 Answers: 2 Comments: 0

find value of tan46^0 using calculus

$${find}\:\:{value}\:{of}\:{tan}\mathrm{46}^{\mathrm{0}} \:{using}\:{calculus} \\ $$

Question Number 118002 Answers: 1 Comments: 0

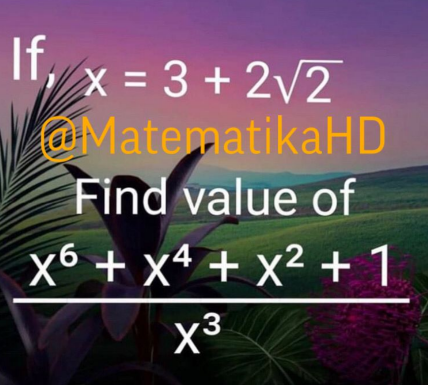
Question Number 117989 Answers: 1 Comments: 0

Question Number 117990 Answers: 1 Comments: 0

(2.3.5.7.9.11.13.17......∞)×(√(6/(3.8.24.48.80.120.168.288.....∞)))

$$\left(\mathrm{2}.\mathrm{3}.\mathrm{5}.\mathrm{7}.\mathrm{9}.\mathrm{11}.\mathrm{13}.\mathrm{17}......\infty\right)×\sqrt{\frac{\mathrm{6}}{\mathrm{3}.\mathrm{8}.\mathrm{24}.\mathrm{48}.\mathrm{80}.\mathrm{120}.\mathrm{168}.\mathrm{288}.....\infty}} \\ $$

Question Number 117984 Answers: 1 Comments: 0

If f(x) is a polynomial function satisfying the relation f(x)+f((1/x))=f(x)f((1/x)) for all 0≠x∈R and if f(2)=9, then f(6) is (A) 216 (B) 217 (C) 126 (D) 127

$$\mathrm{If}\:{f}\left({x}\right)\:\mathrm{is}\:\mathrm{a}\:\mathrm{polynomial}\:\mathrm{function}\:\mathrm{satisfying}\:\mathrm{the}\:\mathrm{relation} \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:{f}\left({x}\right)+{f}\left(\frac{\mathrm{1}}{{x}}\right)={f}\left({x}\right){f}\left(\frac{\mathrm{1}}{{x}}\right) \\ $$$$\mathrm{for}\:\mathrm{all}\:\mathrm{0}\neq{x}\in\mathbb{R}\:\mathrm{and}\:\mathrm{if}\:{f}\left(\mathrm{2}\right)=\mathrm{9},\:\mathrm{then}\:\mathrm{f}\left(\mathrm{6}\right)\:\mathrm{is} \\ $$$$\left(\mathrm{A}\right)\:\mathrm{216}\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\mathrm{217}\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:\mathrm{126}\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\mathrm{127} \\ $$

Question Number 117980 Answers: 0 Comments: 2

Where is the quiz?

$$\mathrm{Where}\:\mathrm{is}\:\mathrm{the}\:\mathrm{quiz}? \\ $$$$ \\ $$

Question Number 117979 Answers: 1 Comments: 1

... nice calculus... prove that: ∣ Γ ( i ) ∣=^? (√(π/(sinh(π)))) Γ: Euler gamma function ...m.n.july.1970...

$$\:\:\:\:\:\:\:\:\:\:...\:{nice}\:\:{calculus}... \\ $$$$\:\:\:\:\:{prove}\:\:{that}: \\ $$$$\:\:\:\:\:\:\:\:\:\:\mid\:\:\Gamma\:\left(\:{i}\:\right)\:\mid\overset{?} {=}\:\sqrt{\frac{\pi}{{sinh}\left(\pi\right)}} \\ $$$$\:\:\:\:\:\:\:\:\:\:\Gamma:\:\mathscr{E}{uler}\:{gamma}\:{function}\:\: \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:...{m}.{n}.{july}.\mathrm{1970}... \\ $$

Question Number 117973 Answers: 1 Comments: 0

consider a non−singular 2×2 square matrix T. If trace (T) =4 and trace (T^2 )=5 what is determinant of the matrix T ?

$$\mathrm{consider}\:\mathrm{a}\:\mathrm{non}−\mathrm{singular}\:\mathrm{2}×\mathrm{2}\: \\ $$$$\mathrm{square}\:\mathrm{matrix}\:\mathrm{T}.\:\mathrm{If}\:\mathrm{trace}\:\left(\mathrm{T}\right)\:=\mathrm{4} \\ $$$$\mathrm{and}\:\mathrm{trace}\:\left(\mathrm{T}^{\mathrm{2}} \right)=\mathrm{5}\:\mathrm{what}\:\mathrm{is}\:\mathrm{determinant} \\ $$$$\mathrm{of}\:\mathrm{the}\:\mathrm{matrix}\:\mathrm{T}\:? \\ $$

Question Number 117972 Answers: 2 Comments: 0

The number of surjections of {1,2,3,4} onto {x,y} is (A) 16 (B) 8 (C) 14 (D) 6

$$\mathrm{The}\:\mathrm{number}\:\mathrm{of}\:\mathrm{surjections}\:\mathrm{of}\:\left\{\mathrm{1},\mathrm{2},\mathrm{3},\mathrm{4}\right\}\:\mathrm{onto}\:\left\{\mathrm{x},\mathrm{y}\right\}\:\mathrm{is} \\ $$$$\left(\mathrm{A}\right)\:\mathrm{16}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{B}\right)\:\mathrm{8}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{C}\right)\:\mathrm{14}\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\left(\mathrm{D}\right)\:\mathrm{6} \\ $$

Question Number 117963 Answers: 2 Comments: 0

Question Number 117960 Answers: 1 Comments: 0

Question Number 117948 Answers: 4 Comments: 0

... nice integral... please evaluate :: I =∫_0 ^( 1) (sin(x)+sin((1/x)))(dx/x) =?? m.n.1970

$$\:\:\:\:\:\:\:\:...\:\:{nice}\:\:{integral}...\: \\ $$$$\:\:\:{please}\:{evaluate}\::: \\ $$$$ \\ $$$$\:\:\:\:\mathrm{I}\:=\int_{\mathrm{0}} ^{\:\mathrm{1}} \left({sin}\left({x}\right)+{sin}\left(\frac{\mathrm{1}}{{x}}\right)\right)\frac{{dx}}{{x}}\:=?? \\ $$$$ \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:{m}.{n}.\mathrm{1970} \\ $$$$\:\: \\ $$

Question Number 117945 Answers: 3 Comments: 1

f(x) = ∫ ((5x^8 +7x^6 )/((2x^7 +x^2 +1)^2 )) dx and f(0) = 0 , then f(1) = _

$$\mathrm{f}\left(\mathrm{x}\right)\:=\:\int\:\frac{\mathrm{5x}^{\mathrm{8}} +\mathrm{7x}^{\mathrm{6}} }{\left(\mathrm{2x}^{\mathrm{7}} +\mathrm{x}^{\mathrm{2}} +\mathrm{1}\right)^{\mathrm{2}} }\:\mathrm{dx}\:\mathrm{and}\:\: \\ $$$$\mathrm{f}\left(\mathrm{0}\right)\:=\:\mathrm{0}\:,\:\mathrm{then}\:\mathrm{f}\left(\mathrm{1}\right)\:=\:\_\: \\ $$

Pg 997 Pg 998 Pg 999 Pg 1000 Pg 1001 Pg 1002 Pg 1003 Pg 1004 Pg 1005 Pg 1006

Contact: info@tinkutara.com