Question and Answers Forum

AllQuestion and Answers: Page 1001

Question Number 113604 Answers: 0 Comments: 0

if A={1,2} and let R={(a,b)∈A×A:a=3b} then R= ? help me sir

$${if}\:{A}=\left\{\mathrm{1},\mathrm{2}\right\}\:{and}\:{let}\:{R}=\left\{\left({a},{b}\right)\in{A}×{A}:{a}=\mathrm{3}{b}\right\}\: \\ $$$${then}\:{R}=\:? \\ $$$$ \\ $$$${help}\:{me}\:{sir} \\ $$

Question Number 113601 Answers: 1 Comments: 0

Question Number 113600 Answers: 1 Comments: 0

Prouver que β(a,b)=((Γ(a)Γ(b))/(Γ(a+b)))=∫_0 ^1 x^(a−1) (1−x)^(b−1) dx

$${Prouver}\:{que} \\ $$$$\beta\left({a},{b}\right)=\frac{\Gamma\left({a}\right)\Gamma\left({b}\right)}{\Gamma\left({a}+{b}\right)}=\int_{\mathrm{0}} ^{\mathrm{1}} {x}^{{a}−\mathrm{1}} \left(\mathrm{1}−{x}\right)^{{b}−\mathrm{1}} {dx} \\ $$

Question Number 113593 Answers: 2 Comments: 1

(6/(5×14))+(6/(14×23))+(6/(23×32))+...+(6/((9n−4)(9n+5)))=?

$$\frac{\mathrm{6}}{\mathrm{5}×\mathrm{14}}+\frac{\mathrm{6}}{\mathrm{14}×\mathrm{23}}+\frac{\mathrm{6}}{\mathrm{23}×\mathrm{32}}+...+\frac{\mathrm{6}}{\left(\mathrm{9}{n}−\mathrm{4}\right)\left(\mathrm{9}{n}+\mathrm{5}\right)}=? \\ $$

Question Number 113598 Answers: 1 Comments: 0

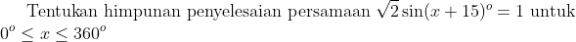
Question Number 113589 Answers: 2 Comments: 0

prove the following integral ∫_0 ^(π/2) (x^2 /(sinx))dx=2πG−(7/2)ζ(3) ∫_0 ^1 ln[((x+(√(1−x^2 )))/(x−(√(1−x^2 ))))]^2 (x/(1−x^2 ))dx=((.π^2 )/2) ∫_0 ^∞ ((e^x lnx)/(1+e^(2x) ))dx=(π/2)ln[((Γ((3/4)))/(Γ((1/4))))(√(2π))]

$${prove}\:{the}\:{following}\:{integral} \\ $$$$\int_{\mathrm{0}} ^{\frac{\pi}{\mathrm{2}}} \frac{{x}^{\mathrm{2}} }{\mathrm{sin}{x}}{dx}=\mathrm{2}\pi{G}−\frac{\mathrm{7}}{\mathrm{2}}\zeta\left(\mathrm{3}\right) \\ $$$$\int_{\mathrm{0}} ^{\mathrm{1}} \mathrm{ln}\left[\frac{{x}+\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}{{x}−\sqrt{\mathrm{1}−{x}^{\mathrm{2}} }}\right]^{\mathrm{2}} \frac{{x}}{\mathrm{1}−{x}^{\mathrm{2}} }{dx}=\frac{.\pi^{\mathrm{2}} }{\mathrm{2}} \\ $$$$\int_{\mathrm{0}} ^{\infty} \frac{{e}^{{x}} \mathrm{ln}{x}}{\mathrm{1}+{e}^{\mathrm{2}{x}} }{dx}=\frac{\pi}{\mathrm{2}}\mathrm{ln}\left[\frac{\Gamma\left(\frac{\mathrm{3}}{\mathrm{4}}\right)}{\Gamma\left(\frac{\mathrm{1}}{\mathrm{4}}\right)}\sqrt{\mathrm{2}\pi}\right] \\ $$

Question Number 113587 Answers: 2 Comments: 1

Question Number 113628 Answers: 1 Comments: 0

find ∫ (dx/((x+1)(√(x^2 −1))+(x−1)(√(x^2 +1))))

$$\mathrm{find}\:\int\:\:\frac{\mathrm{dx}}{\left(\mathrm{x}+\mathrm{1}\right)\sqrt{\mathrm{x}^{\mathrm{2}} −\mathrm{1}}+\left(\mathrm{x}−\mathrm{1}\right)\sqrt{\mathrm{x}^{\mathrm{2}} \:+\mathrm{1}}} \\ $$

Question Number 113627 Answers: 0 Comments: 0

calculate ∫_0 ^∞ (dx/(x^4 +ix^2 +2))

$$\mathrm{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{4}} +\mathrm{ix}^{\mathrm{2}} \:+\mathrm{2}} \\ $$

Question Number 113578 Answers: 1 Comments: 2

Question Number 113576 Answers: 1 Comments: 0

lim_(r→∞) (√(4r^2 +2r)) −((8r^3 +4r^2 ))^(1/(3 )) =?

$$\:\:\:\underset{{r}\rightarrow\infty} {\mathrm{lim}}\:\sqrt{\mathrm{4}{r}^{\mathrm{2}} +\mathrm{2}{r}}\:−\sqrt[{\mathrm{3}\:}]{\mathrm{8}{r}^{\mathrm{3}} +\mathrm{4}{r}^{\mathrm{2}} }\:=? \\ $$

Question Number 113565 Answers: 1 Comments: 0

Question Number 113564 Answers: 1 Comments: 0

∫_1 ^2 f(2x+1) dx = 3 and ∫_1 ^2 (x^2 f(x^3 +2))dx = 5 then ∫_5 ^(10) f(x) dx = ?

$$\:\:\int_{\mathrm{1}} ^{\mathrm{2}} {f}\left(\mathrm{2}{x}+\mathrm{1}\right)\:{dx}\:=\:\mathrm{3}\:{and}\: \\ $$$$\:\:\int_{\mathrm{1}} ^{\mathrm{2}} \left({x}^{\mathrm{2}} \:{f}\left({x}^{\mathrm{3}} +\mathrm{2}\right)\right){dx}\:=\:\mathrm{5} \\ $$$${then}\:\int_{\mathrm{5}} ^{\mathrm{10}} {f}\left({x}\right)\:{dx}\:=\:? \\ $$

Question Number 113562 Answers: 1 Comments: 0

calculate ∫_0 ^∞ (dx/(x^4 +ix^2 +2))

$$\mathrm{calculate}\:\int_{\mathrm{0}} ^{\infty} \:\frac{\mathrm{dx}}{\mathrm{x}^{\mathrm{4}} +\mathrm{ix}^{\mathrm{2}} \:+\mathrm{2}} \\ $$

Question Number 113554 Answers: 2 Comments: 0

Using displacement vector (((−4)),((−2)) ), what is the image of ((6),(3) ) when translated?

$$\mathrm{Using}\:\mathrm{displacement}\:\mathrm{vector}\:\begin{pmatrix}{−\mathrm{4}}\\{−\mathrm{2}}\end{pmatrix}, \\ $$$$\mathrm{what}\:\mathrm{is}\:\mathrm{the}\:\mathrm{image}\:\mathrm{of}\:\begin{pmatrix}{\mathrm{6}}\\{\mathrm{3}}\end{pmatrix}\:\mathrm{when} \\ $$$$\mathrm{translated}? \\ $$

Question Number 113552 Answers: 1 Comments: 0

Question Number 113549 Answers: 0 Comments: 0

let A = (((1 −(1/n))),(((1/n) 1)) ) 1) calculate A^2 2) calculate A^m (m integr natural) 3) coclude A^n and lim_(n→+∞) A^n 4) calculate e^A and e^(−A)

$$\mathrm{let}\:\mathrm{A}\:\:=\begin{pmatrix}{\mathrm{1}\:\:\:\:\:\:\:\:−\frac{\mathrm{1}}{\mathrm{n}}}\\{\frac{\mathrm{1}}{\mathrm{n}}\:\:\:\:\:\mathrm{1}}\end{pmatrix} \\ $$$$\left.\mathrm{1}\right)\:\mathrm{calculate}\:\mathrm{A}^{\mathrm{2}} \\ $$$$\left.\mathrm{2}\right)\:\mathrm{calculate}\:\:\mathrm{A}^{\mathrm{m}} \:\:\:\:\:\left(\mathrm{m}\:\:\mathrm{integr}\:\mathrm{natural}\right) \\ $$$$\left.\mathrm{3}\right)\:\mathrm{coclude}\:\mathrm{A}^{\mathrm{n}} \:\:\:\mathrm{and}\:\mathrm{lim}_{\mathrm{n}\rightarrow+\infty} \:\mathrm{A}^{\mathrm{n}} \\ $$$$\left.\mathrm{4}\right)\:\mathrm{calculate}\:\mathrm{e}^{\mathrm{A}} \:\mathrm{and}\:\mathrm{e}^{−\mathrm{A}} \\ $$

Question Number 113542 Answers: 0 Comments: 2

App Update v2.203 A function plotter for one variable is available. Steps to use Insert Drawing Insert Equation select equation from the list of shape tap on BUILD and select plot A sample plot is show as image below. Line color for plot can be changed using edit shape menu to change range of x values select scale from options menu. to zoomin/out use option menu to scroll with zoom: clear selection and place touch in move mode. You can add multiple plots on one drawing and also add another drawing on the canvas.

$$\mathrm{App}\:\mathrm{Update}\:\mathrm{v2}.\mathrm{203} \\ $$$$\mathrm{A}\:\mathrm{function}\:\mathrm{plotter}\:\mathrm{for}\:\mathrm{one}\:\mathrm{variable} \\ $$$$\mathrm{is}\:\mathrm{available}.\:\mathrm{Steps}\:\mathrm{to}\:\mathrm{use} \\ $$$$\mathrm{Insert}\:\mathrm{Drawing} \\ $$$$\mathrm{Insert}\:\mathrm{Equation} \\ $$$$\mathrm{select}\:\mathrm{equation}\:\mathrm{from}\:\mathrm{the}\:\mathrm{list}\:\mathrm{of}\:\mathrm{shape} \\ $$$$\mathrm{tap}\:\mathrm{on}\:\mathrm{BUILD}\:\:\mathrm{and}\:\mathrm{select}\:\mathrm{plot} \\ $$$$\mathrm{A}\:\mathrm{sample}\:\mathrm{plot}\:\mathrm{is}\:\mathrm{show}\:\mathrm{as}\:\mathrm{image}\:\mathrm{below}. \\ $$$$\mathrm{Line}\:\mathrm{color}\:\mathrm{for}\:\mathrm{plot}\:\mathrm{can}\:\mathrm{be}\:\mathrm{changed} \\ $$$$\mathrm{using}\:\mathrm{edit}\:\mathrm{shape}\:\mathrm{menu} \\ $$$$\mathrm{to}\:\mathrm{change}\:\mathrm{range}\:\mathrm{of}\:\mathrm{x}\:\mathrm{values}\:\mathrm{select} \\ $$$$\mathrm{scale}\:\mathrm{from}\:\mathrm{options}\:\mathrm{menu}. \\ $$$$\mathrm{to}\:\mathrm{zoomin}/\mathrm{out}\:\mathrm{use}\:\mathrm{option}\:\mathrm{menu} \\ $$$$\mathrm{to}\:\mathrm{scroll}\:\mathrm{with}\:\mathrm{zoom}:\:\mathrm{clear}\:\mathrm{selection}\:\mathrm{and} \\ $$$$\mathrm{place}\:\mathrm{touch}\:\mathrm{in}\:\mathrm{move}\:\mathrm{mode}. \\ $$$$\mathrm{You}\:\mathrm{can}\:\mathrm{add}\:\mathrm{multiple}\:\mathrm{plots}\:\mathrm{on} \\ $$$$\mathrm{one}\:\mathrm{drawing}\:\mathrm{and}\:\mathrm{also}\:\mathrm{add}\:\mathrm{another} \\ $$$$\mathrm{drawing}\:\mathrm{on}\:\mathrm{the}\:\mathrm{canvas}. \\ $$

Question Number 113532 Answers: 2 Comments: 1

Question Number 113530 Answers: 2 Comments: 0

Question Number 113524 Answers: 2 Comments: 0

Question Number 113511 Answers: 1 Comments: 0

Question Number 113510 Answers: 1 Comments: 0

Question Number 113508 Answers: 1 Comments: 2

Question Number 113507 Answers: 2 Comments: 0

Question Number 113505 Answers: 1 Comments: 0

Pg 996 Pg 997 Pg 998 Pg 999 Pg 1000 Pg 1001 Pg 1002 Pg 1003 Pg 1004 Pg 1005

Contact: info@tinkutara.com