Question and Answers Forum

All Questions Topic List

AllQuestion and Answers: Page 1000

Question Number 119376 Answers: 3 Comments: 2

Solve for x in the equation below ax^2 +bx + c = 0.

$${Solve}\:{for}\:\boldsymbol{{x}}\:{in}\:{the}\:{equation}\:{below} \\ $$$${ax}^{\mathrm{2}} \:+{bx}\:+\:{c}\:=\:\mathrm{0}. \\ $$

Question Number 119366 Answers: 3 Comments: 0

lim_(x→−1) (((√(1+(√(x+5))))−(√3))/(x+1)) =?

$$\:\underset{{x}\rightarrow−\mathrm{1}} {\mathrm{lim}}\:\frac{\sqrt{\mathrm{1}+\sqrt{{x}+\mathrm{5}}}−\sqrt{\mathrm{3}}}{{x}+\mathrm{1}}\:=? \\ $$

Question Number 119373 Answers: 4 Comments: 1

Find all sum of all x interval [ 0, 2π ] such that 3cot^2 x + 8 cot x + 3 = 0

$${Find}\:{all}\:{sum}\:{of}\:{all}\:{x}\:{interval} \\ $$$$\left[\:\mathrm{0},\:\mathrm{2}\pi\:\right]\:{such}\:{that}\:\mathrm{3cot}\:^{\mathrm{2}} {x}\:+\:\mathrm{8}\:\mathrm{cot}\:{x}\:+\:\mathrm{3}\:=\:\mathrm{0} \\ $$

Question Number 119372 Answers: 2 Comments: 0

Determine minimum value of ((sec^4 α)/(tan^2 β)) + ((sec^4 β)/(tan^2 α)) , over all α,β ≠ ((kπ)/2) and k∈Z

$${Determine}\:{minimum}\:{value}\:{of}\: \\ $$$$\:\frac{\mathrm{sec}\:^{\mathrm{4}} \alpha}{\mathrm{tan}\:^{\mathrm{2}} \beta}\:+\:\frac{\mathrm{sec}\:^{\mathrm{4}} \beta}{\mathrm{tan}\:^{\mathrm{2}} \alpha}\:,\:{over}\:{all}\:\alpha,\beta\:\neq\:\frac{{k}\pi}{\mathrm{2}} \\ $$$${and}\:{k}\in\mathbb{Z} \\ $$

Question Number 119356 Answers: 1 Comments: 2

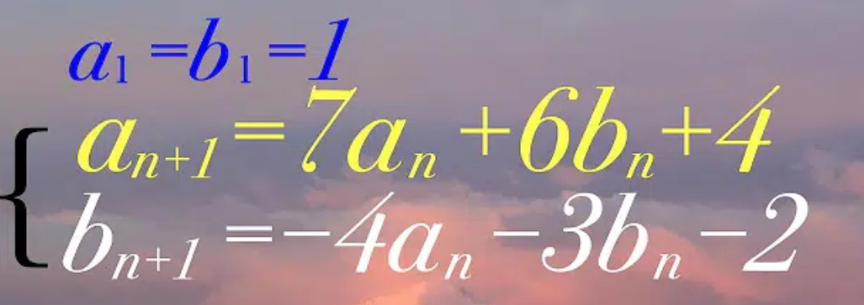
Question Number 119364 Answers: 2 Comments: 0

Suppose once more we′re asked to choose four students from high school class of 15 to form a committee but this time we have a restriction : we don′t want to committee to consist of all seniors or all juniors .suppose there are eight seniors and seven juniors in the class. How many different committe can we form?

$${Suppose}\:{once}\:{more}\:{we}'{re}\:{asked}\: \\ $$$${to}\:{choose}\:{four}\:{students}\:{from}\:{high}\:{school} \\ $$$${class}\:{of}\:\mathrm{15}\:{to}\:{form}\:{a}\:{committee} \\ $$$${but}\:{this}\:{time}\:{we}\:{have}\:{a}\:{restriction} \\ $$$$:\:{we}\:{don}'{t}\:{want}\:{to}\:{committee}\:{to} \\ $$$${consist}\:{of}\:{all}\:{seniors}\:{or}\:{all}\:{juniors} \\ $$$$.{suppose}\:{there}\:{are}\:{eight}\:{seniors} \\ $$$${and}\:{seven}\:{juniors}\:{in}\:{the}\:{class}.\:{How}\:{many} \\ $$$${different}\:{committe}\:{can}\:{we}\:{form}? \\ $$$$ \\ $$

Question Number 119335 Answers: 2 Comments: 0

Express f(x) = (1/((x−1)^2 (x^2 +1))) into partial fractions. hence evaluate I = ∫_0 ^4 f(x) dx

$$\:\mathrm{Express}\:{f}\left({x}\right)\:=\:\frac{\mathrm{1}}{\left({x}−\mathrm{1}\right)^{\mathrm{2}} \left({x}^{\mathrm{2}} +\mathrm{1}\right)}\:\:\mathrm{into}\:\mathrm{partial}\:\mathrm{fractions}. \\ $$$$\mathrm{hence}\:\mathrm{evaluate}\:{I}\:=\:\underset{\mathrm{0}} {\overset{\mathrm{4}} {\int}}\:{f}\left({x}\right)\:{dx} \\ $$

Question Number 119327 Answers: 2 Comments: 0

Question Number 119324 Answers: 0 Comments: 0

Question Number 119323 Answers: 1 Comments: 4

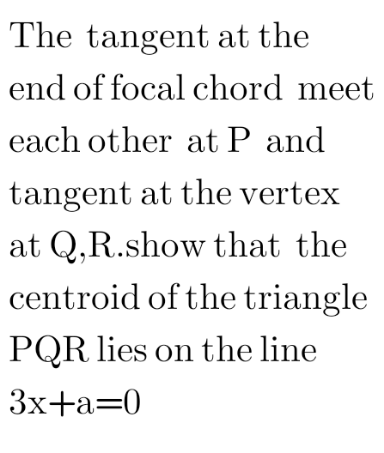
Question Number 119314 Answers: 1 Comments: 5

Question Number 119306 Answers: 3 Comments: 0

Question Number 119303 Answers: 3 Comments: 0

let x,y,z be positive real numbers such that x+y+z=1. Determine the minimum value of (1/x)+(4/y)+(9/z).

$$\:{let}\:{x},{y},{z}\:{be}\:{positive}\:{real}\:{numbers}\: \\ $$$${such}\:{that}\:{x}+{y}+{z}=\mathrm{1}.\:{Determine}\: \\ $$$${the}\:{minimum}\:{value}\:{of}\:\frac{\mathrm{1}}{{x}}+\frac{\mathrm{4}}{{y}}+\frac{\mathrm{9}}{{z}}. \\ $$

Question Number 119298 Answers: 1 Comments: 0

Question Number 119295 Answers: 1 Comments: 0

Let a and b non negative real numbers If sin x+acos x=b , express ∣ asin x−cos x∣ in terms of a and b.

$$\:{Let}\:{a}\:{and}\:{b}\:\:{non}\:{negative}\:{real}\:{numbers}\: \\ $$$${If}\:\mathrm{sin}\:{x}+{a}\mathrm{cos}\:{x}={b}\:,\:{express}\: \\ $$$$\mid\:{a}\mathrm{sin}\:{x}−\mathrm{cos}\:{x}\mid\:{in}\:{terms}\:{of}\:{a}\:{and}\:{b}. \\ $$$$ \\ $$

Question Number 119293 Answers: 1 Comments: 0

Question Number 119292 Answers: 2 Comments: 0

Question Number 119291 Answers: 2 Comments: 0

∫_0 ^( ∞) ((e^(−x) (x^(10) −1))/(ln(x))) dx

$$\int_{\mathrm{0}} ^{\:\infty} \frac{{e}^{−{x}} \left({x}^{\mathrm{10}} −\mathrm{1}\right)}{{ln}\left({x}\right)}\:{dx}\: \\ $$

Question Number 119290 Answers: 1 Comments: 0

(3x−y+1) dx +(6x+2y−3) dy = 0

$$\:\:\left(\mathrm{3}{x}−{y}+\mathrm{1}\right)\:{dx}\:+\left(\mathrm{6}{x}+\mathrm{2}{y}−\mathrm{3}\right)\:{dy}\:=\:\mathrm{0}\: \\ $$

Question Number 119282 Answers: 1 Comments: 0

... nice calculus... evaluate:: I:= ∫_0 ^( 1) li_2 (1−x^2 )dx=?? .m.n.1970.

$$\:\:\:\:\:\:\:\:...\:\:{nice}\:\:{calculus}... \\ $$$$\:\:\:\:\:\:\:\:\:\:\:{evaluate}:: \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\mathrm{I}:=\:\int_{\mathrm{0}} ^{\:\mathrm{1}} {li}_{\mathrm{2}} \left(\mathrm{1}−{x}^{\mathrm{2}} \right){dx}=?? \\ $$$$\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:\:.{m}.{n}.\mathrm{1970}. \\ $$$$\:\:\:\:\:\:\:\: \\ $$

Question Number 119280 Answers: 0 Comments: 0

Please more informations about this operator K_(p=1) ^∞ (......)

$$\:{Please}\:{more}\:{informations}\:{about}\:{this}\:{operator} \\ $$$$\:\:\underset{{p}=\mathrm{1}} {\overset{\infty} {{K}}}\left(......\right) \\ $$

Question Number 119318 Answers: 0 Comments: 0

find ∫_0 ^∞ ((lnx)/(x^4 +x^2 +2))dx

$${find}\:\int_{\mathrm{0}} ^{\infty} \:\:\:\frac{{lnx}}{{x}^{\mathrm{4}} +{x}^{\mathrm{2}} +\mathrm{2}}{dx} \\ $$

Question Number 119317 Answers: 0 Comments: 0

calculate ∫_0 ^(2π) (dθ/((x^2 −2cosθ x+1)^2 ))

$${calculate}\:\int_{\mathrm{0}} ^{\mathrm{2}\pi} \:\frac{{d}\theta}{\left({x}^{\mathrm{2}} −\mathrm{2}{cos}\theta\:{x}+\mathrm{1}\right)^{\mathrm{2}} } \\ $$

Question Number 119316 Answers: 0 Comments: 0

((Σ_(n=1) ^∞ (1/n^n ))/(Σ_(n=1) ^∞ (−1)^(n+1) (1/n^n )))

$$\frac{\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\frac{\mathrm{1}}{{n}^{{n}} }}{\underset{{n}=\mathrm{1}} {\overset{\infty} {\sum}}\left(−\mathrm{1}\right)^{{n}+\mathrm{1}} \frac{\mathrm{1}}{{n}^{{n}} }} \\ $$

Question Number 119315 Answers: 2 Comments: 0

Question Number 119266 Answers: 3 Comments: 2

solve (((√3)−1)/(sin x)) + (((√3)+1)/(cos x)) = 4(√2) where x∈ (0,(π/2))

$$\:{solve}\:\frac{\sqrt{\mathrm{3}}−\mathrm{1}}{\mathrm{sin}\:{x}}\:+\:\frac{\sqrt{\mathrm{3}}+\mathrm{1}}{\mathrm{cos}\:{x}}\:=\:\mathrm{4}\sqrt{\mathrm{2}} \\ $$$${where}\:{x}\in\:\left(\mathrm{0},\frac{\pi}{\mathrm{2}}\right) \\ $$

Pg 995 Pg 996 Pg 997 Pg 998 Pg 999 Pg 1000 Pg 1001 Pg 1002 Pg 1003 Pg 1004

Terms of Service

Contact: info@tinkutara.com