
AlgebraQuestion and Answers: Page 91
Question Number 184095 Answers: 2 Comments: 0

Question Number 184085 Answers: 1 Comments: 0
Question Number 184084 Answers: 1 Comments: 0

Question Number 184083 Answers: 1 Comments: 0
Question Number 184019 Answers: 1 Comments: 2
Question Number 183864 Answers: 4 Comments: 2

Question Number 183848 Answers: 2 Comments: 1

Question Number 183847 Answers: 5 Comments: 0
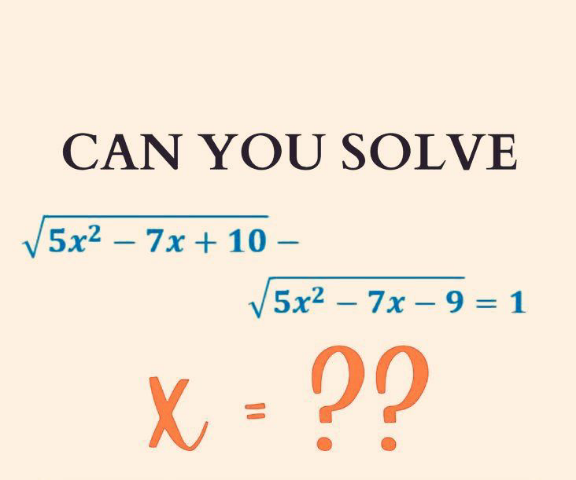
Question Number 183846 Answers: 4 Comments: 0
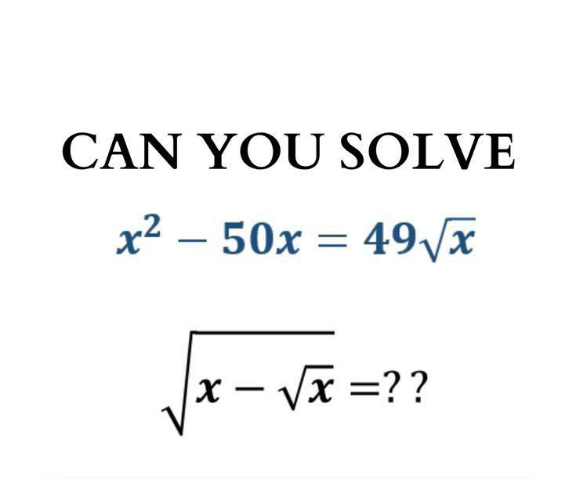
Question Number 183843 Answers: 1 Comments: 0

Question Number 183835 Answers: 2 Comments: 0
Question Number 183825 Answers: 1 Comments: 0
Question Number 183777 Answers: 2 Comments: 1

Question Number 183769 Answers: 0 Comments: 4
Question Number 183756 Answers: 2 Comments: 0
Question Number 183669 Answers: 4 Comments: 0
Question Number 183668 Answers: 1 Comments: 1
Question Number 183660 Answers: 3 Comments: 0
Question Number 183556 Answers: 1 Comments: 0

Question Number 183424 Answers: 0 Comments: 0
Question Number 183381 Answers: 1 Comments: 2
Question Number 183380 Answers: 0 Comments: 1
Question Number 183592 Answers: 1 Comments: 0
Question Number 183366 Answers: 0 Comments: 2
Question Number 183363 Answers: 1 Comments: 0
Question Number 183354 Answers: 0 Comments: 2
