Question and Answers Forum

AlgebraQuestion and Answers: Page 356

Question Number 20118 Answers: 1 Comments: 0

The quadratic equations x^2 − 6x + a = 0 and x^2 − cx + 6 = 0 have one root in common. The other roots of the first and second equations are integers in the ratio 4 : 3. Then, find the common root.

$$\mathrm{The}\:\mathrm{quadratic}\:\mathrm{equations}\:{x}^{\mathrm{2}} \:−\:\mathrm{6}{x}\:+\:{a}\:=\:\mathrm{0} \\ $$$$\mathrm{and}\:{x}^{\mathrm{2}} \:−\:{cx}\:+\:\mathrm{6}\:=\:\mathrm{0}\:\mathrm{have}\:\mathrm{one}\:\mathrm{root}\:\mathrm{in} \\ $$$$\mathrm{common}.\:\mathrm{The}\:\mathrm{other}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{first} \\ $$$$\mathrm{and}\:\mathrm{second}\:\mathrm{equations}\:\mathrm{are}\:\mathrm{integers}\:\mathrm{in} \\ $$$$\mathrm{the}\:\mathrm{ratio}\:\mathrm{4}\::\:\mathrm{3}.\:\mathrm{Then},\:\mathrm{find}\:\mathrm{the}\:\mathrm{common} \\ $$$$\mathrm{root}. \\ $$

Question Number 20116 Answers: 1 Comments: 0

If a and b (≠ 0) are the roots of the equation x^2 + ax + b = 0, then find the least value of x^2 + ax + b (x ∈ R).

$$\mathrm{If}\:{a}\:\mathrm{and}\:{b}\:\left(\neq\:\mathrm{0}\right)\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{equation}\:{x}^{\mathrm{2}} \:+\:{ax}\:+\:{b}\:=\:\mathrm{0},\:\mathrm{then}\:\mathrm{find}\:\mathrm{the} \\ $$$$\mathrm{least}\:\mathrm{value}\:\mathrm{of}\:{x}^{\mathrm{2}} \:+\:{ax}\:+\:{b}\:\left({x}\:\in\:{R}\right). \\ $$

Question Number 20115 Answers: 1 Comments: 0

The value of a for which the equation (1 − a^2 )x^2 + 2ax − 1 = 0 has roots belonging to (0, 1) is

$$\mathrm{The}\:\mathrm{value}\:\mathrm{of}\:{a}\:\mathrm{for}\:\mathrm{which}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\left(\mathrm{1}\:−\:{a}^{\mathrm{2}} \right){x}^{\mathrm{2}} \:+\:\mathrm{2}{ax}\:−\:\mathrm{1}\:=\:\mathrm{0}\:\mathrm{has}\:\mathrm{roots} \\ $$$$\mathrm{belonging}\:\mathrm{to}\:\left(\mathrm{0},\:\mathrm{1}\right)\:\mathrm{is} \\ $$

Question Number 20054 Answers: 1 Comments: 0

If α and β (α < β) are the roots of the equation x^2 + bx + c = 0, where c < 0 < b, then (1) 0 < α < β (2) α < 0 < β < ∣α∣ (3) α < β < 0 (4) α < 0 < ∣α∣ < β

$$\mathrm{If}\:\alpha\:\mathrm{and}\:\beta\:\left(\alpha\:<\:\beta\right)\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the} \\ $$$$\mathrm{equation}\:{x}^{\mathrm{2}} \:+\:{bx}\:+\:{c}\:=\:\mathrm{0},\:\mathrm{where} \\ $$$${c}\:<\:\mathrm{0}\:<\:{b},\:\mathrm{then} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{0}\:<\:\alpha\:<\:\beta \\ $$$$\left(\mathrm{2}\right)\:\alpha\:<\:\mathrm{0}\:<\:\beta\:<\:\mid\alpha\mid \\ $$$$\left(\mathrm{3}\right)\:\alpha\:<\:\beta\:<\:\mathrm{0} \\ $$$$\left(\mathrm{4}\right)\:\alpha\:<\:\mathrm{0}\:<\:\mid\alpha\mid\:<\:\beta \\ $$

Question Number 20053 Answers: 1 Comments: 0

If (4a + c)^2 ≤ 4b^2 then one root of ax^2 + bx + c = 0 lies in (1) (−2, 2) (2) (−1, 1) (3) (−∞, −2) (4) (2, ∞)

$$\mathrm{If}\:\left(\mathrm{4}{a}\:+\:{c}\right)^{\mathrm{2}} \:\leqslant\:\mathrm{4}{b}^{\mathrm{2}} \:\mathrm{then}\:\mathrm{one}\:\mathrm{root}\:\mathrm{of} \\ $$$${ax}^{\mathrm{2}} \:+\:{bx}\:+\:{c}\:=\:\mathrm{0}\:\mathrm{lies}\:\mathrm{in} \\ $$$$\left(\mathrm{1}\right)\:\left(−\mathrm{2},\:\mathrm{2}\right) \\ $$$$\left(\mathrm{2}\right)\:\left(−\mathrm{1},\:\mathrm{1}\right) \\ $$$$\left(\mathrm{3}\right)\:\left(−\infty,\:−\mathrm{2}\right) \\ $$$$\left(\mathrm{4}\right)\:\left(\mathrm{2},\:\infty\right) \\ $$

Question Number 20052 Answers: 1 Comments: 0

If the roots α and β of the equation ax^2 + bx + c = 0 are real and of opposite sign then the roots of the equation α(x − β)^2 + β(x − α)^2 is/are (1) Positive (2) Negative (3) Real and opposite sign (4) Imaginary

$$\mathrm{If}\:\mathrm{the}\:\mathrm{roots}\:\alpha\:\mathrm{and}\:\beta\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$${ax}^{\mathrm{2}} \:+\:{bx}\:+\:{c}\:=\:\mathrm{0}\:\mathrm{are}\:\mathrm{real}\:\mathrm{and}\:\mathrm{of}\:\mathrm{opposite} \\ $$$$\mathrm{sign}\:\mathrm{then}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{equation} \\ $$$$\alpha\left({x}\:−\:\beta\right)^{\mathrm{2}} \:+\:\beta\left({x}\:−\:\alpha\right)^{\mathrm{2}} \:\mathrm{is}/\mathrm{are} \\ $$$$\left(\mathrm{1}\right)\:\mathrm{Positive} \\ $$$$\left(\mathrm{2}\right)\:\mathrm{Negative} \\ $$$$\left(\mathrm{3}\right)\:\mathrm{Real}\:\mathrm{and}\:\mathrm{opposite}\:\mathrm{sign} \\ $$$$\left(\mathrm{4}\right)\:\mathrm{Imaginary} \\ $$

Question Number 20047 Answers: 1 Comments: 0

Solve for x: ((√(x + 1))/x) + (√(x/(x + 1))) = ((13)/6)

$$\mathrm{Solve}\:\mathrm{for}\:\mathrm{x}: \\ $$$$\frac{\sqrt{\mathrm{x}\:+\:\mathrm{1}}}{\mathrm{x}}\:+\:\sqrt{\frac{\mathrm{x}}{\mathrm{x}\:+\:\mathrm{1}}}\:=\:\frac{\mathrm{13}}{\mathrm{6}} \\ $$

Question Number 20051 Answers: 1 Comments: 0

If x ∈ R then ((x^2 + 2x + a)/(x^2 + 4x + 3a)) can take all real values if (1) a ∈ (0, 2) (2) a ∈ [0, 1] (3) a ∈ [−1, 1] (4) None of these

$$\mathrm{If}\:{x}\:\in\:{R}\:\mathrm{then}\:\frac{{x}^{\mathrm{2}} \:+\:\mathrm{2}{x}\:+\:{a}}{{x}^{\mathrm{2}} \:+\:\mathrm{4}{x}\:+\:\mathrm{3}{a}}\:\mathrm{can}\:\mathrm{take}\:\mathrm{all} \\ $$$$\mathrm{real}\:\mathrm{values}\:\mathrm{if} \\ $$$$\left(\mathrm{1}\right)\:{a}\:\in\:\left(\mathrm{0},\:\mathrm{2}\right) \\ $$$$\left(\mathrm{2}\right)\:{a}\:\in\:\left[\mathrm{0},\:\mathrm{1}\right] \\ $$$$\left(\mathrm{3}\right)\:{a}\:\in\:\left[−\mathrm{1},\:\mathrm{1}\right] \\ $$$$\left(\mathrm{4}\right)\:\mathrm{None}\:\mathrm{of}\:\mathrm{these} \\ $$

Question Number 20013 Answers: 0 Comments: 4

Show that the equation (1/(x − a)) + (1/(x − b)) + (1/(x − c)) = 0 can have a pair of equal roots if a = b = c.

$$\mathrm{Show}\:\mathrm{that}\:\mathrm{the}\:\mathrm{equation}\:\frac{\mathrm{1}}{{x}\:−\:{a}}\:+\:\frac{\mathrm{1}}{{x}\:−\:{b}} \\ $$$$+\:\frac{\mathrm{1}}{{x}\:−\:{c}}\:=\:\mathrm{0}\:\mathrm{can}\:\mathrm{have}\:\mathrm{a}\:\mathrm{pair}\:\mathrm{of}\:\mathrm{equal} \\ $$$$\mathrm{roots}\:\mathrm{if}\:{a}\:=\:{b}\:=\:{c}. \\ $$

Question Number 20001 Answers: 1 Comments: 0

The number of the roots of the quadratic equation 8sec^2 θ − 6secθ + 1 = 0 is

$$\mathrm{The}\:\mathrm{number}\:\mathrm{of}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{the}\:\mathrm{quadratic} \\ $$$$\mathrm{equation}\:\mathrm{8sec}^{\mathrm{2}} \theta\:−\:\mathrm{6sec}\theta\:+\:\mathrm{1}\:=\:\mathrm{0}\:\mathrm{is} \\ $$

Question Number 19945 Answers: 1 Comments: 0

If α and β are the roots of equation x^2 + px + q = 0 and α^2 , β^2 are roots of the equation x^2 − rx + s = 0, show that the equation x^2 − 4qx + 2q^2 − r = 0 has real roots.

$$\mathrm{If}\:\alpha\:\mathrm{and}\:\beta\:\mathrm{are}\:\mathrm{the}\:\mathrm{roots}\:\mathrm{of}\:\mathrm{equation} \\ $$$${x}^{\mathrm{2}} \:+\:{px}\:+\:{q}\:=\:\mathrm{0}\:\mathrm{and}\:\alpha^{\mathrm{2}} ,\:\beta^{\mathrm{2}} \:\mathrm{are}\:\mathrm{roots}\:\mathrm{of} \\ $$$$\mathrm{the}\:\mathrm{equation}\:{x}^{\mathrm{2}} \:−\:{rx}\:+\:{s}\:=\:\mathrm{0},\:\mathrm{show} \\ $$$$\mathrm{that}\:\mathrm{the}\:\mathrm{equation}\:{x}^{\mathrm{2}} \:−\:\mathrm{4}{qx}\:+\:\mathrm{2}{q}^{\mathrm{2}} \:−\:{r}\:=\:\mathrm{0} \\ $$$$\mathrm{has}\:\mathrm{real}\:\mathrm{roots}. \\ $$

Question Number 19936 Answers: 1 Comments: 1

Find the pricipal value of (1−i)^(1+i) .

$${Find}\:{the}\:{pricipal}\:{value}\:{of}\: \\ $$$$\:\:\:\:\:\left(\mathrm{1}−{i}\right)^{\mathrm{1}+{i}} \:. \\ $$

Question Number 19914 Answers: 0 Comments: 0

Question Number 19900 Answers: 2 Comments: 0

Prove that this is an identity in x: (((x−a)(x−b))/((c−a)(c−b)))+(((x−b)(x−c))/((a−b)(a−c)))+(((x−c)(x−a))/((b−c)(b−a)))=1

$$\mathrm{Prove}\:\mathrm{that}\:\mathrm{this}\:\mathrm{is}\:\mathrm{an}\:\mathrm{identity}\:\mathrm{in}\:{x}: \\ $$$$\frac{\left({x}−{a}\right)\left({x}−{b}\right)}{\left({c}−{a}\right)\left({c}−{b}\right)}+\frac{\left({x}−{b}\right)\left({x}−{c}\right)}{\left({a}−{b}\right)\left({a}−{c}\right)}+\frac{\left({x}−{c}\right)\left({x}−{a}\right)}{\left({b}−{c}\right)\left({b}−{a}\right)}=\mathrm{1} \\ $$

Question Number 19898 Answers: 2 Comments: 0

Simplify : i log (((x − i)/(x + i))).

$$\mathrm{Simplify}\::\:{i}\:\mathrm{log}\:\left(\frac{{x}\:−\:{i}}{{x}\:+\:{i}}\right). \\ $$

Question Number 19875 Answers: 1 Comments: 1

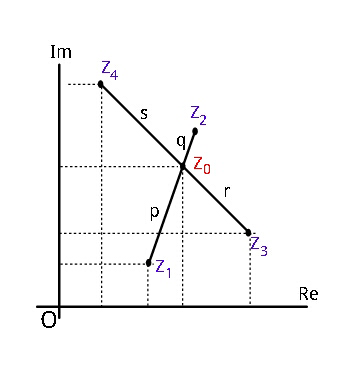
Question Number 19799 Answers: 0 Comments: 2

For a natural number b, let N(b) denote the number of natural numbers a for which the equation x^2 + ax + b = 0 has integer roots. What is the smallest value of b for which N(b) = 20?

$$\mathrm{For}\:\mathrm{a}\:\mathrm{natural}\:\mathrm{number}\:{b},\:\mathrm{let}\:{N}\left({b}\right)\:\mathrm{denote} \\ $$$$\mathrm{the}\:\mathrm{number}\:\mathrm{of}\:\mathrm{natural}\:\mathrm{numbers}\:{a}\:\mathrm{for} \\ $$$$\mathrm{which}\:\mathrm{the}\:\mathrm{equation}\:{x}^{\mathrm{2}} \:+\:{ax}\:+\:{b}\:=\:\mathrm{0}\:\mathrm{has} \\ $$$$\mathrm{integer}\:\mathrm{roots}.\:\mathrm{What}\:\mathrm{is}\:\mathrm{the}\:\mathrm{smallest} \\ $$$$\mathrm{value}\:\mathrm{of}\:{b}\:\mathrm{for}\:\mathrm{which}\:{N}\left({b}\right)\:=\:\mathrm{20}? \\ $$

Question Number 19741 Answers: 0 Comments: 1

If z = x + iy and arg(((z − 2)/(z + 2))) = (π/6), then find the locus of z.

$$\mathrm{If}\:{z}\:=\:{x}\:+\:{iy}\:\mathrm{and}\:\mathrm{arg}\left(\frac{{z}\:−\:\mathrm{2}}{{z}\:+\:\mathrm{2}}\right)\:=\:\frac{\pi}{\mathrm{6}},\:\mathrm{then} \\ $$$$\mathrm{find}\:\mathrm{the}\:\mathrm{locus}\:\mathrm{of}\:{z}. \\ $$

Question Number 19740 Answers: 1 Comments: 0

If ∣z^2 − 1∣ = ∣z∣^2 + 1, then z lies on

$$\mathrm{If}\:\mid{z}^{\mathrm{2}} \:−\:\mathrm{1}\mid\:=\:\mid{z}\mid^{\mathrm{2}} \:+\:\mathrm{1},\:\mathrm{then}\:{z}\:\mathrm{lies}\:\mathrm{on} \\ $$

Question Number 19739 Answers: 1 Comments: 0

If z = x + iy is a complex number satisfying ∣z + (i/2)∣^2 = ∣z − (i/2)∣^2 , then the locus of z is

$$\mathrm{If}\:{z}\:=\:{x}\:+\:{iy}\:\mathrm{is}\:\mathrm{a}\:\mathrm{complex}\:\mathrm{number} \\ $$$$\mathrm{satisfying}\:\mid{z}\:+\:\frac{{i}}{\mathrm{2}}\mid^{\mathrm{2}} \:=\:\mid{z}\:−\:\frac{{i}}{\mathrm{2}}\mid^{\mathrm{2}} ,\:\mathrm{then} \\ $$$$\mathrm{the}\:\mathrm{locus}\:\mathrm{of}\:{z}\:\mathrm{is} \\ $$

Question Number 19738 Answers: 1 Comments: 1

Locus of the point z satisfying the equation ∣iz − 1∣ + ∣z − i∣ = 2 is