
AlgebraQuestion and Answers: Page 327
Question Number 43757 Answers: 0 Comments: 0
Question Number 43756 Answers: 1 Comments: 0
Question Number 43753 Answers: 0 Comments: 0

Question Number 43716 Answers: 1 Comments: 3
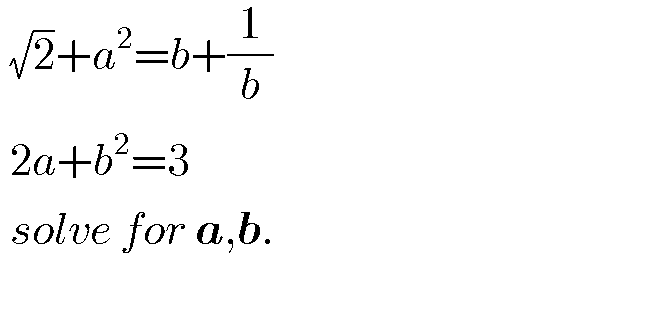
Question Number 43707 Answers: 1 Comments: 3
Question Number 43706 Answers: 1 Comments: 2
Question Number 43705 Answers: 0 Comments: 0
Question Number 43702 Answers: 1 Comments: 0
Question Number 43665 Answers: 1 Comments: 1
Question Number 43608 Answers: 0 Comments: 3
Question Number 43574 Answers: 1 Comments: 0
Question Number 43569 Answers: 0 Comments: 1
Question Number 43549 Answers: 1 Comments: 1
Question Number 43544 Answers: 1 Comments: 0
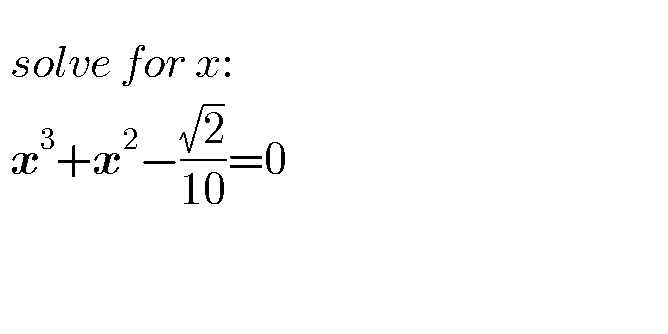
Question Number 43540 Answers: 2 Comments: 0
Question Number 43514 Answers: 0 Comments: 0
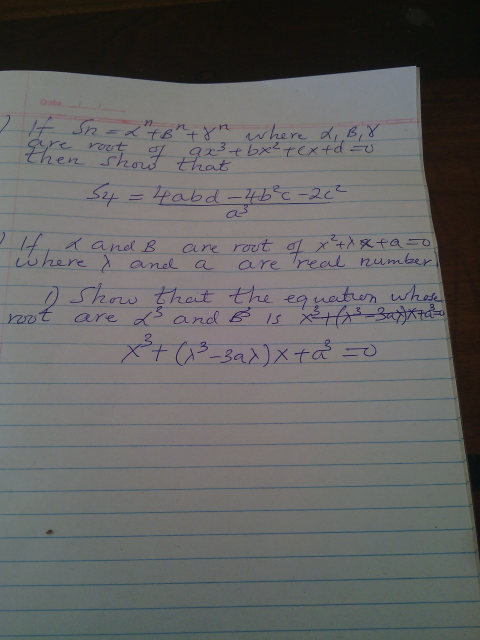
Question Number 43587 Answers: 1 Comments: 0
Question Number 43496 Answers: 1 Comments: 4
Question Number 43488 Answers: 1 Comments: 0
Question Number 43486 Answers: 3 Comments: 1

Question Number 43471 Answers: 1 Comments: 0

Question Number 43449 Answers: 1 Comments: 0
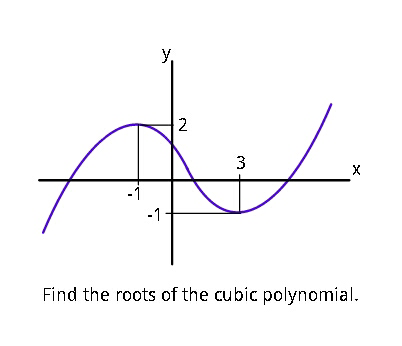
Question Number 43416 Answers: 0 Comments: 1

Question Number 43393 Answers: 0 Comments: 0

Question Number 43391 Answers: 1 Comments: 0
Question Number 43363 Answers: 3 Comments: 1
Pg 322 Pg 323 Pg 324 Pg 325 Pg 326 Pg 327 Pg 328 Pg 329 Pg 330 Pg 331
