
AlgebraQuestion and Answers: Page 312
Question Number 58246 Answers: 1 Comments: 0
Question Number 58210 Answers: 0 Comments: 5
Question Number 58156 Answers: 1 Comments: 0
Question Number 58154 Answers: 1 Comments: 0
Question Number 58153 Answers: 1 Comments: 0
Question Number 58145 Answers: 1 Comments: 0
Question Number 58135 Answers: 1 Comments: 0
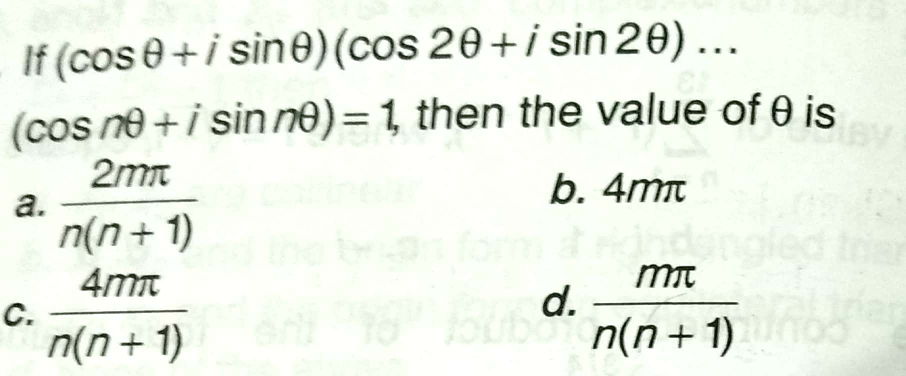
Question Number 58092 Answers: 3 Comments: 0
$$\mathrm{6}{x}^{\mathrm{3}} +\mathrm{5}{x}^{\mathrm{2}} −\mathrm{6}{x}−\mathrm{5}=\mathrm{0} \\ $$
Question Number 58084 Answers: 0 Comments: 3

Question Number 58077 Answers: 1 Comments: 0
Question Number 58046 Answers: 2 Comments: 0
Question Number 57988 Answers: 0 Comments: 5
Question Number 57984 Answers: 0 Comments: 0

Question Number 58045 Answers: 1 Comments: 0
Question Number 57977 Answers: 0 Comments: 0
Question Number 57960 Answers: 1 Comments: 0
$${Find}\:{maximum}\:{n}\:{such}\:{that}\:\mathrm{12}^{{n}} \:{divides} \\ $$$$\mathrm{100}!. \\ $$
Question Number 57955 Answers: 1 Comments: 1

Question Number 57947 Answers: 0 Comments: 0
Question Number 57946 Answers: 0 Comments: 0

Question Number 57932 Answers: 1 Comments: 0
Question Number 57930 Answers: 1 Comments: 0
$$\mathrm{solve}\:\mathrm{2}.\mathrm{3}\left(\frac{\mathrm{2}}{\mathrm{11}}+\mathrm{3}\right) \\ $$
Question Number 57902 Answers: 1 Comments: 1
Question Number 57881 Answers: 0 Comments: 0
$$\mathrm{calculate}\frac{\mathrm{2}}{\mathrm{13}}×\mathrm{2}\frac{\mathrm{1}}{\mathrm{4}} \\ $$
Question Number 57880 Answers: 0 Comments: 0
Question Number 57818 Answers: 1 Comments: 0
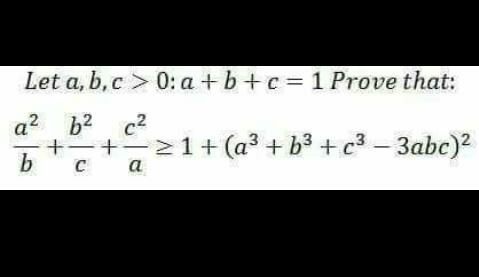
Question Number 57791 Answers: 3 Comments: 0
Pg 307 Pg 308 Pg 309 Pg 310 Pg 311 Pg 312 Pg 313 Pg 314 Pg 315 Pg 316
