
AlgebraQuestion and Answers: Page 307
Question Number 62133 Answers: 0 Comments: 0
$$\mathrm{5}\left(\mathrm{2}+\mathrm{3}+\mathrm{1}\right) \\ $$
Question Number 62132 Answers: 0 Comments: 0
Question Number 62131 Answers: 0 Comments: 0
$$\frac{\mathrm{1}}{\mathrm{3}}+\mathrm{3}\frac{\mathrm{1}}{\mathrm{4}} \\ $$
Question Number 62112 Answers: 2 Comments: 0

Question Number 62092 Answers: 0 Comments: 1
Question Number 62057 Answers: 1 Comments: 2
Question Number 62055 Answers: 0 Comments: 0
Question Number 62054 Answers: 0 Comments: 1
Question Number 62053 Answers: 0 Comments: 1
Question Number 62052 Answers: 0 Comments: 1
$$\mathrm{3}\frac{\mathrm{1}}{\mathrm{4}}−\frac{\mathrm{3}}{\mathrm{4}} \\ $$
Question Number 62051 Answers: 0 Comments: 1
$$\frac{\mathrm{7}^{\mathrm{5}} }{\mathrm{7}^{\mathrm{3}} } \\ $$
Question Number 62050 Answers: 0 Comments: 1
Question Number 62049 Answers: 0 Comments: 1
$$\frac{\mathrm{1}}{\mathrm{4}}+\frac{\mathrm{1}}{\mathrm{5}} \\ $$
Question Number 62048 Answers: 0 Comments: 1
$$\left(\frac{\mathrm{1}}{\mathrm{2}}\right)^{\mathrm{3}} \\ $$
Question Number 62047 Answers: 0 Comments: 1
Question Number 62045 Answers: 1 Comments: 0
Question Number 62041 Answers: 0 Comments: 2
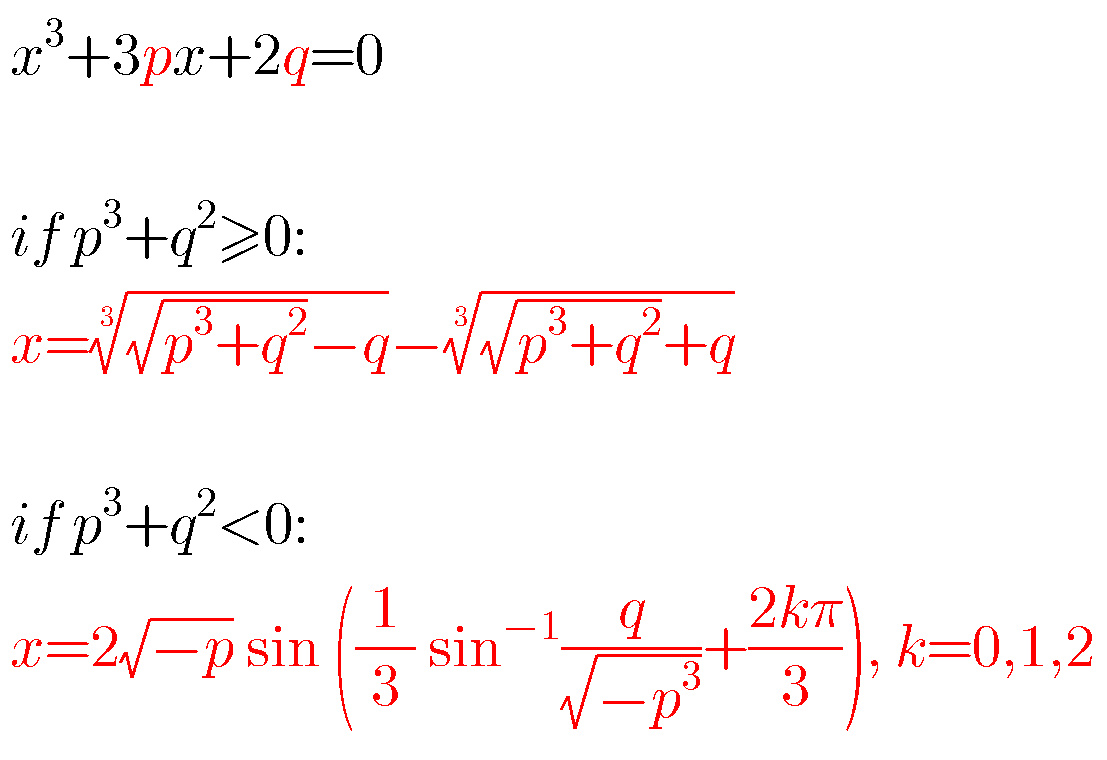
Question Number 62023 Answers: 3 Comments: 3
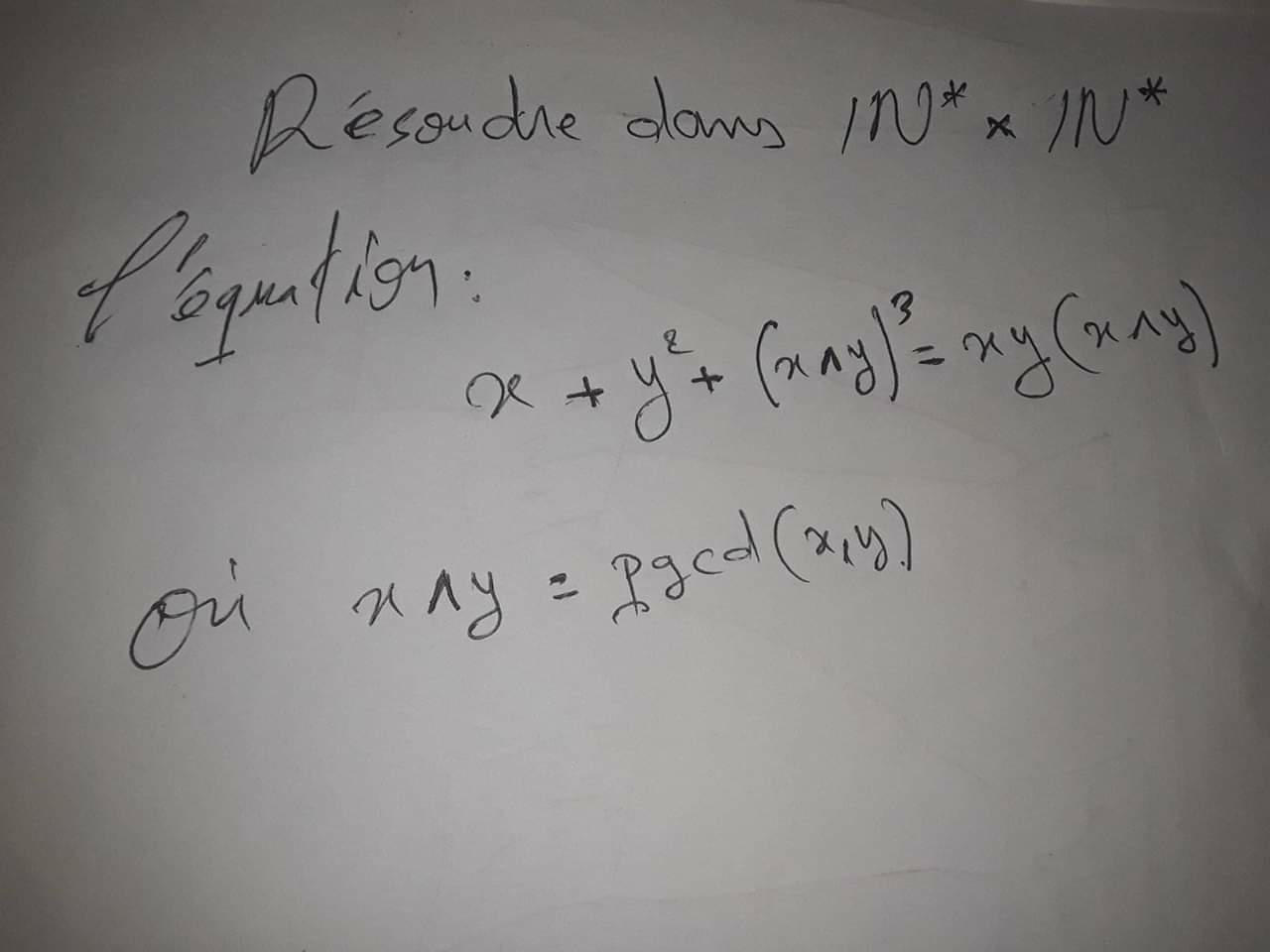
Question Number 62046 Answers: 0 Comments: 1
$$\mathrm{4}×\left(\mathrm{3}+\mathrm{2}−\mathrm{3}\right) \\ $$
Question Number 61937 Answers: 1 Comments: 5
Question Number 61895 Answers: 0 Comments: 3
Question Number 61892 Answers: 0 Comments: 1
$$\frac{\mathrm{1}}{\mathrm{4}}\:\mathrm{of}\:\frac{\mathrm{2}}{\mathrm{5}} \\ $$
Question Number 61873 Answers: 1 Comments: 2
Question Number 61850 Answers: 1 Comments: 8
Question Number 61811 Answers: 0 Comments: 0

Question Number 61708 Answers: 0 Comments: 1
$$\mathrm{8}+\left(\mathrm{4}+\mathrm{3}×\mathrm{2}\right) \\ $$
Pg 302 Pg 303 Pg 304 Pg 305 Pg 306 Pg 307 Pg 308 Pg 309 Pg 310 Pg 311
