
AlgebraQuestion and Answers: Page 302
Question Number 65087 Answers: 1 Comments: 0

Question Number 65115 Answers: 1 Comments: 0
$$\mathrm{x}^{\mathrm{x}} =\mathrm{64} \\ $$$$\mathrm{find}\:\mathrm{x} \\ $$
Question Number 65114 Answers: 0 Comments: 0
$$\mathrm{x}^{\mathrm{x}^{\mathrm{lnx}} } =\mathrm{64} \\ $$$$\mathrm{find}\:\mathrm{x} \\ $$
Question Number 65062 Answers: 1 Comments: 5
Question Number 65054 Answers: 2 Comments: 2
Question Number 64867 Answers: 1 Comments: 0
Question Number 64837 Answers: 1 Comments: 0
Question Number 64758 Answers: 2 Comments: 0
Question Number 64631 Answers: 1 Comments: 3
Question Number 64604 Answers: 0 Comments: 0
Question Number 64580 Answers: 3 Comments: 0
Question Number 64542 Answers: 2 Comments: 0

Question Number 64519 Answers: 1 Comments: 0
Question Number 64508 Answers: 2 Comments: 1
Question Number 64475 Answers: 2 Comments: 6
Question Number 64447 Answers: 1 Comments: 0
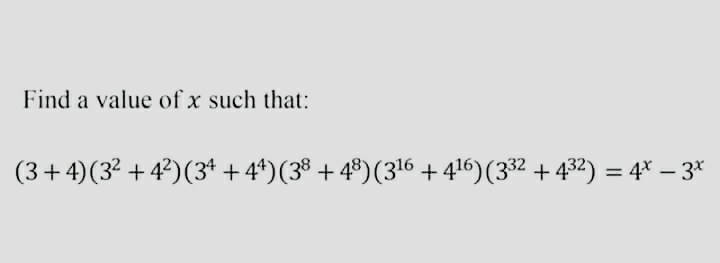
Question Number 64404 Answers: 0 Comments: 4
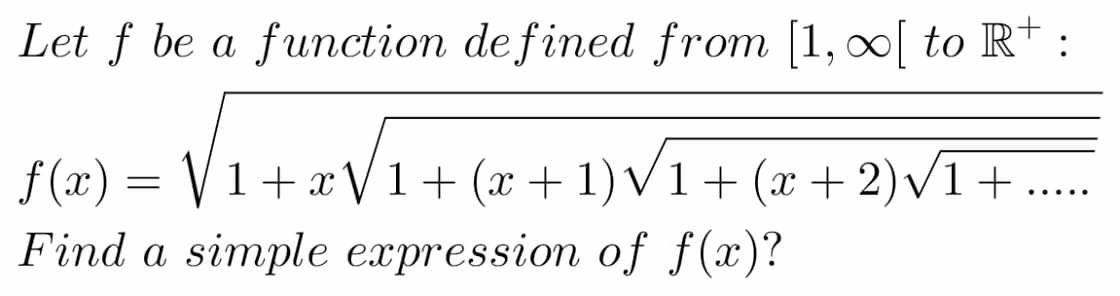
Question Number 64378 Answers: 0 Comments: 1

Question Number 64284 Answers: 0 Comments: 0
Question Number 64187 Answers: 1 Comments: 0

Question Number 64186 Answers: 1 Comments: 0

Question Number 64185 Answers: 2 Comments: 3

Question Number 64174 Answers: 0 Comments: 0
Question Number 64130 Answers: 2 Comments: 1
Question Number 64126 Answers: 1 Comments: 0

Question Number 64111 Answers: 0 Comments: 0
Pg 297 Pg 298 Pg 299 Pg 300 Pg 301 Pg 302 Pg 303 Pg 304 Pg 305 Pg 306
