
AlgebraQuestion and Answers: Page 300
Question Number 68935 Answers: 2 Comments: 0
Question Number 68912 Answers: 1 Comments: 0

Question Number 68899 Answers: 0 Comments: 9
Question Number 68898 Answers: 0 Comments: 7
Question Number 68835 Answers: 1 Comments: 4
Question Number 68768 Answers: 1 Comments: 1
Question Number 68740 Answers: 1 Comments: 1
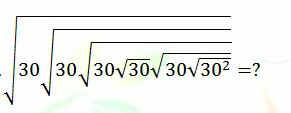
Question Number 68721 Answers: 1 Comments: 1

Question Number 68636 Answers: 2 Comments: 0
Question Number 68624 Answers: 2 Comments: 0

Question Number 68549 Answers: 1 Comments: 3
$${y}=\frac{{x}^{\mathrm{3}} }{{x}^{\mathrm{2}} +\mathrm{1}} \\ $$$${y}^{−\mathrm{1}} =... \\ $$
Question Number 68433 Answers: 0 Comments: 0
Question Number 68422 Answers: 1 Comments: 0

Question Number 68414 Answers: 2 Comments: 1
Question Number 68336 Answers: 1 Comments: 1
Question Number 68285 Answers: 1 Comments: 2
Question Number 68280 Answers: 1 Comments: 1
Question Number 68234 Answers: 1 Comments: 5

Question Number 68207 Answers: 1 Comments: 1
Question Number 68161 Answers: 0 Comments: 7
Question Number 68122 Answers: 1 Comments: 1

Question Number 67997 Answers: 0 Comments: 0
Question Number 67992 Answers: 1 Comments: 0
Question Number 67881 Answers: 1 Comments: 0
Question Number 67826 Answers: 2 Comments: 4

Question Number 67819 Answers: 0 Comments: 1
Pg 295 Pg 296 Pg 297 Pg 298 Pg 299 Pg 300 Pg 301 Pg 302 Pg 303 Pg 304
